Akademik Bilişim '10 10 - 12 Şubat 2010 Muğla

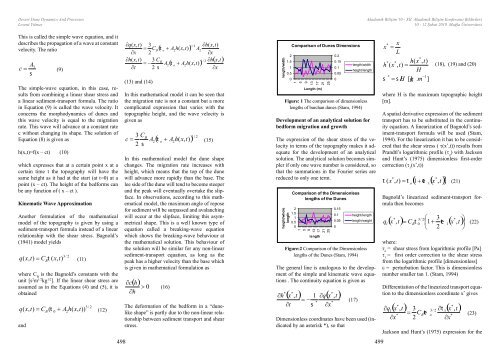
Desert Dune Dynamics And ProcessesLevent YılmazThis is called the simple wave equation, and itdescribes the propagation of a wave at constantvelocity. The ratioAc = 3(9)sThe simple-wave equation, in this case, resultsfrom combining a linear shear stress anda linear sediment-transport formula. The ratioin Equation (9) is called the wave velocity. Itconcerns the morphodynamics of dunes andthis wave velocity is equal to the migrationrate. This wave will advance at a constant ratec without changing its shape. The solution ofEquation (8) is given ash(x,t)=f(x – ct) (10)which expresses that at a certain point x at acertain time t the topography will have thesame height as it had at the start (at t=0) at apoint (x – ct). The height of the bedforms canbe any function of ( x – ct ).Kinematic Wave ApproximationAnother formulation of the mathematicalmodel of the topography is given by using asediment-transport formula instead of a linearrelationship with the shear stress. Bagnold’s(1941) model yieldsq( x,t)t= 3 / 2C t B( x,) (11)where C Bis the Bagnold’s constants with theunit [s 2 m 1/2 kg 1/2 ]. If the linear shear stress areassumed as in the Equations (4) and (5), it isobtainedq( x,t)B+ tand3 / 2= C ( t0A2h(x,)) (12)498∂q(x,t)3= CB∂x2∂h(x,t)3 C= −∂t2 s(13) and (14)( t + A h(x,t))BoA22( t + A h(x,t))o21/ 2∂h(x,t)A2∂x1/ 2 ∂h∂x( x,t)In this mathematical model it can be seen thatthe migration rate is not a constant but a morecomplicated expression that varies with thetopographic height, and the wave velocity isgiven as3 Cc = t2 s( A h(x,t ) 1/ 2BA2 o+2)(15)In this mathematical model the dune shapechanges. The migration rate increases withheight, which means that the top of the dunewill advance more rapidly than the base. Thelee side of the dune will tend to become steeperand the peak will eventually overtake the slipface.In observations, according to this mathematicalmodel, the maximum angle of reposefor sediment will be surpassed and avalanchingwill occur at the slipface, limiting this asymmetricalshape. This is a well known type ofequation called a breaking-wave equationwhich shows the breaking-wave behaviour atthe mathematical solution. This behaviour ofthe solution will be similar for any non-linearsediment-transport equation, as long as thepeak has a higher velocity than the base whichis given in mathematical formulation as( h)∂c∂h> 0(16)The deformation of the bedform in a “dunelikeshape” is partly due to the non-linear relationshipbetween sediment transport and shearstress.length/width21.510.50Comparison of Dunes Dimensions15913172125Length (m)0.20.150.10.050length/widthheight/lengthFigure: 1 The comparison of dimensionlesslengths of barchan dunes (Stam, 1994)Development of an analytical solution forbedform migration and growthThe expression of the shear stress of the velocityin terms of the topography makes it adequatefor the development of an analyticalsolution. The analytical solution becomes simplerif only one wave number is considered, sothat the summations in the Fourier series arereduced to only one term.height/wholelengthComparison of the Dimensionlesslengths of the Dunes21.510.5091317212515length0.150.10.050height/lengthlength/weightFigure:2 Comparison of the Dimensionlesslengths of the Dunes (Stam, 1994)The general line is analogous to the developmentof the simple and kinematic wave equations. The continuity equation is given as*∂h∂t**( x , t) 1 ∂q( x , t)= −s*∂x*(17)Dimensionless coordinates have been used (indicatedby an asterisk *), so thatAkademik Bilişim’10 - XII. Akademik Bilişim Konferansı Bildirileri10 - 12 Şubat 2010 Muğla Üniversitesi499* xx =L** * h(x , t)h ( x , t)=(18), (19) and (20)H*−1s = s H [ . mk g ] Lwhere H is the maximum topographic height[m].A spatial derivative expression of the sedimenttransport has to be substituted in the continuityequation. A linearization of Bagnold’s sediment-transportformula will be used (Stam,1994). For the linearization it has to be consideredthat the shear stress ( τ(x * ,t)) results fromPrandtl’s logarithmic profile (τ o) with Jacksonand Hunt’s (1975) dimensionless first-ordercorrection (τ 1(x * ,t))*( 1 ( x t)*t ( x , t)= to+ e t , (21)1Bagnold’s linearized sediment-transport formulathen becomesq⎛ ⎞( x*3, t ) = C3 / 2⎜1+ ( x*et t )⎟ ⎠t01(22)⎝ 2l B,where:τ o= shear stress from logarithmic profile [Pa]τ 1= first order correction to the shear stressfrom the logarithmic profile [dimensionless]ε = perturbation factor. This is dimensionlessnumber smaller tan 1. (Stam, 1994)Differentiation of the linearized transport equationto the dimensionless coordinate x * gives∂ql∂x**( x , t) 3 3 / 2 ∂t( x , t)*= C21Be ot *(23)∂xJackson and Hunt’s (1975) expression for the