Practical_Antenna_Handbook_0071639586

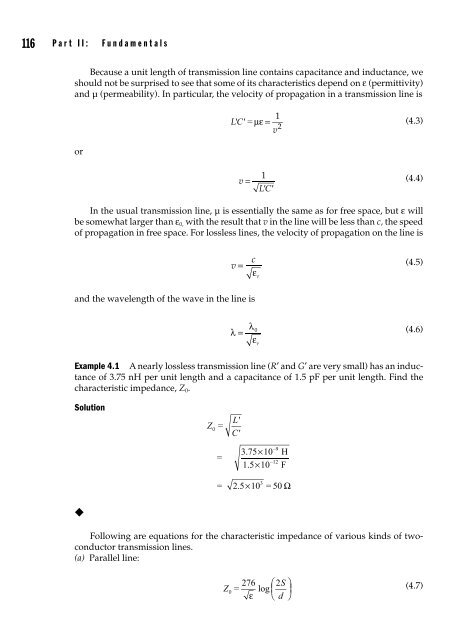
116 P a r t I I : F u n d a m e n t a l s
Because a unit length of transmission line contains capacitance and inductance, we
should not be surprised to see that some of its characteristics depend on ε (permittivity)
and µ (permeability). In particular, the velocity of propagation in a transmission line is
or
1
L'C' = µε =
(4.3)
2
v
v =
1
L'C'
(4.4)
In the usual transmission line, µ is essentially the same as for free space, but ε will
be somewhat larger than ε 0, with the result that v in the line will be less than c, the speed
of propagation in free space. For lossless lines, the velocity of propagation on the line is
and the wavelength of the wave in the line is
c
v = (4.5)
ε
r
0
λ = λ ε
r
(4.6)
Example 4.1 A nearly lossless transmission line (R′ and G′ are very small) has an inductance
of 3.75 nH per unit length and a capacitance of 1.5 pF per unit length. Find the
characteristic impedance, Z 0 .
Solution
Z
0
=
=
L'
C'
–9
3.75×
10 H
–12
1.5×
10 F
3
= 2.5×
10 = 50 Ω
Following are equations for the characteristic impedance of various kinds of twoconductor
transmission lines.
(a) Parallel line:
Z 0
⎛ S ⎞
= 276 log ⎜
2 ⎟
(4.7)
ε ⎝ d ⎠