Practical_Antenna_Handbook_0071639586
C h a p t e r 3 : A n t e n n a B a s i c s 83 area.) Similarly, the application of a constant voltage across the resistor terminals will result in a steady current flow through the resistor until the voltage is removed. The power dissipated in a resistor is given by 2 P V I P V 2 = × or, alternatively, = or P = I × R (3.2) R Note that all three forms of Eq. (3.2) are equivalent and lead to the same answer. The resistance of an ideal resistor (i.e., one with no capacitance or inductance) does not depend on frequency. • Capacitors. A capacitor is a two-terminal component capable of storing electrical energy in the form of stored electric charges. The fundamental unit of capacitance is the farad, and the Capacitor total energy stored in a capacitor is ½ CV 2 , where C is the capacitance of the specific capacitor in question, and V is the voltage (in volts) across its terminals. Another useful relationship for capacitors is = × ∆ V I C ∆t (3.3) where I (in amperes) is the current through a capacitor of value C when the voltage across the capacitor terminals changes by some amount DV (in volts) over a short time interval Dt (in seconds). A simple capacitor you can make at home consists of two identical sheets of aluminum foil insulated from each other by a sheet of waxed paper of the same size. Note The only time current flows on the leads of a capacitor is when the voltage across its terminals is changing: I = C DV/Dt. A capacitor is said to exhibit capacitive reactance. Reactance works much like resistance to impede the flow of current in a circuit but the two are very different: 44 The reactance of an ideal capacitor does not dissipate any power. 44 The reactance of a capacitor is frequency dependent. The magnitude of capacitive reactance is defined in Eq. (3.4): 1 X = − (3.4) C 2 π fC where f is the frequency in hertz and C is the capacitance in farads. Alternatively, f can be in megahertz (MHz) and C in microfarads (µF). The minus sign is important in distinguishing the effect of capacitors from that of inductors. • Inductors. An inductor is a two-terminal component capable of storing magnetic energy in the form of current, or moving electric charges. The fundamental unit of inductance is Inductor the henry (abbreviated H), and the total energy stored in an inductor is ½ LI 2 , where L is the inductance of the device in question and I is the current (in amperes) going through it. Another useful relationship for inductors is
84 P a r t I I : F u n d a m e n t a l s = × ∆ I V L ∆t (3.5) where V (in volts) is the voltage across the inductor of value L when the current through the inductor changes by some amount DI (in amperes) over a short time interval Dt (in seconds). A simple inductor you can make at home is formed from a length of insulated wire (such as the wire left over from the installation of your new garage door opener) wrapped around your hand a few times. Note The only time a voltage can exist across the two terminals of an inductor is when the current through the inductor is changing: V = L DI/Dt. As discussed for capacitors, an ideal inductor does not dissipate power, nor are the current through, and the voltage across, the inductor in phase. The magnitude of inductive reactance is XL = 2πfL (3.6) where f is in hertz and L is in henries (or megahertz and microhenries). Note that inductive reactance X L is defined as positive, in contrast to capacitive reactance in Eq. (3.4). • Complex impedance. Suppose we apply a sinusoidal wave of frequency f across two terminals or nodes in a circuit consisting of interconnected resistors, inductors, and capacitors. If we measure, with an oscilloscope or other appropriate piece of test equipment, the voltage and current relationship at those terminals, we will generally find that while the current also varies sinusoidally at frequency f, it is not exactly in phase with the voltage as it would be if the circuit consisted of only resistors. The capacitors and inductors in the circuit cause phase shifts of varying degrees between currents and voltages in different parts of the circuit. At any one frequency we can describe the relationship between the voltage and the current at the two measurement terminals in terms of a single value of resistance in series with a single value of reactance. In equation form, we say ZIN = RIN + jX (3.7) IN or, more simply, Z = R + jX (3.8) This is known as a complex impedance because of the use of the complex plane mathematical operator j ( j = − 1 ) to represent reactances. The magnitude of X may be either positive or negative, depending on whether the net reactance seen appears to be inductive or capacitive, respectively. For correctness, we speak of R as the resistive part and X as the reactive part of the impedance Z. Both R and X are in ohms or multiples thereof.
- Page 50 and 51: C h a p t e r 2 : r a d i o - W a v
- Page 52 and 53: C h a p t e r 2 : r a d i o - W a v
- Page 54 and 55: C h a p t e r 2 : r a d i o - W a v
- Page 56 and 57: C h a p t e r 2 : r a d i o - W a v
- Page 58 and 59: C h a p t e r 2 : r a d i o - W a v
- Page 60 and 61: C h a p t e r 2 : r a d i o - W a v
- Page 62 and 63: C h a p t e r 2 : r a d i o - W a v
- Page 64 and 65: C h a p t e r 2 : r a d i o - W a v
- Page 66 and 67: C h a p t e r 2 : r a d i o - W a v
- Page 68 and 69: Figure 2.29C Monthly averaged sunsp
- Page 70 and 71: C h a p t e r 2 : r a d i o - W a v
- Page 72 and 73: C h a p t e r 2 : r a d i o - W a v
- Page 74 and 75: C h a p t e r 2 : r a d i o - W a v
- Page 76 and 77: C h a p t e r 2 : r a d i o - W a v
- Page 78 and 79: C h a p t e r 2 : r a d i o - W a v
- Page 80 and 81: C h a p t e r 2 : r a d i o - W a v
- Page 82 and 83: C h a p t e r 2 : r a d i o - W a v
- Page 84 and 85: C h a p t e r 2 : r a d i o - W a v
- Page 86 and 87: C h a p t e r 2 : r a d i o - W a v
- Page 88 and 89: C h a p t e r 2 : r a d i o - W a v
- Page 90 and 91: C h a p t e r 2 : r a d i o - W a v
- Page 92 and 93: C h a p t e r 2 : r a d i o - W a v
- Page 94 and 95: C h a p t e r 2 : r a d i o - W a v
- Page 96 and 97: C h a p t e r 2 : r a d i o - W a v
- Page 98 and 99: CHAPTER 3 Antenna Basics An antenna
- Page 102 and 103: C h a p t e r 3 : A n t e n n a B a
- Page 104 and 105: C h a p t e r 3 : A n t e n n a B a
- Page 106 and 107: C h a p t e r 3 : A n t e n n a B a
- Page 108 and 109: C h a p t e r 3 : A n t e n n a B a
- Page 110 and 111: C h a p t e r 3 : A n t e n n a B a
- Page 112 and 113: C h a p t e r 3 : A n t e n n a B a
- Page 114 and 115: C h a p t e r 3 : A n t e n n a B a
- Page 116 and 117: C h a p t e r 3 : A n t e n n a B a
- Page 118 and 119: C h a p t e r 3 : A n t e n n a B a
- Page 120 and 121: C h a p t e r 3 : A n t e n n a B a
- Page 122 and 123: C h a p t e r 3 : A n t e n n a B a
- Page 124 and 125: C h a p t e r 3 : A n t e n n a B a
- Page 126 and 127: CHAPTER 4 Transmission Lines and Im
- Page 128 and 129: C h a p t e r 4 : T r a n s m i s s
- Page 130 and 131: C h a p t e r 4 : T r a n s m i s s
- Page 132 and 133: C h a p t e r 4 : T r a n s m i s s
- Page 134 and 135: C h a p t e r 4 : T r a n s m i s s
- Page 136 and 137: C h a p t e r 4 : T r a n s m i s s
- Page 138 and 139: C h a p t e r 4 : T r a n s m i s s
- Page 140 and 141: C h a p t e r 4 : T r a n s m i s s
- Page 142 and 143: C h a p t e r 4 : T r a n s m i s s
- Page 144 and 145: C h a p t e r 4 : T r a n s m i s s
- Page 146 and 147: C h a p t e r 4 : T r a n s m i s s
- Page 148 and 149: C h a p t e r 4 : T r a n s m i s s
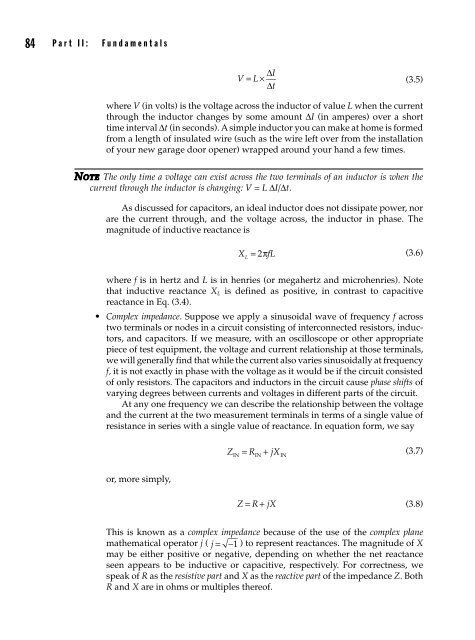
84 P a r t I I : F u n d a m e n t a l s<br />
= × ∆ I<br />
V L<br />
∆t<br />
(3.5)<br />
where V (in volts) is the voltage across the inductor of value L when the current<br />
through the inductor changes by some amount DI (in amperes) over a short<br />
time interval Dt (in seconds). A simple inductor you can make at home is formed<br />
from a length of insulated wire (such as the wire left over from the installation<br />
of your new garage door opener) wrapped around your hand a few times.<br />
Note The only time a voltage can exist across the two terminals of an inductor is when the<br />
current through the inductor is changing: V = L DI/Dt.<br />
As discussed for capacitors, an ideal inductor does not dissipate power, nor<br />
are the current through, and the voltage across, the inductor in phase. The<br />
magnitude of inductive reactance is<br />
XL = 2πfL<br />
(3.6)<br />
where f is in hertz and L is in henries (or megahertz and microhenries). Note<br />
that inductive reactance X L is defined as positive, in contrast to capacitive<br />
reactance in Eq. (3.4).<br />
• Complex impedance. Suppose we apply a sinusoidal wave of frequency f across<br />
two terminals or nodes in a circuit consisting of interconnected resistors, inductors,<br />
and capacitors. If we measure, with an oscilloscope or other appropriate<br />
piece of test equipment, the voltage and current relationship at those terminals,<br />
we will generally find that while the current also varies sinusoidally at frequency<br />
f, it is not exactly in phase with the voltage as it would be if the circuit consisted<br />
of only resistors. The capacitors and inductors in the circuit cause phase shifts of<br />
varying degrees between currents and voltages in different parts of the circuit.<br />
At any one frequency we can describe the relationship between the voltage<br />
and the current at the two measurement terminals in terms of a single value of<br />
resistance in series with a single value of reactance. In equation form, we say<br />
ZIN = RIN + jX<br />
(3.7)<br />
IN<br />
or, more simply,<br />
Z = R + jX (3.8)<br />
This is known as a complex impedance because of the use of the complex plane<br />
mathematical operator j ( j = − 1 ) to represent reactances. The magnitude of X<br />
may be either positive or negative, depending on whether the net reactance<br />
seen appears to be inductive or capacitive, respectively. For correctness, we<br />
speak of R as the resistive part and X as the reactive part of the impedance Z. Both<br />
R and X are in ohms or multiples thereof.