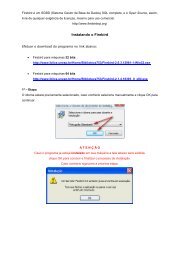
Curso de Equações Diferenciais Ordinárias - Unesp

É fácil verificar que
V (x, y) = x2 + y 2
2
é uma FLE para X em (0, 0) . Logo (0, 0) é uma singularidade assintoticamente
estável.
Corolário: Seja X : U → R 2 um campo de classe C 1 no aberto U ⊆ R 2
com uma singularidade p ∈ U. Suponhamos que V : U 0 → R é uma FL para X
em p tal que o conjunto
Z = {z ∈ U ∩ U 0 |V ′ X (z) = 0}
não contém nenhuma órbita positiva de X, exceto p, então p é uma singularidade
assintoticamente estável.
Exemplo: Seja
X (x, y) = ( y − x 3 , −x 3) .
É fácil verificar que
V (x, y) = x 4 + 2y 2
é uma FL que não é FLE. Porém o corolário acima garante que (0, 0) é uma
singularidade assintoticamente estável.
6.6 Conjuntos Limites e o Teorema de Poincaré-Bendixon
{ x
Consideremos um sistema de edo’s no plano
′ = f(x, y)
y ′ = g(x, y)
de classe C 1 em U ⊂ R 2 aberto.
Dizemos que Q ∈ ϖ(P ) ( Q está no ϖ-limite de P ) se
onde f, g são
∃t n → +∞ tal que ϕ(P, t n ) → Q
onde ϕ(P, t n ) denota o fluxo .
Dizemos que Q ∈ α(P ) ( Q está no α-limite de P ) se
∃t n → −∞ tal que ϕ(P, t n ) → Q.
Na verdade o ϖ-limite e o α-limite dependem da órbita γ que passa por P.
Assim mudaremos nossa notação para ϖ(γ) e α(γ).
Exemplos:
1) Considere a órbita γ do exemplo 1 da seção anterior:
γ = {(0, 0)} ⇒ ϖ(γ) = α(γ) = {(0, 0)}
γ ≠ {(0, 0)} ⇒ ϖ(γ) = {(0, 0)} e α(γ) = ∅
93