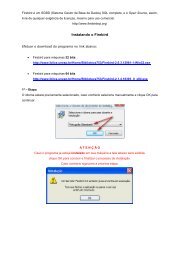
Curso de Equações Diferenciais Ordinárias - Unesp

e como
segue que
y (0) = −1
− 2 3 + c = −1
e portanto
e
c = − 1 3
y = − 2 3 e−t − 1 3 e t 2
Teorema: Sejam p, g funções reais contínuas definidas em um intervalo
aberto I e t 0 ∈ I. O PVI
{ y ′ + p (t) y = g (t)
admite uma única solução
y (t 0 ) = y 0
φ : I → R.
Prova:
Vamos proceder da mesma maneira que procedemos no exemplo acima.
Inicialmente encontremos uma função µ que satisfaça
É trivial vermos que
µ ′ = pµ.
R t
p(s)ds
t
µ (t) = e 0
satisfaz o desejado. µ é chamada de FATOR INTEGRANTE. Temos:
Como
segue que
y ′ + py = g ⇒ y ′ µ + pµy = µg ⇒
⇒
y ′ µ + µ ′ y = µg ⇒ (yµ) ′ = µg ⇒
∫ t
⇒ yµ (t) = g (s) µ (s) ds + k ⇒
t 0
⇒ y = e − R (∫
t
t R
)
p(s)ds
s
t 0 t p(u)du 0 g (s) ds + k
y (t 0 ) = y 0
k = y 0
t 0
e
e que a solução do PVI é
y = e − R (∫
t
t R
)
p(s)ds
s
t 0 t
e
p(u)du 0 g (s) ds + y 0 .
t 0
6