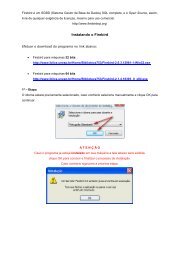
Curso de Equações Diferenciais Ordinárias - Unesp

a)
{y 1 , y 2 }
é um conjunto fundamental de soluções.
b)
{y 1 , y 2 }
é um conjunto LI.
c)
para algum t 0 ∈ I.
d)
W (y 1 , y 2 ) (t 0 ) ≠ 0
W (y 1 , y 2 ) (t) ≠ 0, ∀t ∈ I.
Prova:
O Teorema de Abel nos garante
c) ⇔ d)
c) ⇒ b)
Sejam y 1 e y 2 soluções satisfazendo que W (y 1 , y 2 ) (t 0 ) ≠ 0 para algum t 0 ∈ I.
Queremos provar que y 1 e y 2 são LI.
Suponhamos que
αy 1 + βy 2 = 0.
Assim α e β são soluções do sistema
{
y1 (t 0 ) α + y 2 (t 0 )β = 0
y ′ 1 (t 0 ) α + y ′ 2(t 0 )β = 0
Como o determinante da matriz dos coeficientes do sistema é não nulo, temos
que tal sistema admite somente a solução trivial. Logo
α = β = 0.
b) ⇒ d) :
Suponhamos que exista t 0 ∈ I tal que
W (y 1 , y 2 ) (t 0 ) = 0
Assim o sistema abaixo admite solução diferente da trivial
{
y1 (t 0 ) α + y 2 (t 0 )β = 0
y ′ 1 (t 0 ) α + y ′ 2(t 0 )β = 0
Seja
φ = αy 1 + βy 2
44