Autovalores do Laplaciano - Departamento de Matemática - UFMG
Autovalores do Laplaciano - Departamento de Matemática - UFMG
Autovalores do Laplaciano - Departamento de Matemática - UFMG
You also want an ePaper? Increase the reach of your titles
YUMPU automatically turns print PDFs into web optimized ePapers that Google loves.
Rodney Josué Biezuner 57<br />
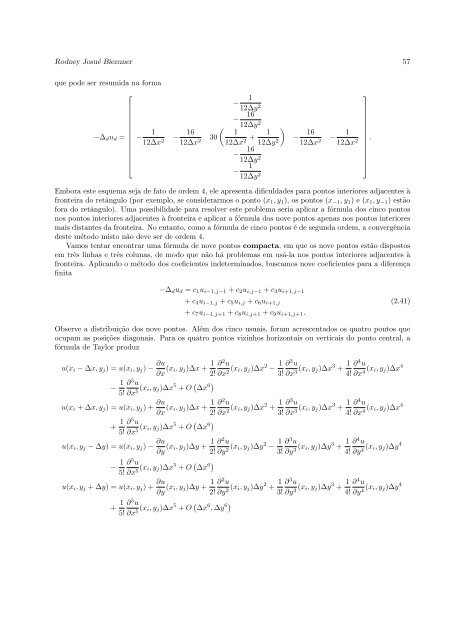
que po<strong>de</strong> ser resumida na forma<br />
⎡<br />
⎢<br />
−∆dud = ⎢<br />
⎣<br />
− 1 16<br />
−<br />
12∆x2 12∆x2 − 1<br />
12∆y2 − 16<br />
12∆y2 <br />
1 1<br />
30 +<br />
12∆x2 12∆y2 <br />
− 16<br />
12∆y2 − 1<br />
12∆y2 − 16 1<br />
−<br />
12∆x2 12∆x2 Embora este esquema seja <strong>de</strong> fato <strong>de</strong> or<strong>de</strong>m 4, ele apresenta dificulda<strong>de</strong>s para pontos interiores adjacentes à<br />
fronteira <strong>do</strong> retângulo (por exemplo, se consi<strong>de</strong>rarmos o ponto (x1, y1), os pontos (x−1, y1) e (x1, y−1) estão<br />
fora <strong>do</strong> retângulo). Uma possibilida<strong>de</strong> para resolver este problema seria aplicar a fórmula <strong>do</strong>s cinco pontos<br />
nos pontos interiores adjacentes à fronteira e aplicar a fórmula <strong>do</strong>s nove pontos apenas nos pontos interiores<br />
mais distantes da fronteira. No entanto, como a fórmula <strong>de</strong> cinco pontos é <strong>de</strong> segunda or<strong>de</strong>m, a convergência<br />
<strong>de</strong>ste méto<strong>do</strong> misto não <strong>de</strong>ve ser <strong>de</strong> or<strong>de</strong>m 4.<br />
Vamos tentar encontrar uma fórmula <strong>de</strong> nove pontos compacta, em que os nove pontos estão dispostos<br />
em três linhas e três colunas, <strong>de</strong> mo<strong>do</strong> que não há problemas em usá-la nos pontos interiores adjacentes à<br />
fronteira. Aplican<strong>do</strong> o méto<strong>do</strong> <strong>do</strong>s coeficientes in<strong>de</strong>termina<strong>do</strong>s, buscamos nove coeficientes para a diferença<br />
finita<br />
−∆dud = c1ui−1,j−1 + c2ui,j−1 + c3ui+1,j−1<br />
+ c4ui−1,j + c5ui,j + c6ui+1,j<br />
+ c7ui−1,j+1 + c8ui,j+1 + c9ui+1,j+1.<br />
⎤<br />
⎥ .<br />
⎥<br />
⎦<br />
(2.41)<br />
Observe a distribuição <strong>do</strong>s nove pontos. Além <strong>do</strong>s cinco usuais, foram acrescenta<strong>do</strong>s os quatro pontos que<br />
ocupam as posições diagonais. Para os quatro pontos vizinhos horizontais ou verticais <strong>do</strong> ponto central, a<br />
fórmula <strong>de</strong> Taylor produz<br />
u(xi − ∆x, yj) = u(xi, yj) − ∂u<br />
∂x (xi, yj)∆x + 1<br />
2!<br />
− 1 ∂<br />
5!<br />
5u ∂x5 (xi, yj)∆x 5 + O ∆x 6<br />
u(xi + ∆x, yj) = u(xi, yj) + ∂u<br />
∂x (xi, yj)∆x + 1<br />
2!<br />
+ 1 ∂<br />
5!<br />
5u ∂x5 (xi, yj)∆x 5 + O ∆x 6<br />
u(xi, yj − ∆y) = u(xi, yj) − ∂u<br />
∂y (xi, yj)∆y + 1<br />
2!<br />
− 1 ∂<br />
5!<br />
5u ∂x5 (xi, yj)∆x 5 + O ∆x 6<br />
u(xi, yj + ∆y) = u(xi, yj) + ∂u<br />
∂y (xi, yj)∆y + 1<br />
2!<br />
+ 1 ∂<br />
5!<br />
5u ∂x5 (xi, yj)∆x 5 + O ∆x 6 , ∆y 6<br />
∂2u ∂x2 (xi, yj)∆x 2 − 1 ∂<br />
3!<br />
3u ∂x3 (xi, yj)∆x 3 + 1 ∂<br />
4!<br />
4u ∂x4 (xi, yj)∆x 4<br />
∂2u ∂x2 (xi, yj)∆x 2 + 1 ∂<br />
3!<br />
3u ∂x3 (xi, yj)∆x 3 + 1 ∂<br />
4!<br />
4u ∂x4 (xi, yj)∆x 4<br />
∂2u ∂y2 (xi, yj)∆y 2 − 1 ∂<br />
3!<br />
3u ∂y3 (xi, yj)∆y 3 + 1 ∂<br />
4!<br />
4u ∂y4 (xi, yj)∆y 4<br />
∂2u ∂y2 (xi, yj)∆y 2 + 1 ∂<br />
3!<br />
3u ∂y3 (xi, yj)∆y 3 + 1 ∂<br />
4!<br />
4u ∂y4 (xi, yj)∆y 4