Autovalores do Laplaciano - Departamento de Matemática - UFMG

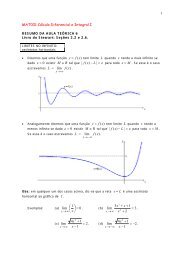
Rodney Josué Biezuner 110
se ∆x é pequeno podemos aproximar
ρ (RJ,ω) ≈ 1 − ω π2
2 ∆x2 + O ∆x 4 ,
R∞ (RJ,ω) ≈ ω π2
2 ∆x2 .
Vemos que a velocidade de convergência do método de Jacobi amortecido é da mesma ordem que a do método
de Jacobi, um pouco pior para valores de ω próximos de 1 e muito pior para valores de ω próximos de 0.
4.3.5 Resumo
Método ρ (R) R∞ (R)
Jacobi cos (π∆x)
π 2
2 ∆x2 + O ∆x 4
Gauss-Seidel cos 2 (π∆x) π 2 ∆x 2 + O ∆x 4
SOR ótimo 1 − 2π∆x + O ∆x 2
2π∆x + O ∆x 2
Jacobi amortecido 1 − ω π2
2 ∆x2 + O ∆x 4 ω π2
2 ∆x2 + O ∆x 4
4.4 Método do Gradiente Conjugado
Nesta seção, A será sempre uma matriz real simétrica, positiva definida. Neste caso, a resolução do sistema
Ax = b é equivalente à resolução de um problema de minimização de um funcional quadrático:
4.24 Teorema. (Método Variacional para a Resolução de Sistemas Lineares) Seja A ∈ Mn (R) uma matriz
simétrica positiva definida e b ∈ R n . Então a solução do sistema
Ax = b
é o único ponto x que minimiza o funcional quadrático
f (y) = 1
2 yt Ay − y t b. (4.60)
Prova: Uma matriz simétrica positiva definida é invertível, logo existe uma única solução x para o sistema
Ax = b. Para provar o teorema, começamos observando que, como y t Ax ∈ R é um escalar, temos
Daí,
y t Ax = y t Ax t = x t A t y = x t Ay.
f (y) − f (x) = 1
2 yt Ay − y t b − 1
2 xt Ax + x t b
= 1
2 yt Ay − y t Ax − 1
2 xt Ax + x t Ax
= 1
2 yt Ay − y t Ax + 1
2 xt Ax
= 1
2 yt Ay − 1
2 yt Ax − 1
2 xt Ay + 1
2 xt Ax
= 1
2 yt A (y − x) − 1
2 xt A (y − x)