Jaarboek no. 89. 2010/2011 - Koninklijke Maatschappij voor ...

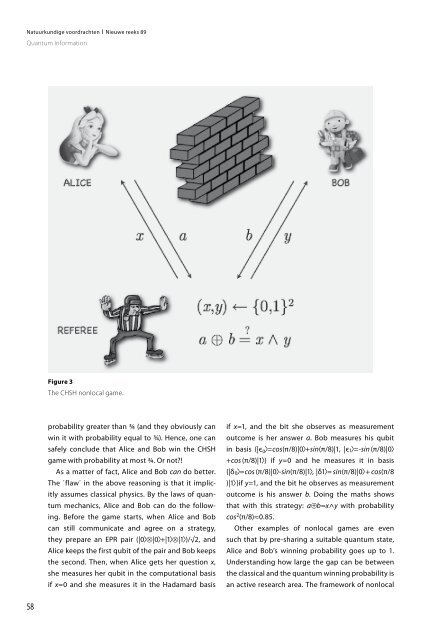
Natuurkundige voordrachten I Nieuwe reeks 89
Quantum Information
58
Figure 3
The CHSH nonlocal game.
probability greater than ¾ (and they obviously can
win it with probability equal to ¾). Hence, one can
safely conclude that Alice and Bob win the CHSH
game with probability at most ¾. Or not?!
As a matter of fact, Alice and Bob can do better.
The ΄flaw΄ in the above reasoning is that it implicitly
assumes classical physics. By the laws of quantum
mechanics, Alice and Bob can do the following.
Before the game starts, when Alice and Bob
can still communicate and agree on a strategy,
they prepare an EPR pair (|0>⊗|0>+|1>⊗|1>)/√2, and
Alice keeps the first qubit of the pair and Bob keeps
the second. Then, when Alice gets her question x,
she measures her qubit in the computational basis
if x=0 and she measures it in the Hadamard basis
if x=1, and the bit she observes as measurement
outcome is her answer a. Bob measures his qubit
in basis {|є0>=cos(π/8)|0>+sin(π/8)|1, |є1>=-sin (π/8)|0>
+cos (π/8)|1>} if y=0 and he measures it in basis
{|δ0>=cos (π/8)|0>-sin(π/8)|1>, |δ1>= sin(π/8)|0> + cos(π/8
)|1> }if y=1, and the bit he observes as measurement
outcome is his answer b. Doing the maths shows
that with this strategy: a⊕b=x^y with probability
cos2 (π/8)≈0.85.
Other examples of nonlocal games are even
such that by pre-sharing a suitable quantum state,
Alice and Bob’s winning probability goes up to 1.
Understanding how large the gap can be between
the classical and the quantum winning probability is
an active research area. The framework of nonlocal