Appunti di Calcolo Numerico - Esercizi e Dispense - Università degli ...

2. RICHIAMI DI ANALISI
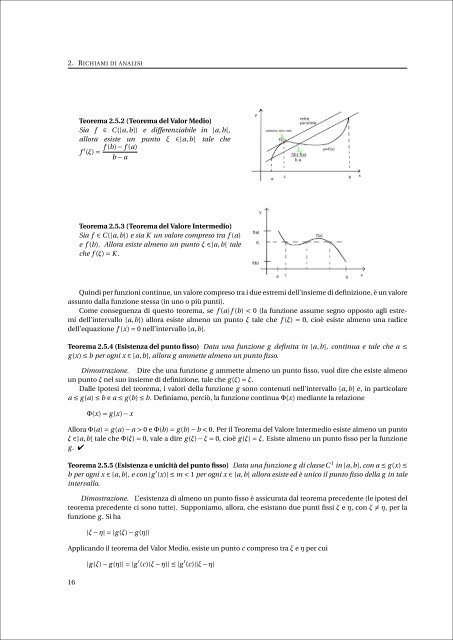
Teorema 2.5.2 (Teorema del Valor Medio)
Sia f ∈ C ([a,b]) e differenziabile in ]a,b[,
allora esiste un punto ξ ∈]a,b[ tale che
f ′ f (b) − f (a)
(ξ) =
b − a
Teorema 2.5.3 (Teorema del Valore Intermedio)
Sia f ∈ C ([a,b]) e sia K un valore compreso tra f (a)
e f (b). Allora esiste almeno un punto ξ ∈]a,b[ tale
che f (ξ) = K .
Quindi per funzioni continue, un valore compreso tra i due estremi dell’insieme di definizione, è un valore
assunto dalla funzione stessa (in uno o più punti).
Come conseguenza di questo teorema, se f (a)f (b) < 0 (la funzione assume segno opposto agli estremi
dell’intervallo [a,b]) allora esiste almeno un punto ξ tale che f (ξ) = 0, cioè esiste almeno una radice
dell’equazione f (x) = 0 nell’intervallo [a,b].
Teorema 2.5.4 (Esistenza del punto fisso) Data una funzione g definita in [a,b], continua e tale che a ≤
g (x) ≤ b per ogni x ∈ [a,b], allora g ammette almeno un punto fisso.
Dimostrazione. Dire che una funzione g ammette almeno un punto fisso, vuol dire che esiste almeno
un punto ξ nel suo insieme di definizione, tale che g (ξ) = ξ.
Dalle ipotesi del teorema, i valori della funzione g sono contenuti nell’intervallo [a,b] e, in particolare
a ≤ g (a) ≤ b e a ≤ g (b) ≤ b. Definiamo, perciò, la funzione continua Φ(x) mediante la relazione
Φ(x) = g (x) − x
Allora Φ(a) = g (a) − a > 0 e Φ(b) = g (b) − b < 0. Per il Teorema del Valore Intermedio esiste almeno un punto
ξ ∈]a,b[ tale che Φ(ξ) = 0, vale a dire g (ξ) − ξ = 0, cioè g (ξ) = ξ. Esiste almeno un punto fisso per la funzione
g . ✔
Teorema 2.5.5 (Esistenza e unicità del punto fisso) Data una funzione g di classe C 1 in [a,b], con a ≤ g (x) ≤
b per ogni x ∈ [a,b], e con |g ′ (x)| ≤ m < 1 per ogni x ∈ [a,b] allora esiste ed è unico il punto fisso della g in tale
intervallo.
Dimostrazione. L’esistenza di almeno un punto fisso è assicurata dal teorema precedente (le ipotesi del
teorema precedente ci sono tutte). Supponiamo, allora, che esistano due punti fissi ξ e η, con ξ ≠ η, per la
funzione g . Si ha
|ξ − η| = |g (ξ) − g (η)|
Applicando il teorema del Valor Medio, esiste un punto c compreso tra ξ e η per cui
16
|g (ξ) − g (η)| = |g ′ (c)(ξ − η)| ≤ |g ′ (c)||ξ − η|