Appunti di Calcolo Numerico - Esercizi e Dispense - Università degli ...

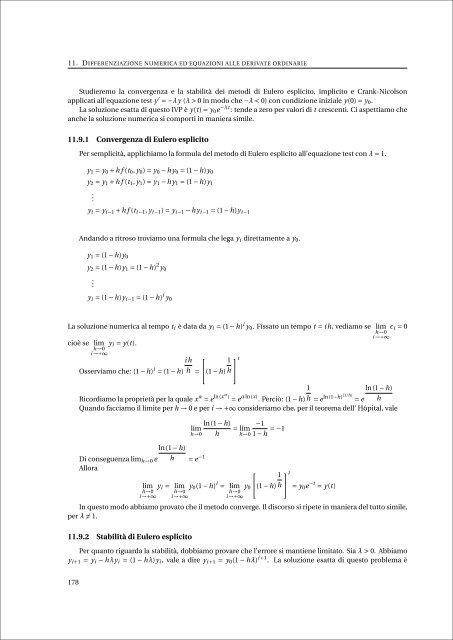
11. DIFFERENZIAZIONE NUMERICA ED EQUAZIONI ALLE DERIVATE ORDINARIE
Studieremo la convergenza e la stabilità dei metodi di Eulero esplicito, implicito e Crank-Nicolson
applicati all’equazione test y ′ = −λy (λ > 0 in modo che −λ < 0) con condizione iniziale y(0) = y 0 .
La soluzione esatta di questo IVP è y(t) = y 0 e −λt : tende a zero per valori di t crescenti. Ci aspettiamo che
anche la soluzione numerica si comporti in maniera simile.
11.9.1 Convergenza di Eulero esplicito
Per semplicità, applichiamo la formula del metodo di Eulero esplicito all’equazione test con λ = 1.
y 1 = y 0 + h f (t 0 , y 0 ) = y 0 − hy 0 = (1 − h)y 0
y 2 = y 1 + h f (t 1 , y 1 ) = y 1 − hy 1 = (1 − h)y 1
.
y i = y i−1 + h f (t i−1 , y i−1 ) = y i−1 − hy i−1 = (1 − h)y i−1
Andando a ritroso troviamo una formula che lega y i direttamente a y 0 .
y 1 = (1 − h)y 0
y 2 = (1 − h)y 1 = (1 − h) 2 y 0
.
y i = (1 − h)y i−1 = (1 − h) i y 0
La soluzione numerica al tempo t i è data da y i = (1 − h) i y 0 . Fissato un tempo t = i h, vediamo se
cioè se
lim
h→0
i→+∞
y i = y(t).
i h
⎡
1
⎤
Osserviamo che: (1 − h) i = (1 − h) h = ⎣(1 − h) h ⎦
t
lim
h→0
i→+∞
Ricordiamo la proprietà per la quale x α = e ln(xα) = e αln(x) . Perciò: (1 − h) h = e ln(1−h)(1/h) = e h
Quando facciamo il limite per h → 0 e per i → +∞ consideriamo che, per il teorema dell’ Hôpital, vale
Di conseguenza lim h→0 e
Allora
lim
h→0
i→+∞
ln(1 − h)
h
y i = lim
h→0
i→+∞
ln(1 − h) −1
lim = lim
h→0 h h→0 1 − h = −1
= e −1
⎡
y 0 (1 − h) i = lim y 0 h→0
i→+∞
1
⎤t
⎣(1 − h) h ⎦ = y 0 e −t = y(t)
1
ln(1 − h)
ɛ i = 0
In questo modo abbiamo provato che il metodo converge. Il discorso si ripete in maniera del tutto simile,
per λ ≠ 1.
11.9.2 Stabilità di Eulero esplicito
Per quanto riguarda la stabilità, dobbiamo provare che l’errore si mantiene limitato. Sia λ > 0. Abbiamo
y i+1 = y i − hλy i = (1 − hλ)y i , vale a dire y i+1 = y 0 (1 − hλ) i+1 . La soluzione esatta di questo problema è
178