Trave appoggiata a un estremo e incastrata all'altro, con carico ... - Sei

12 Travi iperstatiche 12.1 Travi iperstatiche a una campata
12.1.3 Trave appoggiata a un estremo e incastrata all’altro, gravata di un carico concentrato
Trave appoggiata a un estremo e incastrata all’altro,
con carico ripartito triangolare
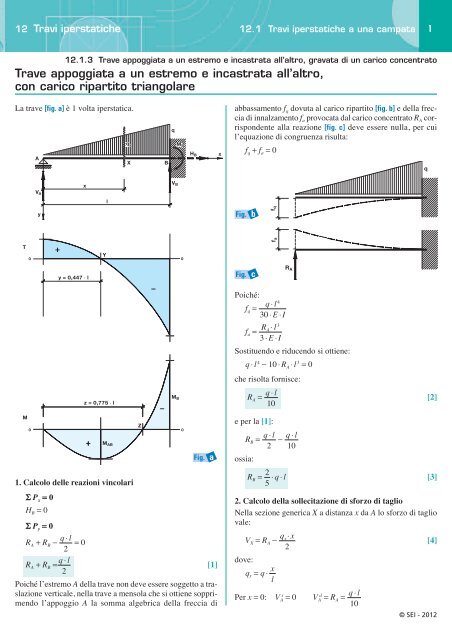
La trave [fig. a] è 1 volta iperstatica.
T
M
V A
1. Calcolo delle reazioni vincolari
S P x = 0
H B = 0
x
Y
0 0
0
A
y
y = 0,447 ◊ l
z = 0,775 ◊ l
l
M AB
S Py = 0
q ⋅ l
RA + RB − =0
2
q ⋅ l
RA + RB = [1]
2
Poiché l’estremo A della trave non deve essere soggetto a traslazione
verticale, nella trave a mensola che si ottiene sopprimendo
l’appoggio A la somma algebrica della freccia di
q x
X
Z
B
q
V B
M B
M B
0
H B
Fig. a
x
abbassamento f q dovuta al carico ripartito [fig. b] e della freccia
di innalzamento f a provocata dal carico concentrato R A corrispondente
alla reazione [fig. c] deve essere nulla, per cui
l’equazione di congruenza risulta:
f q + f a = 0
Fig. b
Fig. c
f q
f a
Poiché:
fq =
RA ⋅ l
fa =
3
q ⋅ l
3 ⋅ E ⋅ I
4
30 ⋅ E ⋅ I
R A
Sostituendo e riducendo si ottiene:
q ⋅ l 4 − 10 ⋅ R A ⋅ l 3 = 0
che risolta fornisce:
q ⋅ l
RA = [2]
10
e per la [1]:
q ⋅ l
RB = −
2
ossia:
2
RB = ⋅ q ⋅ l [3]
5
2. Calcolo della sollecitazione di sforzo di taglio
Nella sezione generica X a distanza x da A lo sforzo di taglio
vale:
q
VX = RA − x⋅ x
[4]
2
dove:
qx = q ⋅
x
l
q ⋅ l
10
Per x = 0: V A s = 0 VA d = RA =
q ⋅ l
10
q
1
© SEI - 2012

12 Travi iperstatiche 12.1 Travi iperstatiche a una campata
12.1.3 Trave appoggiata a un estremo e incastrata all’altro, gravata di un carico concentrato
s q ⋅ l q ⋅ l 2
Per x = l: VB = − =− ⋅q⋅l = RB
10 2 5
Uguagliando a zero l’equazione del taglio si ricava l’ascissa
della sezione ove si ha V = 0:
l
y = ≈ 0,4472 ⋅ l
5
3. Calcolo della sollecitazione di momento flettente
In una sezione generica X il momento flettente è dato da:
q
MX = RA ⋅ x − ⋅ = ⋅ x − x⋅ x
[5]
Per x = 0: MA = 0
3
qx⋅ x x q ⋅ l
2 3 10 6 ⋅ l
Per x = :
MAB = M + max = [6]
Per x = l:
MB = M − q ⋅ l
max = − [7]
2
q ⋅ l
15
2
l
5
15 ⋅ 5
Uguagliando a zero la [5] si ricava la posizione della sezione
Z di momento nullo, che fornisce il valore z ≈ 0,775 ⋅ l.
4. Calcolo della rotazione
Si applica il principio di sovrapposizione degli effetti, sopprimendo
l’incastro in B e sostituendolo con il momento d’incastro
M B [fig. d], per cui la rotazione α in A è data dalla
somma algebrica della rotazione α q dovuta al carico ripartito
gravante sulla trave considerata appoggiata, e dalla rotazione
α m dovuta al momento M B agente in B sulla trave considerata
scarica, ossia:
α = αq + αm ed essendo:
q ⋅ l MB ⋅ l
αq = ⋅ αm = = −
6 ⋅ E ⋅ I
3 7
360 E ⋅ I
q ⋅ l 3
90 ⋅ E ⋅ I
sostituendo e risolvendo si ottiene:
q ⋅ l
α = [8]
3
120 ⋅ E ⋅ I
5. Calcolo della freccia in mezzeria
Con le indicazioni della figura d risulta:
f = fq + fm ed essendo:
MB ⋅ l
fq = ⋅ fm = = −
2
q ⋅ l
16 ⋅ E ⋅ I
4
l
2
5
768 E ⋅ I
sostituendo si ottiene:
q ⋅ l
f = ⋅ [9]
4 3
1280 E ⋅ I
l
2
Fig. d
A
A
α
f l 2
f
q ⋅ l 4
240 ⋅ E ⋅ I
A B
αq fq αm fm A B
B
q
q
q
B
M B
M B
2
© SEI - 2012