Tema 6: Derivadas. Técnicas de derivación

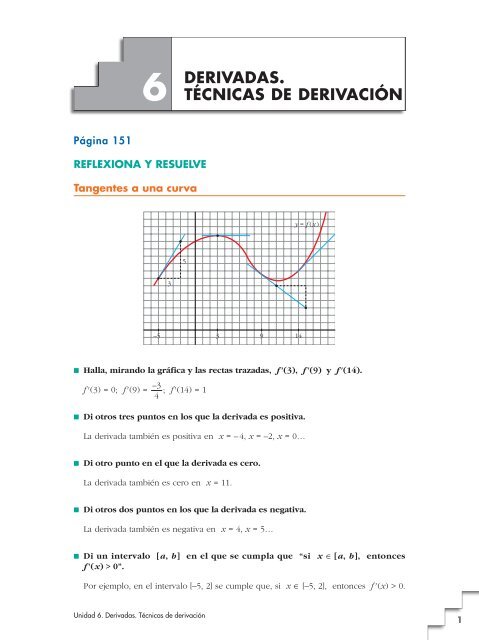
Función derivada■ Continúa escribiendo las razones por las cuales g (x) es una función cuyo comportamientoresponde al de la derivada de f (x).• En el intervalo (a, b), f (x)es decreciente. Por tanto, suderivada es negativa. Es loque le pasa a g(x) en (a, b).y = f (x)• La derivada de f en b es 0:f'(b) = 0. Y también esg(b) =0.ab• En general:g(x) = f'(x) = 0 donde f (x)tiene tangente horizontal.g(x) = f'(x) > 0 donde f (x)es creciente.g(x) = f'(x) < 0 donde f (x)es decreciente.aby = g(x) = f'(x)■ Las tres gráficas de abajo, A, B y C, son las funciones derivadas de las gráficasde arriba, 1, 2 y 3, pero en otro orden.Explica razonadamente cuál es la de cada una.1) B1232) A3) CLa derivada se anula en lospuntos de tangente horizontal,es positiva donde la función escreciente, y es negativa dondela función decrece.ABC2Unidad 6. Derivadas. Técnicas de derivación

UNIDAD6Página 153° 2 – 3x, x Ì 21. f (x) = ¢£ x 2 – 3, x > 2¿Es derivable en x 0= 2?lím f (x) = lím (2 – 3x) = –4x 8 2 – x 8 2 –límx 8 2 + f (x) = lím (x 2 – 3) = 1x 8 2 +La función no es continua en x = 2, pues límx 8 2 – f (x) ? límx 8 2 + f (x).Por tanto, tampoco es derivable en x = 2.° 2 – 3x, x Ì 22. f (x) = ¢¿Es derivable en x 0= 2?£ x 2 – 8, x > 2lím f (x) = lím (2 – 3x) = –4x 8 2 – x 8 2 –lím f (x) = lím (x 2 – 8) = –4x 8 2 + x 8 2 +La función es continua, pues: límx 8 2 – f (x) = lím f (x) = f (2) = –4.x 8 2 +° –3 si x < 2f'(x) = ¢£ 2x si x > 2f'(2 – ) = –3 ? f'(2 + ) = 4Por tanto, f (x) no es derivable en x = 2.Página 1571. Calcula la derivada de cada una de las siguientes funciones:1 – x1 – xa) f (x) = b) f (x) =1 + x√ 1 + x1 – x1 – tg xc) f (x) = ln d) f (x) =1 + x1 + tg x1 – tg xe) f (x) = f ) f (x) = ln√ 1 + tg x√3 x +1√e tg xg) f (x) = h) f (x) = log (sen x · cos x) 2i) f (x) = sen 2 x + cos 2 x + x j) f (x) = sen √x +1· cos √x – 1Unidad 6. Derivadas. Técnicas de derivación3

k) f (x) = 7 sen (x2 + 1)l) f (x)= sen (3x 5 – 2 √x + √2x)m) f (x) = n) f (x) = cos 2 3√sen x + x 2 +1√x + (3 – x) 2a) f'(x) =–1 · (1 + x) – (1 – x) · 1=–1 – x – 1 + x=–2(1 + x) 2 (1 + x) 2 (1 + x) 2b) Utilizamos el resultado obtenido en a):f'(x) =1–1·–2=(1 + x) 2 √(1 – x)(1 + x)2 √ 1 – x31 + xc) Utilizamos el resultado obtenido en a):f'(x) =1·–2=–2(1 + x)=1 – x (1 + x) 2 (1 – x)(1 + x) 21 + xDe otra forma: Si tomamos logaritmos previamente:f (x) = ln (1 – x) – ln (1 + x). Derivamos:–1 1f'(x) = – =–1 – x – 1 + x=–21 – x 1 + x 1 – x 2 1 – x 2d) f'(x) =– (1 + tg 2 x)(1 + tg x) – (1 – tg x) · (1 + tg 2 x)=(1 + tg x) 2=(1 + tg 2 x)[–1 – tg x – 1 + tg x]=– 2(1 + tg 2 x)(1 + tg x) 2(1 + tg x) 2De otra forma: Si tenemos en cuenta el resultado obtenido en a):f'(x) =–2· D [tg x] =–2· (1 + tg 2 x) =– 2(1 + tg 2 x)(1 + tg x) 2 (1 + tg x) 2(1 + tg x) 2e) Teniendo en cuenta lo obtenido en d):f'(x) =1·– 2(1 + tg 2 x) – (1 + tg=2 x)(1 + tg x) 2 √(1 – tg x)(1 + tg x)2 √ 1 – tg x31 + tg xTambién podríamos haber llegado a este resultado utilizando lo obtenido en b).f) f (x) = ln √e tg x = ln e (tg x) / 2 tg x=2f'(x) =1 + tg 2 x2√3 x + 1g) f (x) = = 3(x + 1) / 2f'(x) = 3 (x + 1) / 2 1 ln 3· · ln 3 = · √3 x + 122–21 – x 234Unidad 6. Derivadas. Técnicas de derivación

UNIDAD6h) f (x) = log (sen x · cos x) 2 = 2[log (sen x + log (cos x)]cos xsen xf'(x) = 2[· + ·] = · =4 cos= · 2 x – sen 2 x 4 cos 2x= · =ln 10 2sen x · cos x ln 10 sen 2xDe otra forma:1ln 10–sen xcos xf (x) = log (sen x · cos x) 2 sen 2x= 2 log( 2 )1f'(x) = 2 · ·cos 2x=ln 10 sen 2x2i) f (x) = sen 2 x + cos 2 x + x = 1 + xf'(x) = 11ln 102ln 104ln 10 · tg 2xcos 2 x – sen 2 xsen x · cos x4ln 10 · tg 2xcos √ — x + 1 · cos √ — x – 1j) f'(x) = + =2 √x + 12 √x – 1sen √ — x + 1 · (– sen √ — x – 1)cos √ — x + 1 · cos √ — x – 1= –2 √x + 1sen √ — x + 1 · sen √ — x – 12 √x – 1k) f'(x) = 7 sen(x2 + 1) · ln 7 · D [sen(x 2 + 1)] = 7 sen(x2 +1) · ln 7 · 2x · cos (x 2 +1)3l) f'(x) = cos (3x 5 31– 2 √x + √2x ) ·( 15x4 – + √2)√x3 3 √x 2m) f'(x) =1· (cos x + 2x) =2√sen x + x 2 +1cos x + 2x2√sen x + x 2 +1n)331 + 2(3 – x) · (–1)f'(x) = 2cos √x + (3 – x) 2 · [–sen √x + (3 – x) 2 ] · 3√(x + (3 – x) 2 ) 2=–2 cos 3 √ — x +( — 3 – x)= sen 3 √ — x +( — 3 – x) 2 · (2x – 5)=3 3 √x + (3 – x) 2 ) 2(5 – 2x) · sen (2 3 √x + (3 – x) 2 )=3 3 √(x + (3 – x) 2 ) 22. Halla las derivadas 1. a , 2. a y 3. a de las siguientes funciones:a) y = x 5 b) y = x cos x c) y = sen 2 x + cos 2 x + xa) y = x 5y' = 5x 4 ; y'' = 20x 3 ; y''' = 60x 2Unidad 6. Derivadas. Técnicas de derivación5

) y = x cos xy' = cos x – x sen xy'' = –sen x – sen x – x cos x = –2sen x – x cos xy''' = –2cos x – cos x + x sen x = –3cos x + x sen xc) y = sen 2 x + cos 2 x + x = 1 + xy' = 1; y'' = 0; y''' = 03. Calcula f'(1) siendo: f (x) =√ x — 3 √ 3x— · e 415f (x) =√ — x 3 √ — 3x· e 4 3=x 1/2 · 3 1/3 · x 1/3 · e 4= 2/15 · e 4· x 13/30 √9 · e= 4· x 13/302 5 √3x 2 2 · 3 1/5 · x 2/5 2215√9 · ef'(x) = 4 13· x –17/30 =3 3013 15 √9 · e 46030√x 172 5 √3x 2 Unidad 6. Derivadas. Técnicas de derivaciónPor tanto: f'(1) =13 15 √9 · e 4604. Calcula f'(π/6) siendo: f (x) = (cos 2 3x – sen 2 3x) · sen 6xf (x) = (cos 2 3x – sen 2 sen 12x3x) · sen 6x = cos 6x · sen 6x =2f'(x) =12cos 12x2= 6cos 12xPor tanto:πf'( ) = 6 · cos12π= 6 · cos (2π) = 6 · 1 = 6665. Calcula f'(0) siendo:1f (x) = ln √x 2 + x + 1 – · (2x + 1) 2√31f (x) = ln – (2x + 1) 2 = ln (x 2 1√x 2 + x + 11+ x + 1) – (2x + 1) 2√32√31f'(x) = ·2x + 1 4– · (2x + 1) 2 4(2x + 1)=2x + 1– =2 x 2 + x + 1 √32x 2 + 2x + 2 √3=2x + 1 8x +4– =2x 2 + 2x + 2 √3Por tanto: f'(0) = √— 3 – 82√ — 3–16x 3 – 24x 2 + (2√ — 3 – 24)x + √ — 3 – 8√ — 3(2x 2 + 2x + 2)6

°§§¢£°§°§§¢£UNIDAD6Página 1581. Estudia la derivabilidad en x 0= 3 de la función:° x 2 – 3x, x Ì 3f (x) = ¢£ 3x – 9, x > 3• Continuidad en x 0= 3:lím f (x) = lím (x 2 – 3x) = 0 lím f (x) = f (3) = 0x 8 3 – x 8 3x 8 3lím f (x) = lím (3x – 9) = 0 Por tanto, f (x) es continua en x 0= 3.x 8 3 + x 8 3• Derivabilidad en x 0= 3:§°§¢£límx 8 3 –límx 8 3 +f'(x) = lím (2x – 3) = 3 = f'(3 – )x 8 3 –f'(x) = lím (3) = 3 = f'(3 + )x 8 3 +Las derivadas laterales existeny coinciden.Por tanto, f (x) es derivable en x 0= 3. Además, f'(3) = 3.2. Calcula m y n para que f (x) sea derivable en Á:° x 2 – mx + 5, x Ì 0f (x) = ¢£ –x 2 + n, x > 0• Si x ? 0, la función es continua y derivable, pues está formada por dos polinomios.• Continuidad en x = 0:lím f (x) = lím (x 2 – mx + 5) = 5x 8 0 – x 8 0lím f (x) = lím (–x 2 Para que f (x) sea continua en x = 0,+ n) = nx 8 0 + x 8 0ha de ser: n = 5f (0) = 5• Derivabilidad en x = 0:§¢§£§límx 8 0 –límx 8 0 +f'(x) = lím (2x – m) = – m = f'(0 – )x 8 0 –f'(x) = lím (–2x) = 0 = f'(0 + )x 8 0 +Para que sea derivable en x = 0, hade ser: – m = 0 8 m = 0Por tanto, f (x) es derivable en Á para m = 0 y n = 5.Unidad 6. Derivadas. Técnicas de derivación7

Página 162EJERCICIOS Y PROBLEMAS PROPUESTOSPARA PRACTICARDefinición de derivada1 Halla la tasa de variación media (T.V.M.) de las siguientes funciones en losintervalos: [–3, –1]; [0, 2]; [2, 5]; [1, 1 + h]:a) f(x) = x 2 + 1 b) f(x) = 7x – 5 c) f(x) = 3 d) f(x) = 2 x¿En cuáles de ellas es constante la T.V.M.? ¿Qué tipo de funciones son?a) f (x) = x 2 + 1En [–3, –1] 8f (–1) – f (–3)T.V.M. = = –42f (2) – f (0)En [0, 2] 8 T.V.M. = = 22f (5) – f (2)En [2, 5] 8 T.V.M. = = 73En [1, 1 + h] 8f (1 + h) – f (1) hT.V.M. = = + 2hhh= h + 2b) f (x) = 7x – 5En [–3, –1] 8f (–1) – f (–3)T.V.M. = = 72f (2) – f (0)En [0, 2] 8 T.V.M. = = 72f (5) – f (2)En [2, 5] 8 T.V.M. = = 73En [1, 1 + h] 8f (1 + h) – f (1) 7hT.V.M. = =hh= 7c) f (x) = 3En [–3, –1] 8f (–1) – f (–3)T.V.M. = = 02f (2) – f (0)En [0, 2] 8 T.V.M. = = 02f (5) – f (2)En [2, 5] 8 T.V.M. = = 03f (1 + h) – f (1)En [1, 1 + h] 8 T.V.M. = = 0h8Unidad 6. Derivadas. Técnicas de derivación

UNIDAD6d) f (x) = 2 xf (–1) – f (–3)En [–3, –1] 8 T.V.M. = =2316En [0, 2] 8f (2) – f (0)T.V.M. = =2En [2, 5] 8f (5) – f (2)T.V.M. = =332283f (1 + h) – f (1) 2En [1, 1 + h] 8 T.V.M. = = 1 + h – 2=hh2(2 h – 1)hLa función b) f (x) = 7x – 5 es una función afín y la T.V.M. es constante.La función c) f (x) = 3 es una función afín y la T.V.M. es 0 (constante).2 Halla la T.V.M. de la función f(x) = –x 2 + 5x – 3 en el intervalo [2, 2 + h] y,con el resultado obtenido, calcula f'(2).f (x) = –x 2 + 5x – 3 en [2, 2 + h]f (2 + h) – f (2) –h= 2 + h= –h + 1hhf'(2) = lím (–h + 1) = 1h 8 03 Utilizando la definición de derivada, calcula f'(3) en las siguientes funciones:3x – 2a) f(x) =7b) f(x) = x 2 – 4c) f(x) = (x – 5) 2d) f(x) =2 + xxf (3 + h) – f (3) (3h/7)a) f'(3) = lím= lím =h 8 0 hh 8 0 h37f (3 + h) – f (3) hb) f'(3) = = 2 + 6hlímlím = 6h 8 0 hh 8 0 hf (3 + h) – f (3) hc) f'(3) = = 2 – 4hlímlím = – 4h 8 0 hh 8 0 hf (3 + h) – f (3)–2hd) f'(3) = lím= lím= –2 h 8 0 hh 8 0 9h + 3h 2 9Unidad 6. Derivadas. Técnicas de derivación9

UNIDAD6—————–1 + h – 1– 2f (–1 + h) – f (–1)–1 + h• f'(–1) = lím= lím=h 8 0 hh 8 0 h–1 + h – 1 + 2 – 2h–h= lím= lím=h 8 0 (–1 + h) h h 8 0 (–1 + h) h–1 –1= lím = = 1h 8 0 –1 + h –1x————+ h – 1–x——– 1f (x + h) – f (x)x +h x• f'(x) = lím= lím=h 8 0 hh 8 0 hx 2 + xh – x – x 2 + x – xh + h(x + h)xh= lím= lím=h 8 0hh 8 0 (x + h) xh1= lím =h 8 0 (x + h) x1x 26 Comprueba, utilizando la definición de derivada, que la función f (x) =no tiene derivada en x = 0.Intentamos hallar f'(0) usando la definición de derivada:f (0 + h) – f (0) √h – 0 √hf'(0) = lím= lím = lím =h 8 0 hh 8 0 h h 8 0 h1 1= lím = = ±@h 8 0 √h 0Por tanto, f (x) = √x no tiene derivada en x = 0.7 Halla la tasa de variación media de la función f (x) = e x en el intervalo[2; 2,001] y comprueba que su valor está muy próximo a e 2 .f (2,001) – f (2) eT.V.M. [2; 2,001] = = 2,001 – e 2› 7,39282,001 – 2 0,001e 2 › 7,3891. Los dos valores están muy próximos.√x° 2x – 3 si x < 28 Dada f (x) = ¢, halla f'(1) y f'(3) utilizando la definición£ x – 1 si x Ó 2de derivada.f (1 + h) – f (1) [2 (1 + h) – 3] – (–1)• f'(1) = lím= lím=h 8 0 hh 8 0 h2 + 2h – 3 + 1 2h= lím= lím = lím 2 = 2h 8 0 hh 8 0 h h 8 0Unidad 6. Derivadas. Técnicas de derivación11

UNIDAD611 Deriva las funciones siguientes:a) y = e 4x (1 – x) 2(x – 1) b) y = c) y = √2 x d) y = ln (2x – 1)a) y' = 4 · e 4x · (x – 1) + e 4x · 1 = e 4x · (4x – 3)–2 · (1 – x) · eb) y' = x – (1 – x) 2 · e x –2 · (1 – x) – (1 – x) –x= 2=2 + 4x – 3e 2xe xe x2 2c) y' = x · ln2=x – 1 · ln22√2 x √2 xd) y' =22x – 1e x12 Deriva estas funciones:a) y = ln (x 2 ln x– 1) b) y = ln √1 – x c) y = d) y = e x2 + 1a) y' =2xx 2 – 1e x–1— 2 √ 1 – x –1b) y' = =√ — 1 – x 2(1 – x)11— · e x – ln x · e x — – ln xxxc) y' = = =e 2x e x1 – x · ln xx · e xd) y' = 2xe x2 +113 Calcula la derivada de estas funciones:a) y = sen x cos 2 sen 2 xx b) y =1 + cos 2 xc) y = sen 2 x 2 d) y = cos 3 (2x + 1)a) y' = cos x cos 2 x – 2cos x sen x sen x = cos 3 x – 2sen 2 x cos x == cos 3 x – 2(1 – cos 2 x) cos x = cos 3 x – 2cos x + 2cos 3 x = 3cos 3 x – 2cos x2sen x cos x (1 + cos 2 x)+ 2cos x sen x sen 2 xb) y' = =(1 + cos 2 x) 22sen x cos x + 2sen x cos 3 x + 2cosx sen 3 x= =(1 + cos 2 x) 22sen x cos x (1 + cos 2 x + sen 2 x)= =(1 + cos 2 x) 24sen x cos x(1 + cos 2 x) 2Unidad 6. Derivadas. Técnicas de derivación13

c) y' = 2x · 2sen x 2 · cos x 2 = 4x sen x 2 cos x 2d) y' = 3cos 2 (2x + 1) · [–sen (2x + 1) · 2] = –6sen (2x + 1) cos 2 (2x + 1)14 Deriva las funciones siguientes:131 + 2xa) y = log 2b) y = √sen x c) y = d) y = √x + √ — x√ 2x1 – 2xa) y = log 21 – log 2x1 1 –1y' = – · =x ln 2 xln 22x · cos xb) y' =23 3 √sen 2 x 2——————2 · (1 – 2x) + (1 + 2x) · 2——4(1 – 2x)c) y' = 2(1 – 2x)22= = =1 + 2x1 + 2x2 ·1 + 2x√——2 · (1 – 2x) 2 ·1 – 2x √—— 1 – 2x √1 – 2x22= =√1 + 2x √(1 – 2x) 3 (1 + 2x)(1 – 2x) 4 · 1 – 2x11 + — 2√ — 2 √ x x + 1d) y' = = =2 · √ — x + √ x 4√ — x · √ — x + √ x2√ — x + 14· √ ——x 2 + x√ — x15 Halla la derivada de:xa) y = √x √ — xb) y = ln√ x + 1x – 1c) y = ln (sen √e ) d) y =√ xx + 1☛ b) Aplica las propiedades de los logaritmos antes de derivar.a) y = = = x 3/4 38 y' = · x –1/4 3√√ x — 2 · x4√x 34 4 · 4 √x1b) y = · (ln x – ln (x + 1))21 1 1 1y' = · – =2 x x +1 2x 2 +2x()c) y = ln (sen e x/2 (1/2) · e x/2 · cos e x/2) 8 y' = = e x/2 · cos √ — e xsen e x/2 2 · sen √ — e x14Unidad 6. Derivadas. Técnicas de derivación

UNIDAD6———x + 1 – x + 1(x +1)d) y' = 211= = =x – 1√2 ·x – 1x – 1(x +1) 2 · √ √——(x + 1) 4x + 1·x + 1x + 11=√(x – 1) · (x +1) 3Página 163Continuidad y derivabilidad16 Estudia la continuidad y la derivabilidad de las siguientes funciones en lospuntos que se indican, y represéntalas:° 3x – 1 si x < 1a) f (x) = ¢en x = 1£ x 2 + x si x Ó 1° –x 2 si x < 0b) f (x) = ¢en x = 0£ x 2 si x Ó 0° 2x – 1 si x < 3c) f (x) = ¢en x = 3£ x 2 – 4 si x Ó 3° 3x – 2 si x Ì 2d) f (x) = ¢en x = 2£ 3x + 1 si x > 2a) Continuidad en x = 1:lím f (x) = lím (3x – 1) = 2x 8 1 – x 8 1 –lím f (x) = lím (x 2 + x) = 2x 8 1 + x 8 1 +f (1) = 2Derivabilidad en x = 1:° 3 si x < 1f'(x) = ¢£ 2x + 1 si x > 1f'(1 – ) = 3f'(1 + ) = 3°¢£f (x) es derivable en x = 1 y f'(1) = 3.°§§¢§£f (x) es continuaen x = 1.Gráfico:10987654321–3–2 –1–11 2 3 4 5–2–3–4–5Unidad 6. Derivadas. Técnicas de derivación15

) Continuidad en x = 0:lím f (x) = lím –x 2 = 0x 8 0 – x 8 0 –lím f (x) = lím x 2 = 0x 8 0 + x 8 0 +f (0) = 0Derivabilidad en x = 0:Derivabilidad en x = 3:° 2 si x < 3f'(x) = ¢£ 2x si x > 3f'(3 – ) = 2f'(3 + ) = 6° –2x si x < 0¢£ 2x si x > 0¢£°¢£°d) Continuidad en x = 2:f (x) no es derivable en x = 3.lím f (x) = lím (3x – 2) = 4 f (x) no es continuax 8 2 – x 8 2 –en x = 2 (tiene unalím f (x) = lím (3x + 1) = 7 discontinuidad dex 8 2 + x 8 2 +salto finito).Derivabilidad en x = 2:Como f (x) no es continua en x = 2, tampoco esderivable en ese punto.°§§¢§§£f (x) es continuaen x = 0.Gráfico:f'(x) =–4–3–2 –1–1–21 2 3 4–3f'(0 – ) = 0f (x) es derivable en x = 0 y f'(0) = 0.f'(0 + ) = 0c) Continuidad en x = 3:lím f (x) = lím (2x – 1) = 5x 8 3 – x 8 3 –f (x) es continualím f (x) = lím (x 2 – 4) = 5x 8 3 + x 8 3 +en x = 3.–4–5Gráfico:f (3) = 5°§¢§§£§°§¢£12111098765432154321–3–2 –1–11 2 3 4 5–2Gráfico:10987654321–3–2 –1–11 2 3 4–2–3–4–516Unidad 6. Derivadas. Técnicas de derivación

£°§§¢§£UNIDAD617 Comprueba que f (x) es continua pero no derivable en x = 2:° ln (x – 1) si x < 2f (x) = ¢£ 3x – 6 si x Ó 2Continuidad en x = 2:lím f (x) = lím ln (x – 1) = ln 1 = 0x 8 2 – x 8 2 –lím f (x) = lím (3x – 6) = 0 f (x) es continua en x = 2.x 8 2 + x 8 2 +f (2) = 0Derivabilidad en x = 2:° 1§ —---- si x < 2f'(x) = ¢ x –1§£ 3 si x > 2f'(2 – ) = 1f'(2 + ) = 3°¢£°§¢§§£Como las derivadas laterales no coinciden, f (x) no es derivableen x = 2.s18Considera la siguiente función:° 0 si x < 0§f (x) = ¢ x 2 si 0 Ì x < 1§£ x si x Ó 1a) Estudia su continuidad.b) Estudia su derivabilidad.a) • Si x ? 0 y x ? 1 8 Es continua, pues está formada por funciones continuas.• En x = 0:lím f (x) = lím 0 = 0x 8 0 – x 8 0lím f (x) = lím x 2 lím f (x) = f (0). Por tanto, la función es= 0x 8 0 +x 8 0x 8 0continua en x = 0.°§§¢§§f (0) = 0• En x = 1:lím f (x) = lím x 2 = 1x 8 1 – x 8 1lím f (x) = lím x = 1x 8 1 + x 8 1f (1) = 1límx 8 1f (x) = f (1). Por tanto, la función escontinua en x = 1.La función es continua en Á.Unidad 6. Derivadas. Técnicas de derivación17

) • Si x ? 0 y x ? 1 8 La función es derivable. Su derivada es, en esos puntos:° 0 si x < 0f'(x) =§¢ 2x si 0 < x < 1§ 1 si x > 1£• En x = 0:f'(0 – ) = 0 = f'(0 + ). Por tanto, f (x) es derivable en x = 0; y f'(0) = 0.• En x = 1:f'(1 – ) = 2 ? f'(1 + ) = 1. Por tanto, f (x) no es derivable en x = 1.La función es derivable en Á – {1}. Su derivada es:° 0 si x < 0f'(x) =§¢ 2x si 0 Ì x < 1§ 1 si x > 1£19 Prueba que la función f (x) =|x + 1| no es derivable en x = –1.° –x – 1 si x Ì –1f (x) = | x +1| = ¢£ x + 1 si x Ó –1x 8 –1 – x 8 –1 –f (x) es una función continua, pues está formada por dos funciones continuas, six ? –1.• En x = –1:lím f(x) = lím (–x – 1) = 0lím f(x) = lím (x + 1) = 0 f es continua en x = –1.x 8 –1 + x 8 –1 +f(–1) = 0• Su derivada, si x ? –1, es:° –1 si x < –1f'(x) = ¢£ 1 si x > –1Las derivadas laterales en x = –1 son:f'(–1 + ) = 1f'(–1 – ) = –1°§§¢§£°¢£No coinciden; por tanto, f (x) no es derivableen x = –1.20 Estudia la continuidad y la derivabilidad de la siguiente función:° x 2 – 1 si x Ì 1f (x) = ¢£ x – 1 si x > 1Continuidad:Si x ? 1 8 f (x) es continua, pues está formada por polinomios, que son funcionescontinuas.18Unidad 6. Derivadas. Técnicas de derivación

°§§¢§£UNIDAD6En x = 1 8 límx 8 1 –límx 8 1 +f (x) = lím (x 2 – 1) = 0x 8 1 –f (x) = lím (x – 1) = 0x 8 1 +f (1) = 0f (x) es continua en x = 1.Por tanto, f (x) es una función continua.Derivabilidad:Si x ? 1: f (x) es derivable y su derivada es:° 2x si x < 1f'(x) = ¢£ 1 six > 1En x = 1: Hallamos las derivadas laterales:f'(1 + ) = 1f'(1 – ) = 2°¢£No coinciden; por tanto, f (x) no es derivable en x = 1.° e –x si x Ì 021 Dada la función f (x) = ¢£ 1 – x si x Ó 0Estudia su continuidad y su derivabilidad.Continuidad:Si x ? 0: f (x) es continua, pues está formada por funciones continuas.En x = 0:lím f (x) = lím e –x = 1x 8 0 – x 8 0 –lím f (x) = lím (1 – x) = 1f (x) es continua en x = 0.x 8 0 + x 8 0 +f (0) = 1Por tanto, f (x) es una función continua.Derivabilidad:Si x ? 0: f (x) es derivable y su derivada es:° –e –x si x < 0f'(x) = ¢£ –1 si x > 0En x = 0: Hallamos las derivadas laterales:f'(0 – ) = –1Coinciden; luego, f (x) es derivable en x = 0.f'(0 + ) = –1Por tanto, f (x) es una función derivable.° –e –x si x < 0Su derivada es f'(x) = ¢£ –1 si x Ó 0°§¢§§£°¢£Unidad 6. Derivadas. Técnicas de derivación19

°¢£°¢£22 ¿En qué puntos no son derivables las funciones siguientes?:a) f(x) = | x 2 – 4| b) f(x) = | 2x – 3|a) f(x) = | x 2 – 4|f (x) es una función continua, pues es la composición de funciones continuas.La definimos a trozos:° x 2 – 4 si x < –2§f (x) = ¢ –x 2 + 4 si –2 Ì x Ì 2§£ x 2 – 4 si x > 2Si x ? –2 y x ? 2, f'(x) es derivable y su derivada es:f'(x) =° 2x si x < –2§¢ –2x si –2 < x < 2§£ 2x si x > 2En x = –2: Hallamos las derivadas laterales:f'(–2 – ) = –4f'(–2 + ) = 4f (x) no es derivable en x = –2.En x = 2: Hallamos las derivadas laterales:f'(2 – ) = –4f'(2 + ) = 4f (x) no es derivable en x = 2.Por tanto, f (x) no es derivable en los puntos (–2, 0) y (2, 0).° –2x + 3 si x < 3/2b) f(x) = | 2x – 3| = ¢£ 2x – 3 si x Ó 3/2f(x) es una función continua pues es la composición de dos funciones continuas(y = 2x – 3 e y = | x | ).3° –2 si x < 3/2En x ? , f(x) es derivable y su derivada es f'(x) = ¢2£ 2 si x > 3/2En x =3, f no es derivable porque f'2()3 – 3 += –2 y f' = 222()PARA RESOLVER° 3x – 1 si x Ì 223 Dada f (x) = ¢:£ x 2 + 1 si x > 2a) Calcula f'(1) y f'(3). b) Comprueba que f'(2 – ) ? f'(2 + ).Si x ? –2: f(x) es una función continua, pues está formada por polinomios, queson funciones continuas.20Unidad 6. Derivadas. Técnicas de derivación

°§§¢§£UNIDAD6En x = 2:lím f (x) = lím (3x – 1) = 5x 8 2 – x 8 2 –lím f (x) = lím (x 2 + 1) = 5x 8 2 + x 8 2 +f (2) = 5f (x) es continua en x = 2.Por tanto, f (x) es una función continua.Si x ? 2: f (x) es derivable y su derivada es:° 3 si x < 2f'(x) = ¢£ 2x si x > 2a) f'(1) = 3; f'(3) = 6b) f'(2 – ) = 3f'(2 + ) = 4°¢£no coinciden24 Esta es la gráfica de una función y = f (x).Calcula, observándola:f'(–1), f'(1) y f'(3)¿En qué puntos no es derivable?2–2 2 4• En x = –1, la recta tangente a f es horizontal; su pendiente es 0. Por tanto,f'(–1) = 0.• En x = 1, f es una función constante. Luego f'(1) = 0.• En x = 3, f es una recta que pasa por los puntos (2, 1) y (4, 5). Calculamos supendiente:5 – 1m = = 2. Por tanto, f'(3) = 2.4 – 2• No es derivable en x = 0 ni en x = 2, porque en ellos observamos que:f'(0 – ) ? f'(0 + ) y f'(2 – ) ? f'(2 + )25 ¿Cuántos puntos que no tengan derivada hay en la función y = |x 2 + 6x + 8|?x 2 –6 ± √36 – 32 –6 ± √4 –6 ± 2 x = –2+ 6x + 8 = 0 8 x = = =22 2 x = –4° x 2 + 6x + 8 si x < –4 ° 2x + 6 si x < –4§§y = ¢ –x 2 – 6x – 8 si –4 Ì x Ì –2 y' = ¢ –2x – 6 si –4 < x < –2§§£ x 2 + 6x + 8 si x > –2 £ 2x + 6 si x > –2La función es continua, pues es el valor absoluto de una función continua.Unidad 6. Derivadas. Técnicas de derivación21

°§§¢§£°¢£°¢£En x = –4 8 y' (– 4 – ) = –2 ? y' (– 4 + ) = 2En x = –2 8 y' (– 2 – ) = –2 ? y' (– 2 + ) = 2La función no es derivable en x = –4 ni en x = –2; es decir, en (–4, 0) y en (–2, 0).Son dos puntos “angulosos”.s26 Calcula a y b para que la siguiente función sea derivable en todo Á:° ax 2 + 3x si x Ì 2f (x) = ¢£ x 2 – bx – 4 si x > 2Para que sea derivable, en primer lugar, ha de ser continua.• Si x ? 2 8 la función es continua, pues está formada por dos polinomios.• En x = 2 debe cumplirse quelímx 8 2f(x) = f(2):límx 8 2 –f (x) = lím (ax 2 + 3x) = 4a + 6lím f (x) = lím (x 2 – bx – 4) = –2bx 8 2 +f (2) = 4a + 6x 8 2x 8 2Para que sea continua, ha de ser 4a + 6 = –2b; es decir, 2a + 3 = –b, o bienb = –2a – 3.Derivabilidad:• Si x ? 2 8 la función es derivable. Además:f'(x) =° 2ax + 3 si x < 2¢£ 2x – b si x > 2• En x = 2 debe cumplirse que f'(2 – ) = f'(2 + ):f'(2 – ) = 4a + 3f'(2 + ) = 4 – bPara que sea derivable, ha de ser 4a + 3 = 4 – b; es decir, b = – 4a +1.Teniendo en cuenta las dos condiciones obtenidas:b = –2a – 3b = – 4a +1–2a – 3 = – 4a + 1 8 2a = 4 8 a = 2b = –7Por tanto, para que f (x) sea derivable en todo Á, ha de ser a = 2 y b = –7.22Unidad 6. Derivadas. Técnicas de derivación

°¢£UNIDAD627 Observa las gráficas de las siguientes funciones e indica en qué puntos noson derivables:a) b) c)112–2 2 –2–2 –222¿Alguna de ellas es derivable en todo Á?a) No es derivable en x = –1 (tiene un punto “anguloso”), ni en x = 2 (no estádefinida la función).b) Es derivable en todo Á.c) No es derivable en x = 0 (tiene un punto “anguloso”).s28Calcula a y b para que f(x) sea continua y derivable:° x 3 – x si x Ì 0f (x) = ¢£ ax + b si x > 0Continuidad:• En x ? 0 8 La función es continua, pues está formada por dos polinomios.• En x = 0:lím f (x) = lím (x 3 – x) = 0x 8 0 – x 8 0límx 8 0 +f (0) = 0f (x) =Derivabilidad:lím (ax + b) = bx 8 0Si x ? 0: 8 La función es derivable. Además:° 3x 2 – 1 si x < 0f'(x) = ¢£ a si x > 0En x = 0:°§§¢§§£Para que sea continua ha de ser b = 0.f'(0 – ) = –1f'(0 + ) = aPara que sea derivable, ha de ser a = –1.Por tanto, f (x) será continua y derivable si a = –1 y b = 0.Unidad 6. Derivadas. Técnicas de derivación23

s29Estudia la continuidad y la derivabilidad de la siguiente función:° e x si x Ì 0§f (x) = ¢ 1 si 0 < x < 3§£ –x 2 + 3x + 2 si x Ó 3x 8 0 – x 8 0Continuidad:Si x ? 0 y x ? 3: es continua, pues está formada por funciones continuas.En x = 0:lím f (x) = lím e x = 1lím f (x) = lím 1 = 1f (x) = f (0). La función es continua en x = 0.x 8 0 +f (0) = 1En x = 3:límx 8 3 –límx 8 3 +f (3) = 2x 8 0f (x) = lím 1 = 1x 8 3f (x) = lím (–x 2 + 3x +2) = 2x 8 3°§¢§£límx 8 0°§¢§£límx 8 3 –f (x) ? lím f (x) = f (0).x 8 3 +No es continua en x = 3.La función es continua en Á –{3}.Derivabilidad:Si x ? 0 y x ? 3, es derivable y:° e x x < 0§f'(x) = ¢ 0 0 < x < 3§£ –2x + 3 x > 3En x = 0:f'(0 – ) = 1 ? f'(0 + ) = 0 8 No es derivable en x = 0.En x = 3: no es derivable, pues no es continua.La función es derivable en Á –{0, 3}.Página 16430 Averigua para qué valores de x es f'(x) = 0 en cada una de las siguientesfunciones:x 2 (3x – 8)a) f (x) = b) f (x) = x 4 + 2x1221c) f (x) = d) f (x) = e x (x – 1)x 2 + 124Unidad 6. Derivadas. Técnicas de derivación

UNIDAD63xa) f (x) = 3 – 8x 28 f'(x) =129x 2 – 16x122 c) f'(x) =–2x(x 2 + 1)x = 0f'(x) = 0 8 9x 2 – 16x = 0 8 x (9x – 16) = 016x = —— 9b) f'(x) = 4x 3 + 4x = 4x (x 2 + 1)f'(x) = 0 8 4x (x 2 + 1) = 0 8 x = 0f'(x) = 0 8 –2x = 0 8 x = 0d) f'(x) = e x (x – 1) + e x · 1 = e x (x – 1 + 1) = e x xf'(x) = 0 8 x = 031 Halla los puntos en los que la pendiente de la recta tangente es igual a 0 encada una de las siguientes funciones:x 2 +1x 3a) f (x) = b) f (x) =x 2 – 1x 2 – 12x 2 – 3xx 2 +1c) f (x) = d) f (x) =2 – xxDebemos hallar los puntos en los que f'(x) = 0 en cada caso:2x (xa) f'(x) = 2 – 1) – (x 2 + 1) · 2x 2x= 3 –2x –2x 3 – 2x –4x=(x 2 – 1) 2 (x 2 – 1) 2 (x 2 – 1) 2f'(x) = 0 8 –4x = 0 8 x = 0 8 y = –1 8 Punto (0, –1)3x 3x xb) f'(x) = 2 (x 2 – 1) – x 3 · 2x= 4 – 3x 2 –2x 4=4 – 3x 2(x 2 – 1) 2 (x 2 – 1) 2 (x 2 – 1) 2f'(x) = 0 8 x 4 – 3x 2 = 0 8 x 2 (x 2 – 3) = 0x = 0 8 (0, 0)x = –√ — 3 8 –√ — –3√3( 3,2 )x 2 – 3 = 0x = √ — 3 8 √ — 3√3( 3,2 )(4x – 3) (2 – x) – (2x 8x –4xc) f'(x) = 2 –3x) · (–1)= 2 – 6 + 3x + 2x 2 – 3x=(2 – x) 2 (2 – x) 2= –2x2 +8x – 6(2 – x) 2Unidad 6. Derivadas. Técnicas de derivación25

f'(x) = 0 8 –2x 2 + 8x – 6 = 0 8 x 2 – 4x + 3 = 0–4 ± √16 – 12 4 ± √4 4 ± 2 x = 1 8 (1, –1)x = = = =22 2x = 3 8 (3, –9)2x · x – (x 2x xd) f'(x) = 2 + 1) · 1= 2 – x 2 –1=2 –1x 2 x 2 x 2f'(x) = 0 8 x 2 – 1 = 0x = –1 8 (–1, –2)x = 1 8 (1, 2)32 Averigua si en las siguientes funciones existen puntos en los que f'(x) = 0:2x – 36xa) f (x) = b) f (x) =x + 1x 2 + 1c) f (x) = ln (x + 1) d) f (x) = 10 – (x – 2) 4a) f'(x) =2(x + 1) – (2x – 3) · 1=2x + 2 – 2x + 3=5(x + 1) 2 (x + 1) 2 (x + 1) 2f'(x) ? 0 para cualquier valor de x.6(x 6x –6xb) f'(x) = 2 + 1) – 6x · 2x= 2 + 6 – 12x 2=2 + 6(x 2 + 1) 2 (x 2 + 1) 2 (x 2 + 1) 2f'(x) = 0 8 –6x 2 + 6 = 0 8 x 2 = 1x = –1 8 (–1, –3)x = 1 8 (1, 3)1c) f'(x) = ? 0 para cualquier valor de x.x + 1d) f'(x) = –4 (x – 2) 3f'(x) = 0 8 x = 2 8 (2, 10)33 Las siguientes funciones tienen algún punto donde la derivada no existe.Hállalos en cada caso:3a) f (x) = √x 2b) f (x) = √x + 2c) f (x) = √x 2 – 1d) f (x) =|x – 3|||4x – 5e) f (x) = f) f (x) =|x 2 – 2x|2a) f (x) = x 2/3 2; Dom f = Á 8 f'(x) = x –1/3 =323 3 √xf'(x) no existe si x = 0; es decir, f (x) no es derivable en x = 0.26Unidad 6. Derivadas. Técnicas de derivación

°¢£°¢£°¢£°¢£UNIDAD61b) f'(x) =2√x + 2f'(x) no existe si x = –2; el dominio de f (x) es [–2, +@).Por tanto, en los puntos en los que la función está definida, no es derivable enx = –2.c) El dominio de la función es (–@, –1] « [1, +@).f'(x) =2x=x2√x 2 – 1 √x 2 – 1En los puntos en los que f (x) está definida, no es derivable en x = –1, ni enx = 1.° –x + 3 si x < 3d) f (x) = ¢; f'(x) =£ x – 3 si x Ó 3°¢£–1 si x < 31 si x > 3f (x) es continua en Á; pero no es derivable en x = 3, pues sus derivadaslaterales no coinciden:f'(3 – ) = –1f'(3 + ) = 1Son distintas.° –4x + 5 5§— si x < —2 4e) f (x) = ¢f'(x) =§4x – 5 5— si x Ó —£ 2 4–2 si x < 5/42 si x > 5/4f (x) es continua en Á; pero no es derivable en x =laterales no coinciden:°¢£5, pues sus derivadas4f'(5/4 – ) = –2f'(5/4 + ) = 2Son distintas.° x 2 – 2x si x < 0° 2x – 2 si x < 0§§f) f (x) = ¢ –x 2 + 2x si 0 Ì x Ì 2 f'(x) = ¢ –2x + 2 si 0 < x < 2§§£ x 2 – 2x si x > 2£ 2x – 2 si x > 2f (x) es continua en Á; pero no es derivable en x = 0, ni en x = 2, pues susderivadas laterales no coinciden:f'(0 – ) = –2f'(0 + ) = 2Son distintas.f'(2 – ) = –2f'(2 + ) = 2Son distintas.Unidad 6. Derivadas. Técnicas de derivación27

°¢£°¢°¢££34 Esta es la gráfica de una función y = f (x).Estudia su continuidad y su derivabilidad.f (x) es continua en Á –{3}. En x = 3, presenta unadiscontinuidad de salto finito.2–2 2 4f (x) es derivable en Á –{0, 3}. En x = 0 hay un punto anguloso (las derivadaslaterales no coinciden), en x = 3 no es continua, por tanto, no puede ser derivable.35 Considera esta función:° x 2 – 5x + m si x Ì 1f (x) = ¢£ –x 2 + nx si x > 1Calcula m y n para que f sea derivable en todo Á.Continuidad:• Si x ? 1: f (x) es continua, pues está formada por polinomios, que son funcionescontinuas.• En x = 1:lím f (x) = lím (x 2 – 5x + m) = –4 + mx 8 1 – x 8 1 –lím f (x) = lím (–x 2 + nx) = –1 + nx 8 1 + x 8 1 +f (1) = –4 + mPara que f (x) sea continua en x = 1, ha de ser – 4 + m = –1 + n.°§¢§£Derivabilidad:• Si x ? 1: f (x) es derivable y su derivada es:f'(x) =° 2x – 5 si x < 1¢£ –2x + n si x > 1• En x = 1: Para que f (x) sea derivable en x = 1, las derivadas laterales hande coincidir, es decir:f'(1 – ) = –3f'(1 + ) = –2 + n–3 = –2 + nUniendo las dos condiciones anteriores tenemos que:–4 + m = –1 + n–3 = –2 + nm = n + 3n = –1m = 2n = –128Unidad 6. Derivadas. Técnicas de derivación

UNIDAD636 Estudia la continuidad y la derivabilidad de la función:° x 2 + 2x – 1 si x Ì 1f (x) = ¢£ x + 1 si x > 1¿Existe algún punto en el que f'(x) = 0?Represéntala gráficamente.Continuidad:• En x ? 1: La función es continua, pues está formada por dos polinomios.• En x = 1:lím f (x) = lím (x 2 + 2x – 1) = 2x 8 1 – x 8 1lím f (x) = f (1).x 8 1lím f (x) = lím (x + 1) = 2x 8 1 + x 8 1Por tanto, la función escontinua en x = 1.f (1) = 2La función es continua en todo Á.Derivabilidad:• Si x ? 1: La función es derivable. Además:° 2x + 2 si x < 1f'(x) = ¢£ 1 si x > 1• En x = 1:f'(1 – ) = 4 ? f'(1 + ) = 1La función no es derivable en x = 1.Por tanto, la función es derivable en Á – {1}.Puntos en los que f'(x) = 0:f'(x) = 2x + 2 si x < 12x + 2 = 0 8 x = –1f'(x) = 1 si x > 1 8 f'(x) ? 0 si x > 1Por tanto, la derivada se anula en x = –1.Gráfica de f (x):°§¢§£1–1 1–1Unidad 6. Derivadas. Técnicas de derivación29

37 Halla a y b para que la función f (x) sea continua:° 2x + a si x < –1§f (x) = ¢ ax + b si –1 Ì x < 0§£ 3x 2 + 2 si 0 Ì xPara los valores de a y b obtenidos, estudia la derivabilidad de f.• Si x ? –1 y x ? 0: La función es continua, pues está formada por polinomios.• En x = –1:lím f (x) = lím (2x + a) = –2 + ax 8 –1 – x 8 –1Para que sea continua, ha de serlím f (x) = lím (ax + b) = –a + b –2 + a = –a + b, es decir:x 8 –1 + x 8 –1b = 2a – 2f (–1) = –a + b• En x = 0:lím f (x) = lím (ax + b) = bx 8 0 – x 8 0lím f (x) = lím (3x 2 + 2) = 2x 8 0 + x 8 0f (0) = 2Por tanto, f (x) será continua si a = 2 y b = 2.Para estos valores, queda:f (x) =° 2x + 2 si x < –1§¢ 2x + 2 si –1 Ì x < 0; es decir:§£ 3x 2 + 2 si 0 Ì x°§¢§£°§¢§£Para que sea continua, ha de ser b = 2.f (x) =° 2x + 2 si x < 0¢£ 3x 2 + 2 si x Ó 0Derivabilidad:• Si x ? 0: Es derivable. Además:f'(x) =° 2 si x < 0¢£ 6x si x > 0• En x = 0:f'(0 – ) = 2 ? f'(0 + ) = 0La función no es derivable en x = 0.Por tanto, es derivable en Á – {0}.30Unidad 6. Derivadas. Técnicas de derivación

UNIDAD638 Calcula f'(0), siendo:ef (x) = lnx + e√ –x2x +1☛ Aplica las propiedades de los logaritmos antes de derivar.Hallamos f'(x) y después sustituimos en x = 0.1f (x) = [ln (e x + e –x ) – ln (2x + 1)]21f'(x) =e[ x – e –x–22 e 2x + 1 ]x + e –x1f'(0) = · (–2) = –1239 Halla la pendiente de la recta tangente a las siguientes curvas en los puntosindicados:a) y = sen x cos x en x =b) y = x ln x en x = ex 2e xc) y = en x = 0 y x = 1d) y = e x2 – 1 en x = 1π4Debemos hallar la derivada en los puntos indicados en cada caso:a) y' = cosx · cosx + senx (–senx) = cos 2 x – sen 2 xπ4√22√22y' ( ) = ( ) 2 – ( ) 2 = 01b) y' = 1 · ln x + x · = ln x + 1; y' (e) = ln e + 1 = 1 + 1 = 2x2xe 2x – xc) y' = x – x 2 · e x e= x (2x – x 2 )=2(e x ) 2 (e x ) 2 e xy' (0) = 0; y' (1) =1ed) y' = 2xe x2 – 1 ; y' (1) = 2Unidad 6. Derivadas. Técnicas de derivación31

40 Estas gráficas representan las funciones derivadas de las funciones f, g, h y j:–222f'2–22g'2h'2224j'a) ¿Cuáles de estas funciones tienen puntos de tangente horizontal?b) ¿Cuál de estas gráficas es la función derivada de una función polinómicade primer grado?c) ¿Cuál de ellas corresponde a una función polinómica de segundo grado?a) Los puntos de tangente horizontal son los puntos en los que se anula la derivada.f tiene un punto de tangente horizontal en x = –2, pues f'(–2) = 0.j tiene dos puntos de tangente horizontal en x = 1 y en x = 3, puesj'(1) = j'(3) = 0.g y h no tienen ningún punto de tangente horizontal.b) La derivada de una función polinómica de primer grado es una función constante.Por tanto, es g'.c) La derivada de una función polinómica de segundo grado es una función polinómicade primer grado. Por tanto, es f'.Página 165CUESTIONES TEÓRICASs41Una función polinómica de tercer grado, ¿cuántos puntos de derivada nulapuede tener?¿Puede tener uno o ninguno?La derivada de una función polinómica de tercer grado es una función polinómicade segundo grado.Por tanto, puede haber dos puntos, un punto, o ningún punto, con derivada nula.Por ejemplo:f (x) = x 3 – 3x 8 f'(x) = 3x 2 x = 1– 3 = 0 Dos puntosx = –1f (x) = x 3 8 f'(x) = 3x 2 = 0 8 x = 0 8 Un puntof (x) = x 3 + 3x 8 f'(x) = 3x 2 + 3 ? 0 para todo x 8 Ninguno¢°£32Unidad 6. Derivadas. Técnicas de derivación

UNIDAD6s42Justifica que una función polinómica de segundo grado tiene siempre unpunto de tangente horizontal.Su derivada es una función polinómica de primer grado, que se anula siempre enun punto.43 Si una función tiene un punto anguloso en x = a, ¿qué podemos decir def'(a)?f'(a) no existe.44 Sea f una función de la que sabemos que:f'(2 – f(2 + h) – f(2)) = = –1 f'(2 + f(2 + h) – f(2)lím) = lím= 1h 8 0 – hh 8 0 + h¿Es f derivable en x =2?No, pues las derivadas laterales no coinciden.45 La función f (x) = √x – 3 es continua en x =3 y f'(3) = +@. ¿Cómo es larecta tangente a f en x =3?Es una recta vertical.46 Prueba que la función f (x) = x + |x – 3| no es derivable en x = 3.° x – x + 3 si x < 3f (x) = ¢=£ x + x – 3 si x Ó 3¢°£° 3 si x < 3¢£ 2x – 3 si x Ó 3f'(x) =° 0 si x < 3¢£ 2 si x > 3f'(3 – ) = 0 ? f'(3 + ) = 2. Por tanto, la función no es derivable en x = 3.PARA PROFUNDIZAR47 ¿Cuáles de estas gráficasrepresentan la funciónf y su derivada f'?Justifica tu respuesta.a) b)2222c)d)2222Unidad 6. Derivadas. Técnicas de derivación33

°§¢§£a) La función en rojo es una recta que tiene pendiente 3. Por tanto, su derivada esy = 3 (la recta verde).Luego estas gráficas sí representan a una función y su derivada.b) La función en rojo es un polinomio de 2.° grado, una parábola. Su derivada esuna recta. En x = 0, la función tiene un máximo; la derivada se anula. Paraque la recta fuera la derivada, tendría que pasar por (0, 0).No representan, por tanto, a una función y su derivada.c) La función tiene que ser un polinomio de 3. er grado porque tiene dos extremosrelativos. Su derivada será un polinomio de 2.° grado, una parábola. En x = 1,la función tiene un máximo; la derivada se anula, f'(1) = 0, y tendría quepasar por (1, 0).Estas tampoco representan a una función y su derivada.d) La función en rojo es una recta de pendiente 0. Por tanto, su derivada es y = 0,la recta en verde.En este caso, las gráficas representan a una función y su derivada.48 La función f (x) = x 3 + ax 2 + bx + c verifica que f (1) = 1, f'(1) = 0 yf''(1) = 0. Calcula a, b y c.f'(x) = 3x 2 + 2ax + b; f''(x) = 6x + 2af (1) = 1 8 1 + a + b + c = 1f'(1) = 0 8 3 + 2a + b = 0f''(1) = 0 8 6 + 2a = 0Por tanto: f (x) = x 3 – 3x 2 + 3xa = –3b = 3c = 02(x – 1) – 2x · 1 2x – 2 – 2xf'(x) = = =(x – 1) 2 (x – 1) 2 (x – 1) 2492xHalla los puntos de la función y =x – 1en los que la pendiente de la rectatangente es igual a –2.Buscamos los puntos en los que f'(x) = –2:f'(x) = –2 8–2= –2 8 –2 = –2(x – 1) 2(x – 1) 2 = 1 8 x 2 – 2x + 1 = 1 8 x 2 – 2x = 0 8 x (x – 2) = 0x = 0 8 (0, 0)x = 2 8 (2, 4)(x – 1) 2 –234Unidad 6. Derivadas. Técnicas de derivación

UNIDAD6Página 165AUTOEVALUACIÓN1. Halla la función derivada de cada una de las siguientes funciones:5xa) y = 3x √2x + 1b) y = c) y =√x(x +2) 2d) y =(1 – x1 + x2e) y = e 2x +1 xf) y = ln +132 3(2x + 1) + 3x 9x +3a) y' = 3 √2x + 1 + 3x = =2√ — 2x +1 √ — 2x +1 √ — 2x +11–5 · — 2√ x –5b) y' =x=2x √ — x(x +2) 2 – x · 2(x +2) x + 2 – 2x –x +2c) y' = = =(x + 2) 4 (x + 2) 3 (x + 2) 31 – x)–1(1 + x) – (1 – x) 1 – x)–2d) y' = 2 ( = 2 ( =1 + x (1 + x) 2 1 + x (1 + x) 2e) y' = 2e2x +1)—13 1 x +3f) y' = = : =x 3 3— + 131x + 3–4(1 – x)(1 + x) 3()2. Aplica la definición de derivada para hallar f'(2) siendo f(x) = x 2 – 5x.f'(2) =límh 8 0f(2 + h) – f(2)h• f(2 + h) = (2 + h) 2 – 5(2 + h) = h 2 – h – 6• f(2) = 2 2 – 5 · 2 = –6• f(2 + h) – f(2) = h 2 – hf(2 + h) – f(2) h 2 – h• = = h – 1hhf'(2) = lím (h – 1) = –1h 8 0Unidad 6. Derivadas. Técnicas de derivación35

3. Estudia la continuidad y la derivabilidad de esta función:° x 2 + 2x – 1 si x Ì 1§f(x) = ¢ 4§— si x > 1£ x +1¿Existe algún punto en el que f'(x) = 0?f(x) es continua si x < 1 y si x > 1, porque las funciones que la definen lo son.Estudiamos la continuidad en x = 1.límx 8 1f(x)límx 8 1 –límx 8 1 +f(x) = lím (x 2 + 2x – 1) = 2x 8 1 –lím 4f(x) = x 8 1 + = 2x +1f(1) = 1 + 2 – 1 = 2Como lím f(x) = f(1) = 2, f es continua en x = 1.x 8 1Por tanto, f es continua en Á.° 2x + 2 si x < 1§Hallamos f'(x) = ¢ –4§— si x > 1£ (x +1) 2f es derivable si x < 1 y si x > 1.Estudiamos su derivabilidad en x = 1.f'(1 – ) = 2 · 1 + 2 = 4–4Como f'(1 – ) ? f'(1 + ), no existe f'(1).f'(1 + ) = — = –1(1 + 1) 2f es derivable en Á – {1}.Veamos si f'(x) = 0 tiene solución:2x + 2 = 0 8 x = –1–4= 0 no tiene solución.(x +1) 2Por tanto, f'(x) = 0 cuando x = –1.¢§°£§4. Calcula a y b para que la siguiente función sea derivable:° ax + b si x < 0f(x) = ¢£ x 2 – 3x + 2 si x Ó 0Representa la función para los valores de a y b que has hallado.Para que f sea derivable en x = 0, debe ser continua en ese punto.36Unidad 6. Derivadas. Técnicas de derivación

°§¢§£°¢£°¢£UNIDAD6límx 8 0f(x)lím (ax + b) = bx 8 0 –lím (x 2 – 3x + 2) = 2x 8 0 +Para que lím f(x) = f(0) = 2, debe ser b = 2.x 8 0Si b = 2, f es continua en Á.f'(x) =° a si x < 0¢£ 2x – 3 si x > 0Veamos si f es derivable en x = 0:f'(0 – ) = af'(0 + ) = –3Para que exista f'(0), debe ser a = –3.YSi a = –3, f es derivable en Á.f(x) =£°¢° –3x + 2 si x < 0¢£ x 2 – 3x + 2 si x Ó 011X5. ¿En qué puntos no es derivable la función f(x) = |x 2 – 4x + 3|? Justifica turespuesta.Definimos la función por intervalos. Para ello, hacemos:x 2 – 4x + 3 = 0 8 x =4 ± √16 – 122x = 1x = 3° x 2 – 4x + 3 si x Ì 1§f(x) = ¢ –x 2 + 4x – 3 si 1 < x < 3§£ x 2 – 4x + 3 si x Ó 3Hallamos f'(x):° 2x – 4 si x < 1§f'(x) = ¢ –2x + 4 si 1 < x < 3§£ 2x – 4 si x > 3Estudiamos la derivabilidad de f en x = 1 y en x = 3:f'(1 – ) = 2 · 1 – 4 = –2f'(1 + ) = –2 · 1 + 4 = 2Como f'(1 – ) ? f'(1 + ), no existe f'(1).f'(3 – ) = –2 · 3 + 4 = –2f'(3 + ) = 2 · 3 – 4 = 2Como f'(3 – ) ? f'(3 + ), no existe f'(3).f no es derivable ni en x = 1, ni en x = 3.Unidad 6. Derivadas. Técnicas de derivación37

6. Observando la gráfica de esta función f, estudia su derivabilidad. Halla, siexisten, f'(–4), f'(0), f'(3).22• f es discontinua en x = 1. Por tanto, no es derivable en x = 1.En x = –2 observamos que f'(–2 – ) ? f'(–2 + ): tampoco es derivable.Luego f es derivable en Á – {–2, 1}.• f'(–4) = 0 porque en ese punto la función es constante.f'(0) = 0 porque en x = 0 la tangente es horizontal.f'(3) = –1 porque –1 es la pendiente de la recta que pasa por (1, 2) y (3, 0):2 – 0m = = –11 – 338Unidad 6. Derivadas. Técnicas de derivación