1 Dadas las matrices A, B y C. Comprobar que AB = AC. A
1 Dadas las matrices A, B y C. Comprobar que AB = AC. A
1 Dadas las matrices A, B y C. Comprobar que AB = AC. A
Create successful ePaper yourself
Turn your PDF publications into a flip-book with our unique Google optimized e-Paper software.
Geometría Vectorial.<br />
Primera Relación de Problemas<br />
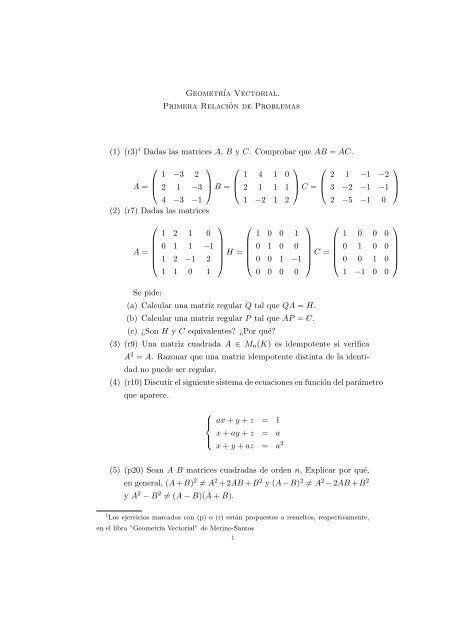
(1) (r3) 1 <strong>Dadas</strong> <strong>las</strong> <strong>matrices</strong> A, B y C. <strong>Comprobar</strong> <strong>que</strong> <strong>AB</strong> = <strong>AC</strong>.<br />
A =<br />
⎛<br />
⎜<br />
⎝<br />
1 −3 2<br />
2 1 −3<br />
4 −3 −1<br />
(2) (r7) <strong>Dadas</strong> <strong>las</strong> <strong>matrices</strong><br />
⎞<br />
⎛<br />
⎟ ⎜<br />
⎠ B = ⎝<br />
1 4 1 0<br />
2 1 1 1<br />
1 −2 1 2<br />
⎞<br />
⎛<br />
⎟ ⎜<br />
⎠ C = ⎝<br />
2 1 −1 −2<br />
3 −2 −1 −1<br />
2 −5 −1 0<br />
⎛<br />
⎞ ⎛<br />
⎞ ⎛<br />
⎞<br />
1 2 1 0<br />
1 0 0 1<br />
1 0 0 0<br />
A =<br />
0 1 1 −1<br />
⎜<br />
⎝ 1 2 −1 2<br />
⎟<br />
⎠ H = 0 1 0 0<br />
⎜<br />
⎝ 0 0 1 −1<br />
⎟<br />
⎠ C = 0 1 0 0<br />
⎜<br />
⎝ 0 0 1 0<br />
⎟<br />
⎠<br />
1 1 0 1<br />
0 0 0 0<br />
1 −1 0 0<br />
Se pide:<br />
(a) Calcular una matriz regular Q tal <strong>que</strong> QA = H.<br />
(b) Calcular una matriz regular P tal <strong>que</strong> AP = C.<br />
(c) ¿Son H y C equivalentes? ¿Por qué?<br />
(3) (r9) Una matriz cuadrada A ∈ M n (K) es idempotente si verifica<br />
A 2 = A. Razonar <strong>que</strong> una matriz idempotente distinta de la identidad<br />
no puede ser regular.<br />
(4) (r10) Discutir el siguiente sistema de ecuaciones en función del parámetro<br />
<strong>que</strong> aparece.<br />
⎧<br />
⎪⎨<br />
⎪⎩<br />
ax + y + z = 1<br />
x + ay + z = a<br />
x + y + az = a 2<br />
⎞<br />
⎟<br />
⎠<br />
(5) (p20) Sean A B <strong>matrices</strong> cuadradas de orden n, Explicar por qué,<br />
en general, (A + B) 2 ≠ A 2 + 2<strong>AB</strong> + B 2 y (A − B) 2 ≠ A 2 − 2<strong>AB</strong> + B 2<br />
y A 2 − B 2 ≠ (A − B)(A + B).<br />
1 Los ejercicios marcados con (p) o (r) están propuestos o resueltos, respectivamente,<br />
en el libro ”Geometría Vectorial” de Merino-Santos<br />
1
(6) (p21) 2 Una matriz se dice idempotente si A 2 = A. Demostrar <strong>que</strong><br />
la siguiente matriz es idempotente.<br />
A =<br />
⎛<br />
⎜<br />
⎝<br />
2 −2 −4<br />
−1 3 4<br />
1 −2 −3<br />
⎞<br />
⎟<br />
⎠<br />
(7) (p22) Probar <strong>que</strong> si A es una matriz idempotente, entonces B = I−A<br />
también lo es y <strong>que</strong> <strong>AB</strong> = BA = 0.<br />
(8) (p23) Demostrar <strong>que</strong> la matriz<br />
( )<br />
2 1<br />
A =<br />
1 2<br />
verifica una ecuación del tipo A 2 + αA + βI = 0, determinando α<br />
y β. Utilizar este resultado para calcular la inversa de A.<br />
(9) (p25) Probar <strong>que</strong> la suma de <strong>matrices</strong> simétricas es simétrica. Demostrar<br />
<strong>que</strong> el producto no lo es en general.<br />
2 Los ejercicios marcados con (p) o (r) están propuestos o resueltos, respectivamente,<br />
en el libro ”Geometría Vectorial” de Merino-Santos<br />
2