Tensors: Geometry and Applications J.M. Landsberg - Texas A&M ...

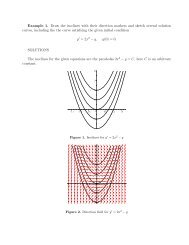
2.1. Rust removal exercises 29
This is sometimes referred to as the fundamental theorem of linear
algebra. It implies rank(f) = rank(f T ), i.e., that for a matrix, row
rank equals column rank, as was already seen in Exercise (4) above.
(7) Let V denote the vector space of 2 × 2 matrices. Take a basis
� � � � � � � �
1 0 0 1 0 0 0 0
v1 = , v2 = , v3 = , v4 =
0 0 0 0 1 0 0 1
Fix a,b,c,d ∈ C and let
A =
� �
a b
.
c d
Write out a 4 × 4 matrix expressing the linear map
LA : V → V
X ↦→ AX
that corresponds to left multiplication by A. Write the analogous
matrix for right multiplication. For which matrices A are the two
induced linear maps the same?
(8) Given a 2 × 2 matrix A, write out a 4 × 4 matrix expressing the
linear map
ad(A) : V → V
X ↦→ AX − XA.
What is the largest possible rank of this linear map?
(9) Let A be a 3 × 3 matrix and write out a 9 × 9 matrix representing
the linear map LA : Mat3×3 → Mat3×3.
(10) Choose new bases such that the matrices of Exercises 7 and 9 become
block diagonal (i.e., the only non-zero entries occur in 2 × 2
(resp. 3 × 3) blocks centered about the diagonal). What will the
n × n case look like?
(11) A vector space V admits a direct sum decomposition V = U ⊕
W if U,W ⊂ V are linear subspaces and if for all v ∈ V there
exists unique u ∈ U and w ∈ W such that v = u + w. Show
that that a necessary and sufficient condition to have a direct sum
decomposition V = U ⊕ W is that dim U + dim W ≥ dim V and
U ∩W = (0). Similarly, show that another necessary and sufficient
condition to have a direct sum decomposition V = U ⊕ W is that
dim U + dim W ≤ dim V and span{U,W } = V .
(12) Let S 2 C n denote the vector space of symmetric n × n matrices.
Calculate dim S 2 C n . Let Λ 2 C n denote the vector space of skew