Implicit-Explicit Runge-Kutta schemes for hyperbolic systems ... - utenti

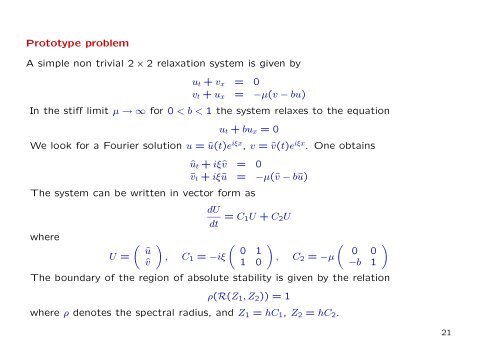
Prototype problem
A simple non trivial 2 × 2 relaxation system is given by
ut + vx = 0
vt + ux = −µ(v − bu)
In the stiff limit µ → ∞ for 0 < b < 1 the system relaxes to the equation
ut + bux = 0
We look for a Fourier solution u = û(t)e iξx , v = ˆv(t)e iξx . One obtains
ût + iξˆv = 0
ˆvt + iξû = −µ(ˆv − bû)
The system can be written in vector form as
where
U =
� û
ˆv
�
, C1 = −iξ
dU
dt = C1U + C2U
� 0 1
1 0
�
, C2 = −µ
� 0 0
−b 1
The boundary of the region of absolute stability is given by the relation
ρ(R(Z1, Z2)) = 1
where ρ denotes the spectral radius, and Z1 = hC1, Z2 = hC2.
�
21