Implicit-Explicit Runge-Kutta schemes for hyperbolic systems ... - utenti

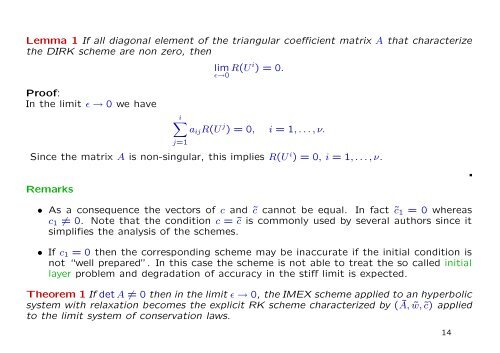
Lemma 1 If all diagonal element of the triangular coefficient matrix A that characterize
the DIRK scheme are non zero, then
Proof:
In the limit ɛ → 0 we have
lim
ɛ→0 R(U i ) = 0.
i�
aijR(U j ) = 0, i = 1, . . . , ν.
j=1
Since the matrix A is non-singular, this implies R(U i ) = 0, i = 1, . . . , ν.
Remarks
• As a consequence the vectors of c and ˜c cannot be equal. In fact ˜c1 = 0 whereas
c1 �= 0. Note that the condition c = ˜c is commonly used by several authors since it
simplifies the analysis of the schemes.
• If c1 = 0 then the corresponding scheme may be inaccurate if the initial condition is
not “well prepared”. In this case the scheme is not able to treat the so called initial
layer problem and degradation of accuracy in the stiff limit is expected.
Theorem 1 If det A �= 0 then in the limit ɛ → 0, the IMEX scheme applied to an hyperbolic
system with relaxation becomes the explicit RK scheme characterized by (Ã, ˜w, ˜c) applied
to the limit system of conservation laws.
14