Simplicial Structures in Topology

More documents

Recommendations

Info

II.4 Simplicial Homology 77 such that k : K → K ′ and ℓ: L → L ′ is the restriction of k to L. The reader can easily verify that the relative homology determines a covariant functor H(−,−;Z): CCsim −→ Ab Z . The next result, which is an immediate application of the Long Exact Sequence Theorem (II.3.1), is called Long Exact Homology Sequence Theorem; it relates the homology groups of L, K, and(K,L) to each other. (II.4.1) Theorem. Let (K,L) be a pair of simplicial complexes. For every n > 0, there is a homomorphism λn : Hn(K,L;Z) → Hn−1(L;Z) (connecting homomorphism) that causes the following sequence of homology groups ...→ Hn(L;Z) Hn(i) −→ Hn(K;Z) q∗(n) −→ Hn(K,L;Z) λn −→ Hn−1(L;Z) → ... , to be exact; here, Hn(i) is the homomorphism induced by the inclusion i: L → K and q∗(n) is the homomorphism induced by the quotient homomorphism qn : Cn(K) → Cn(K)/Cn(L). Proof. For every n > 0, let qn : Cn(K) → Cn(K)/Cn(L) be the quotient homomorphism. With the given definitions, it is easily proved that and therefore, (∀n ≥ 0) ∂ K,L n qn = qn−1∂ K n q = {qn}: C(K) → C(K,L) is a homomorphism of chain complexes. We note furthermore that for each n ≥ 0, the sequence of Abelian groups Cn(L) �� Cn(i) �� Cn(K) qn �� Cn(K)/Cn(L) is a short exact sequence and therefore, we have a short exact sequence of chain complexes C(L) � C(i) � �� C(K) q �� C(K,L); the result follows from Theorem (II.3.1). � The exact sequence of homology groups described in the statement of Theorem (II.4.1) is the exact homology sequence of the pair (K,L).
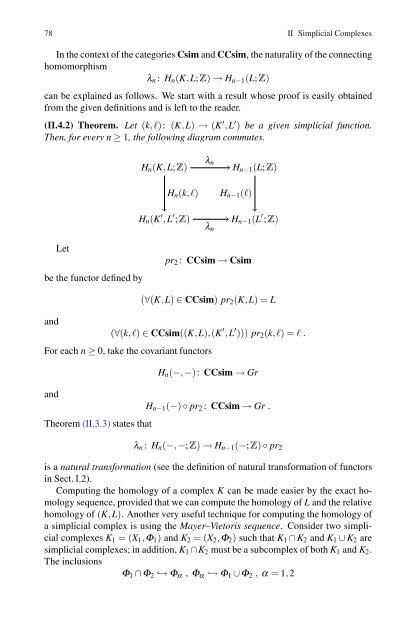
78 II Simplicial Complexes In the context of the categories Csim and CCsim, the naturality of the connecting homomorphism λn : Hn(K,L;Z) → Hn−1(L;Z) can be explained as follows. We start with a result whose proof is easily obtained from the given definitions and is left to the reader. (II.4.2) Theorem. Let (k,ℓ): (K,L) → (K ′ ,L ′ ) be a given simplicial function. Then, for every n ≥ 1, the following diagram commutes. Let be the functor defined by Hn(K,L;Z) Hn(k,ℓ) �� Hn(K ′ ,L ′ ;Z) λn �� λn Hn−1(L;Z) Hn−1(ℓ) �� Hn−1(L ′ ;Z) pr2 : CCsim → Csim (∀(K,L) ∈ CCsim) pr2(K,L)=L and (∀(k,ℓ) ∈ CCsim((K,L),(K ′ ,L ′ ))) pr2(k,ℓ)=ℓ. For each n ≥ 0, take the covariant functors Hn(−,−): CCsim → Gr and Hn−1(−) ◦ pr2 : CCsim → Gr . Theorem (II.3.3) states that λn : Hn(−,−;Z) → Hn−1(−;Z) ◦ pr2 is a natural transformation (see the definition of natural transformation of functors in Sect. I.2). Computing the homology of a complex K can be made easier by the exact homology sequence, provided that we can compute the homology of L and the relative homology of (K,L). Another very useful technique for computing the homology of a simplicial complex is using the Mayer–Vietoris sequence. Consider two simplicial complexes K1 =(X1,Φ1) and K2 =(X2,Φ2) such that K1 ∩ K2 and K1 ∪ K2 are simplicial complexes; in addition, K1 ∩K2 must be a subcomplex of both K1 and K2. The inclusions Φ1 ∩ Φ2 ↩→ Φα , Φα ↩→ Φ1 ∪ Φ2 , α = 1,2
Page 2:
Canadian Mathematical Society Soci
Page 5 and 6:
Dr. Davide L. Ferrario Università
Page 8:
Foreword to the English Edition Exc
Page 11 and 12:
x Preface same qualitative properti
Page 13 and 14:
xii Preface homology groups, also w
Page 16 and 17:
Contents I Fundamental Concepts ...
Page 18 and 19:
Chapter I Fundamental Concepts I.1
Page 20 and 21:
I.1 Topology 3 We need to show that
Page 22 and 23:
I.1 Topology 5 Let X be a topologic
Page 24 and 25:
I.1 Topology 7 (x, y) (x, −y) Fig
Page 26 and 27:
I.1 Topology 9 that f is a homeomor
Page 28 and 29:
I.1 Topology 11 We recall that an i
Page 30 and 31:
I.1 Topology 13 Cx = � Xj j∈J i
Page 32 and 33:
I.1 Topology 15 A Fig. I.5 Example
Page 34 and 35:
I.1 Topology 17 with the topology i
Page 36 and 37:
I.1 Topology 19 are closed in Y. Th
Page 38 and 39:
I.1 Topology 21 is the diagonal of
Page 40 and 41:
I.1 Topology 23 (I.1.38) Lemma. Let
Page 42 and 43:
I.1 Topology 25 Here is an example.
Page 44 and 45: I.2 Categories 27 5. Prove that a f
Page 46 and 47: I.2 Categories 29 3. For every pair
Page 48 and 49: I.2 Categories 31 If conditions 2.
Page 50 and 51: I.2 Categories 33 Conversely, given
Page 52 and 53: I.2 Categories 35 q: B ⊔C → B
Page 54 and 55: I.2 Categories 37 (I.2.5) Lemma. Gi
Page 56 and 57: I.3 Group Actions 39 I.3 Group Acti
Page 58: I.3 Group Actions 41 S2 = {(x,y,z)
Page 61 and 62: 44 II Simplicial Complexes (0, 1) (
Page 63 and 64: 46 II Simplicial Complexes II.2 Abs
Page 65 and 66: 48 II Simplicial Complexes II.2.1 T
Page 67 and 68: 50 II Simplicial Complexes Let us r
Page 69 and 70: 52 II Simplicial Complexes r = tp+(
Page 71 and 72: 54 II Simplicial Complexes In a sim
Page 73 and 74: 56 II Simplicial Complexes (not nec
Page 75 and 76: 58 II Simplicial Complexes Let K3,3
Page 77 and 78: 60 II Simplicial Complexes ordering
Page 79 and 80: 62 II Simplicial Complexes are two
Page 81 and 82: 64 II Simplicial Complexes 1-simple
Page 83 and 84: 66 II Simplicial Complexes An infin
Page 85 and 86: 68 II Simplicial Complexes 2. λn i
Page 87 and 88: 70 II Simplicial Complexes Please n
Page 89 and 90: 72 II Simplicial Complexes (II.3.7)
Page 91 and 92: 74 II Simplicial Complexes (II.3.10
Page 93: 76 II Simplicial Complexes If we do
Page 97 and 98: 80 II Simplicial Complexes Φi = {
Page 99 and 100: 82 II Simplicial Complexes obtained
Page 101 and 102: 84 II Simplicial Complexes (that is
Page 103 and 104: 86 II Simplicial Complexes Therefor
Page 105 and 106: 88 II Simplicial Complexes Exercise
Page 107 and 108: 90 II Simplicial Complexes Hn(C;G)=
Page 109 and 110: 92 II Simplicial Complexes Since im
Page 111 and 112: 94 II Simplicial Complexes and cons
Page 113 and 114: 96 II Simplicial Complexes In parti
Page 116 and 117: Chapter III Homology of Polyhedra I
Page 118 and 119: III.1 The Category of Polyhedra 101
Page 126 and 127: III.2 Homology of Polyhedra 109 Now
Page 128 and 129: III.2 Homology of Polyhedra 111 whe
Page 130 and 131: III.2 Homology of Polyhedra 113 A s
Page 132 and 133: III.2 Homology of Polyhedra 115 Fro
Page 134 and 135: III.3 Some Applications 117 where H
Page 136 and 137: III.3 Some Applications 119 we now
Page 138 and 139: III.3 Some Applications 121 has no
Page 140 and 141: III.3 Some Applications 123 Proof.
Page 142 and 143: III.4 Relative Homology 125 6. Let
Page 144 and 145:
III.4 Relative Homology 127 such th
Page 146 and 147:
III.5 Real Projective Spaces 129 We
Page 148 and 149:
III.5 Real Projective Spaces 131 0
Page 150 and 151:
III.5 Real Projective Spaces 133 No
Page 152 and 153:
III.5 Real Projective Spaces 135 Le
Page 154 and 155:
III.5 Real Projective Spaces 137 he
Page 156 and 157:
III.5 Real Projective Spaces 139 We
Page 158 and 159:
III.6 Homology of the Product of Tw
Page 160 and 161:
Page 162 and 163:
Page 164 and 165:
Page 166:
Page 169 and 170:
152 IV Cohomology (IV.1.1) Theorem.
Page 171 and 172:
154 IV Cohomology (IV.1.2) Remark.
Page 173 and 174:
156 IV Cohomology If, starting from
Page 175 and 176:
158 IV Cohomology When we apply thi
Page 177 and 178:
160 IV Cohomology groups Q and R ar
Page 179 and 180:
162 IV Cohomology (IV.2.2) Corollar
Page 181 and 182:
164 IV Cohomology Since g and π r
Page 183 and 184:
166 IV Cohomology It is easily prov
Page 185 and 186:
168 IV Cohomology ∩: B p (K;Z) ×
Page 188 and 189:
Chapter V Triangulable Manifolds V.
Page 190 and 191:
V.1 Topological Manifolds 173 is a
Page 192 and 193:
V.1 Topological Manifolds 175 |Ki|
Page 194 and 195:
V.2 Closed Surfaces 177 we define C
Page 196 and 197:
V.2 Closed Surfaces 179 Fig. V.6 Co
Page 198 and 199:
V.2 Closed Surfaces 181 a a b a a d
Page 200 and 201:
V.2 Closed Surfaces 183 where i = 1
Page 202 and 203:
V.2 Closed Surfaces 185 Before proc
Page 204 and 205:
V.2 Closed Surfaces 187 Simplicial
Page 206 and 207:
V.3 Poincaré Duality 189 to the co
Page 208 and 209:
V.3 Poincaré Duality 191 (V.3.3) T
Page 210:
V.3 Poincaré Duality 193 therefore
Page 213 and 214:
196 VI Homotopy Groups and define t
Page 215 and 216:
198 VI Homotopy Groups is a homotop
Page 217 and 218:
200 VI Homotopy Groups Proof. First
Page 219 and 220:
202 VI Homotopy Groups (VI.1.10) Ex
Page 221 and 222:
204 VI Homotopy Groups (VI.1.13) Th
Page 223 and 224:
206 VI Homotopy Groups to the simpl
Page 225 and 226:
208 VI Homotopy Groups Proof. For e
Page 227 and 228:
210 VI Homotopy Groups Exercises 1.
Page 229 and 230:
212 VI Homotopy Groups Proof. Since
Page 231 and 232:
214 VI Homotopy Groups is precisely
Page 233 and 234:
216 VI Homotopy Groups The group π
Page 235 and 236:
218 VI Homotopy Groups 4. R ′ α
Page 237 and 238:
220 VI Homotopy Groups and � y0 H
Page 239 and 240:
222 VI Homotopy Groups Let f := F(
Page 241 and 242:
224 VI Homotopy Groups be two homot
Page 243 and 244:
226 VI Homotopy Groups (VI.3.16) Re
Page 245 and 246:
228 VI Homotopy Groups where iA is
Page 247 and 248:
230 VI Homotopy Groups f : I n g: I
Page 249 and 250:
232 VI Homotopy Groups 8. Prove tha
Page 251 and 252:
234 VI Homotopy Groups This derives
Page 253 and 254:
236 VI Homotopy Groups Proof. The f
Page 256 and 257:
References 1. J.W. Alexander - A pr
Page 258 and 259:
Index H-space, 219 n-simple, 225 ab
Page 260:
Index 243 Euclidean, 43 join, 47 su
show all

Simplicial Structures in Topology

Create successful ePaper yourself

Delete template?

Save as template?