Simplicial Structures in Topology

II.3 Homological Algebra 71
ε ′ f0∂1(x1)= f ε∂1(x1)=0
and im∂ ′ 1 = kerε′ , there exists y ′ 1 ∈ C′ 1 such that ∂ ′ 1 (y′ 1 )= f0∂1(x1). This defines a
homomorphism f1 : C1 → C ′ 1 such that f0∂1 = ∂ ′ 1 f1.
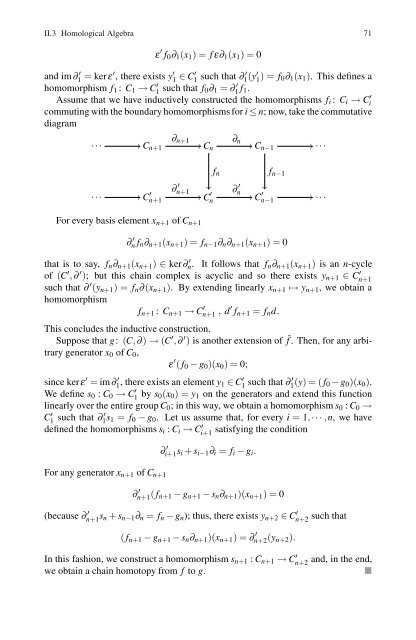
Assume that we have inductively constructed the homomorphisms fi : Ci → C ′ i
commuting with the boundary homomorphisms for i ≤ n; now, take the commutative
diagram
···
···
��
Cn+1
��
′
C n+1
∂n+1 ��
Cn
��
∂ ′ n+1 ��
′
C n
For every basis element xn+1 of Cn+1
fn
∂n ��
Cn−1
��
∂ ′ n ��
′
C n−1
fn−1
∂ ′ n fn∂n+1(xn+1)= fn−1∂n∂n+1(xn+1)=0
��
···
��
···
that is to say, fn∂n+1(xn+1) ∈ ker∂ ′ n. It follows that fn∂n+1(xn+1) is an n-cycle
of (C ′ ,∂ ′ ); but this chain complex is acyclic and so there exists yn+1 ∈ C ′ n+1
such that ∂ ′ (yn+1) = fn∂(xn+1). By extending linearly xn+1 ↦→ yn+1, we obtain a
homomorphism
fn+1 : Cn+1 → C ′ n+1 , d′ fn+1 = fnd.
This concludes the inductive construction.
Suppose that g: (C,∂) → (C ′ ,∂ ′ ) is another extension of ¯f . Then, for any arbitrary
generator x0 of C0,
ε ′ ( f0 − g0)(x0)=0;
since kerε ′ = im∂ ′ 1 , there exists an element y1 ∈ C ′ 1 such that ∂ ′ 1 (y)=(f0 − g0)(x0).
We define s0 : C0 → C ′ 1 by s0(x0) =y1 on the generators and extend this function
linearly over the entire group C0; in this way, we obtain a homomorphism s0 : C0 →
C ′ 1 such that ∂ ′ 1s1 = f0 − g0. Let us assume that, for every i = 1,···,n, wehave
defined the homomorphisms si : Ci → C ′ i+1 satisfying the condition
For any generator xn+1 of Cn+1
∂ ′
i+1 si + si−1∂i = fi − gi.
∂ ′ n+1 ( fn+1 − gn+1 − sn∂n+1)(xn+1)=0
(because ∂ ′ n+1sn + sn−1∂n = fn − gn); thus, there exists yn+2 ∈ C ′ n+2 such that
( fn+1 − gn+1 − sn∂n+1)(xn+1)=∂ ′ n+2(yn+2).
In this fashion, we construct a homomorphism sn+1 : Cn+1 → C ′ n+2 and, in the end,
we obtain a chain homotopy from f to g. �