Simplicial Structures in Topology

I.2 Categories 35
q: B ⊔C → B ⊔ f ,g C; finally, give B ⊔ f ,g C the quotient topology relative to the surjection
q and define the maps
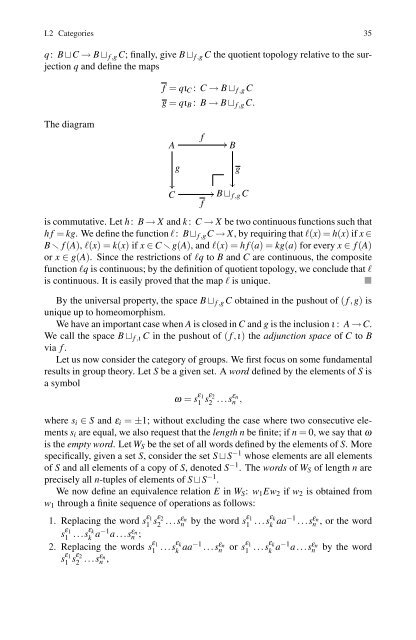
The diagram
f = qιC : C → B ⊔ f ,g C
g = qιB : B → B ⊔ f ,g C.
A
��
C
g
f
f
��
B
g
��
��
B ⊔ f ,g C
is commutative. Let h: B → X and k : C → X be two continuous functions such that
hf = kg. We define the function ℓ: B⊔f,gC → X, by requiring that ℓ(x)=h(x) if x ∈
B � f (A), ℓ(x)=k(x) if x ∈ C � g(A),andℓ(x)=hf(a)=kg(a) for every x ∈ f (A)
or x ∈ g(A). Since the restrictions of ℓq to B and C are continuous, the composite
function ℓq is continuous; by the definition of quotient topology, we conclude that ℓ
is continuous. It is easily proved that the map ℓ is unique. �
By the universal property, the space B ⊔ f ,g C obtained in the pushout of ( f ,g) is
unique up to homeomorphism.
We have an important case when A is closed in C and g is the inclusion ι : A → C.
We call the space B ⊔ f ,ι C in the pushout of ( f ,ι) the adjunction space of C to B
via f .
Let us now consider the category of groups. We first focus on some fundamental
results in group theory. Let S beagivenset.Aword defined by the elements of S is
a symbol
ω = s ε1
1 sε2
2 ...sεn
n ,
where si ∈ S and εi = ±1; without excluding the case where two consecutive elements
si are equal, we also request that the length n be finite; if n = 0, we say that ω
is the empty word. LetWS be the set of all words defined by the elements of S. More
specifically, given a set S, consider the set S ⊔ S −1 whose elements are all elements
of S and all elements of a copy of S, denoted S −1 .Thewords of WS of length n are
precisely all n-tuples of elements of S ⊔ S −1 .
We now define an equivalence relation E in WS: w1Ew2 if w2 is obtained from
w1 through a finite sequence of operations as follows:
1. Replacing the word s ε1
1 sε2
2 ...sεn n by the word s ε1
1 ...sε k
k aa−1 ...sεn n ,ortheword
s ε1
1 ...sε k
k a−1a...sεn n ;
2. Replacing the words s ε1
1 ...sε k
k aa−1 ...sεn n or s ε1
1 ...sε k
k a−1a...sεn n by the word
s ε1
1 sε2
2 ...sεn n ,