Simplicial Structures in Topology

24 I Fundamental Concepts
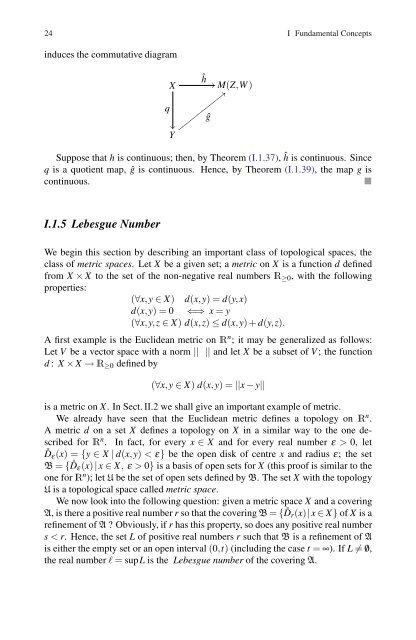
induces the commutative diagram
ˆh
X ��
M(Z,W )
��
��
��
q ��
��
ˆg
��
��
��
Y
Suppose that h is continuous; then, by Theorem (I.1.37), ˆh is continuous. Since
q is a quotient map, ˆg is continuous. Hence, by Theorem (I.1.39), themapg is
continuous. �
I.1.5 Lebesgue Number
We begin this section by describing an important class of topological spaces, the
class of metric spaces. LetX be a given set; a metric on X is a function d defined
from X × X to the set of the non-negative real numbers R≥0, with the following
properties:
(∀x,y ∈ X) d(x,y)=d(y,x)
d(x,y)=0 ⇐⇒ x = y
(∀x,y,z ∈ X) d(x,z) ≤ d(x,y)+d(y,z).
A first example is the Euclidean metric on R n ; it may be generalized as follows:
Let V be a vector space with a norm || || and let X be a subset of V; the function
d : X × X → R≥0 defined by
(∀x,y ∈ X) d(x,y)=||x − y||
is a metric on X. In Sect. II.2 we shall give an important example of metric.
We already have seen that the Euclidean metric defines a topology on R n .
Ametricd on a set X defines a topology on X in a similar way to the one described
for R n . In fact, for every x ∈ X and for every real number ε > 0, let
˚Dε(x) ={y ∈ X | d(x,y) < ε} be the open disk of centre x and radius ε; theset
B = { ˚Dε(x) | x ∈ X, ε > 0} is a basis of open sets for X (this proof is similar to the
one for R n ); let U be the set of open sets defined by B. ThesetX with the topology
U is a topological space called metric space.
We now look into the following question: given a metric space X and a covering
A, is there a positive real number r so that the covering B = { ˚Dr(x)|x ∈ X} of X is a
refinement of A ? Obviously, if r has this property, so does any positive real number
s < r. Hence, the set L of positive real numbers r such that B is a refinement of A
is either the empty set or an open interval (0,t) (including the case t = ∞). If L �= /0,
the real number ℓ = supL is the Lebesgue number of the covering A.