Simplicial Structures in Topology
Simplicial Structures in Topology
Simplicial Structures in Topology
You also want an ePaper? Increase the reach of your titles
YUMPU automatically turns print PDFs into web optimized ePapers that Google loves.
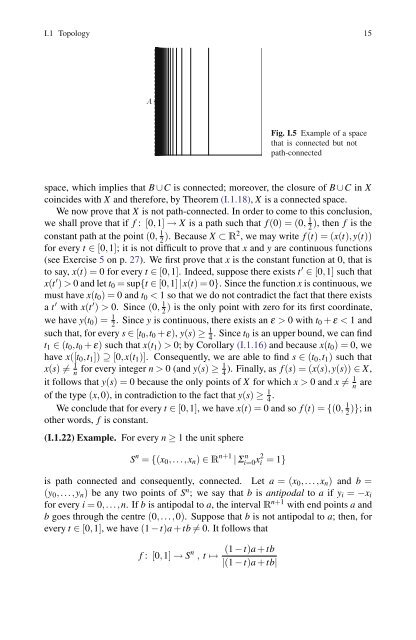
I.1 <strong>Topology</strong> 15<br />
A<br />
Fig. I.5 Example of a space<br />
that is connected but not<br />
path-connected<br />
space, which implies that B ∪C is connected; moreover, the closure of B ∪C <strong>in</strong> X<br />
co<strong>in</strong>cides with X and therefore, by Theorem (I.1.18), X is a connected space.<br />
We now prove that X is not path-connected. In order to come to this conclusion,<br />
we shall prove that if f : [0,1] → X isapathsuchthatf (0)=(0, 1 2 ),thenfis the<br />
constant path at the po<strong>in</strong>t (0, 1<br />
2 ). Because X ⊂ R2 , we may write f (t)=(x(t),y(t))<br />
for every t ∈ [0,1]; it is not difficult to prove that x and y are cont<strong>in</strong>uous functions<br />
(see Exercise 5 on p. 27). We first prove that x is the constant function at 0, that is<br />
to say, x(t)=0foreveryt∈ [0,1]. Indeed, suppose there exists t ′ ∈ [0,1] such that<br />
x(t ′ ) > 0andlett0 = sup{t ∈ [0,1]|x(t)=0}. S<strong>in</strong>ce the function x is cont<strong>in</strong>uous, we<br />
must have x(t0)=0andt0 < 1 so that we do not contradict the fact that there exists<br />
a t ′ with x(t ′ ) > 0. S<strong>in</strong>ce (0, 1 2 ) is the only po<strong>in</strong>t with zero for its first coord<strong>in</strong>ate,<br />
we have y(t0)= 1 2 .S<strong>in</strong>ceyiscont<strong>in</strong>uous, there exists an ε > 0 with t0 + ε < 1and<br />
such that, for every s ∈ [t0,t0 + ε), y(s) ≥ 1 4 .S<strong>in</strong>cet0isan upper bound, we can f<strong>in</strong>d<br />
t1 ∈ (t0,t0 + ε) such that x(t1) > 0; by Corollary (I.1.16) and because x(t0)=0, we<br />
have x([t0,t1]) ⊇ [0,x(t1)]. Consequently, we are able to f<strong>in</strong>d s ∈ (t0,t1) such that<br />
x(s) �= 1<br />
1<br />
n for every <strong>in</strong>teger n > 0(andy(s) ≥ 4 ). F<strong>in</strong>ally, as f (s)=(x(s),y(s)) ∈ X,<br />
it follows that y(s)=0 because the only po<strong>in</strong>ts of X for which x > 0andx �= 1 n are<br />
of the type (x,0), <strong>in</strong> contradiction to the fact that y(s) ≥ 1<br />
4 .<br />
We conclude that for every t ∈ [0,1],wehavex(t)=0andsof (t)={(0, 1<br />
2 )};<strong>in</strong><br />
other words, f is constant.<br />
(I.1.22) Example. For every n ≥ 1 the unit sphere<br />
S n = {(x0,...,xn) ∈ R n+1 | Σ n i=0x 2 i = 1}<br />
is path connected and consequently, connected. Let a =(x0,...,xn) and b =<br />
(y0,...,yn) be any two po<strong>in</strong>ts of S n ; we say that b is antipodal to a if yi = −xi<br />
for every i = 0,...,n. Ifb is antipodal to a, the <strong>in</strong>terval R n+1 with end po<strong>in</strong>ts a and<br />
b goes through the centre (0,...,0). Suppose that b is not antipodal to a; then, for<br />
every t ∈ [0,1],wehave(1 −t)a +tb�= 0. It follows that<br />
f : [0,1] → S n , t ↦→<br />
(1 −t)a + tb<br />
|(1 −t)a + tb|