Simplicial Structures in Topology

VI.1 Fundamental Group 209
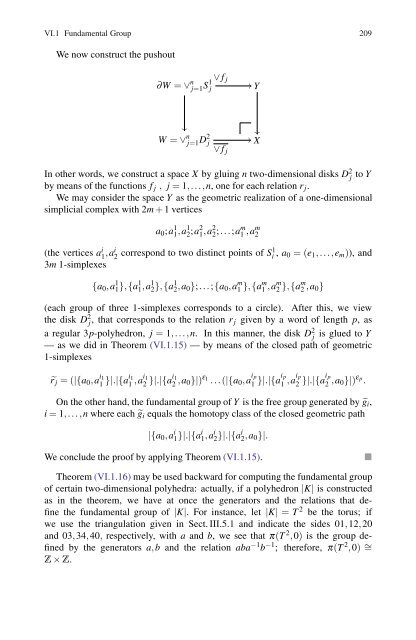
We now construct the pushout
∂W = ∨ n j=1S1 ∨ f j
j
��
W = ∨ n j=1 D2 j ∨ f j
In other words, we construct a space X by gluing n two-dimensional disks D2 j to Y
by means of the functions f j , j = 1,...,n, one for each relation r j.
We may consider the space Y as the geometric realization of a one-dimensional
simplicial complex with 2m + 1 vertices
��
Y
��
��
X
a0;a 1 1 ,a1 2 ;a2 1 ,a2 2 ;...;am 1 ,am 2
(the vertices a i 1 ,ai 2 correspond to two distinct points of S1 i , a0 =(e1,...,em)), and
3m 1-simplexes
{a0,a 1 1},{a 1 1,a 1 2},{a 1 2,a0};...;{a0,a m 1 },{am 1 ,am 2 },{am 2 ,a0}
(each group of three 1-simplexes corresponds to a circle). After this, we view
the disk D2 j , that corresponds to the relation r j given by a word of length p, as
a regular 3p-polyhedron, j = 1,...,n. In this manner, the disk D2 j is glued to Y
— as we did in Theorem (VI.1.15) — by means of the closed path of geometric
1-simplexes
�r j =(|{a0,a i1
1 }|.|{ai1
1 ,ai1
2 }|.|{ai1
2 ,a0}|) ε1 ...(|{a0,a ip
1 }|.|{aip
1 ,aip
2 }|.|{aip
2 ,a0}|) εp .
On the other hand, the fundamental group of Y is the free group generated by �gi,
i = 1,...,n where each �gi equals the homotopy class of the closed geometric path
|{a0,a i 1 }|.|{ai 1 ,ai 2 }|.|{ai 2 ,a0}|.
We conclude the proof by applying Theorem (VI.1.15). �
Theorem (VI.1.16) may be used backward for computing the fundamental group
of certain two-dimensional polyhedra: actually, if a polyhedron |K| is constructed
as in the theorem, we have at once the generators and the relations that define
the fundamental group of |K|. For instance, let |K| = T 2 be the torus; if
we use the triangulation given in Sect. III.5.1 and indicate the sides 01,12,20
and 03,34,40, respectively, with a and b, we see that π(T 2 ,0) is the group defined
by the generators a,b and the relation aba −1 b −1 ; therefore, π(T 2 ,0) ∼ =
Z × Z.