Simplicial Structures in Topology

204 VI Homotopy Groups
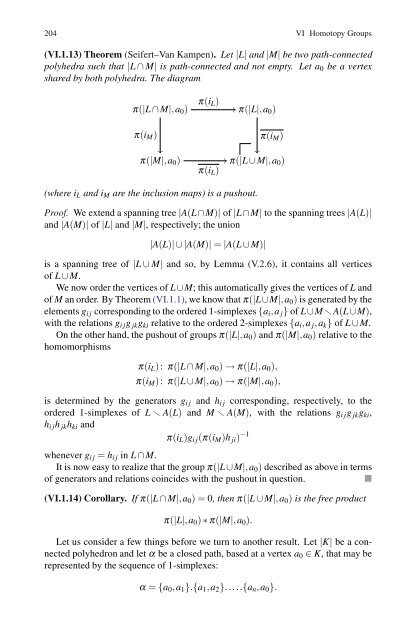
(VI.1.13) Theorem (Seifert–Van Kampen). Let |L| and |M| be two path-connected
polyhedra such that |L ∩ M| is path-connected and not empty. Let a0 be a vertex
shared by both polyhedra. The diagram
π(|L ∩ M|,a0) π(iL)
π(iM)
��
π(|M|,a0)
π(iL)
��
π(|L|,a0)
π(iM)
��
��
π(|L ∪ M|,a0)
(where iL and iM are the inclusion maps) is a pushout.
Proof. We extend a spanning tree |A(L∩M)| of |L∩M| to the spanning trees |A(L)|
and |A(M)| of |L| and |M|, respectively; the union
|A(L)|∪|A(M)| = |A(L ∪ M)|
is a spanning tree of |L ∪ M| and so, by Lemma (V.2.6), it contains all vertices
of L ∪ M.
We now order the vertices of L∪M; this automatically gives the vertices of L and
of M an order. By Theorem (VI.1.1), we know that π(|L∪M|,a0) is generated by the
elements gij corresponding to the ordered 1-simplexes {ai,aj} of L∪M �A(L∪M),
with the relations gijg jkgki relative to the ordered 2-simplexes {ai,a j,ak} of L ∪ M.
On the other hand, the pushout of groups π(|L|,a0) and π(|M|,a0) relative to the
homomorphisms
π(iL): π(|L ∩ M|,a0) → π(|L|,a0),
π(iM): π(|L ∪ M|,a0) → π(|M|,a0),
is determined by the generators gij and hij corresponding, respectively, to the
ordered 1-simplexes of L � A(L) and M � A(M), with the relations gijg jkgki,
hijh jkhki and
π(iL)gij(π(iM)h ji) −1
whenever gij = hij in L ∩ M.
It is now easy to realize that the group π(|L∪M|,a0) described as above in terms
of generators and relations coincides with the pushout in question. �
(VI.1.14) Corollary. If π(|L ∩ M|,a0)=0,thenπ(|L ∪ M|,a0) is the free product
π(|L|,a0) ∗ π(|M|,a0).
Let us consider a few things before we turn to another result. Let |K| be a connected
polyhedron and let α be a closed path, based at a vertex a0 ∈ K, that may be
represented by the sequence of 1-simplexes:
α = {a0,a1}.{a1,a2}.....{an,a0}.