Simplicial Structures in Topology

V.2 Closed Surfaces 183
where i = 1,...,n (we note that the generators β(a) i 1 and β(b)i1 are cyclic – see the
definition of block homology); similarly, the simplicial complex e2 is given an orientation
by choosing a generator β2. The chain groupC1(e(nT 2 )) is the free Abelian
group generated by β(a) i 1 and β(b)i 1 , i = 1,...,n; on the other hand, d2(β2) =0,
since the 1-blocks appear twice, and in opposite directions. Therefore,
H1(nT 2 ;Z) ∼ = Z 2n
(see Theorem (III.5.9)). In the case of nRP2 , we give the 1-blocks ei 1 an orientation
by choosing a generator β i 1 for each H1(ei 1 , • ei 1 ;Z), and a generator
β2 ∈ H2(e2, • e2;Z) ∼ = Z .
In this case, we have d2(β2)=2(∑ n i=1 β i 1 ); then, C1(e(nRP2 )) is the Abelian group
generated by β 1 1 ,β2 1 ,∑ n i=1 β i 1 and since 2(∑ni=1 β i 1 ) is a boundary,
,...,β n−1
1
H1(nRP 2 ;Z) ∼ = Z n−1 × Z2 . �
The connected sum enables us to join two surfaces, but there are other similar
constructions. Let us consider the cylinder I × S 1 and two immersions of the disk
h1,h2 : D 2 → S in the surface S in such a way that D1 = h1(D 2 ) and D2 = h2(D 2 )
are disjoint. The space
S ′ = S � (IntD1 ∪ IntD2) ⊔h (I × S 1 ),
obtained by joining S � (IntD1 ∪ IntD2) and the cylinder I × S 1 through the map
h: {0,1}×S 1 → S � (IntD1 ∪ IntD2),
defined by h(0,t)=h1(t) and h(1,t)=h2(t) for every t ∈ S 1 ⊂ D 2 , is still a surface.
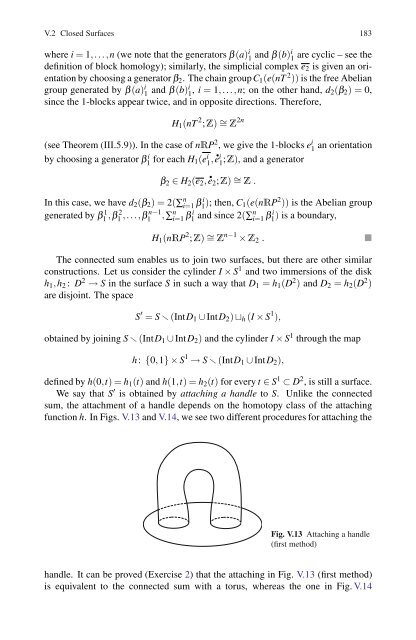
We say that S ′ is obtained by attaching a handle to S. Unlike the connected
sum, the attachment of a handle depends on the homotopy class of the attaching
function h. InFigs.V.13 and V.14, we see two different procedures for attaching the
Fig. V.13 Attaching a handle
(first method)
handle. It can be proved (Exercise 2) that the attaching in Fig. V.13 (first method)
is equivalent to the connected sum with a torus, whereas the one in Fig. V.14