Simplicial Structures in Topology

138 III Homology of Polyhedra
III.5.2 Homology of RP n , with n ≥ 4
We first wish to find a convenient triangulation for RP n . We remind the reader that
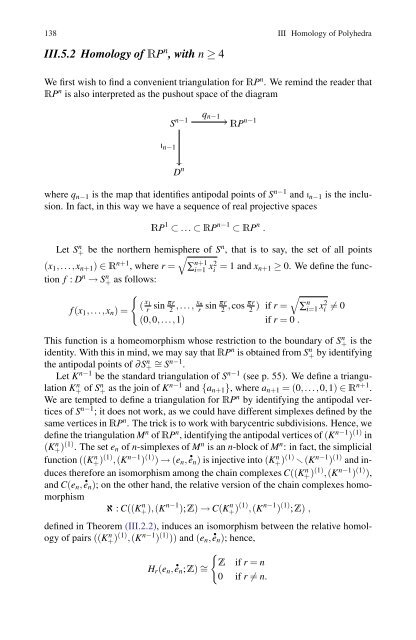
RP n is also interpreted as the pushout space of the diagram
ın−1
S n−1
��
D n
qn−1 ��
RP n−1
where qn−1 is the map that identifies antipodal points of S n−1 and ın−1 is the inclusion.
In fact, in this way we have a sequence of real projective spaces
RP 1 ⊂ ...⊂ RP n−1 ⊂ RP n .
Let Sn + be the northern hemisphere of Sn , that is to say, the set of all points
(x1,...,xn+1) ∈ Rn+1 �
,wherer = ∑ n+1
i=1 x2 i = 1andxn+1≥0. We define the function
f : Dn → Sn + as follows:
�
(
f (x1,...,xn)=
x1
�
πr xn πr πr
r sin 2 ,..., r sin 2 ,cos 2 ) if r = ∑ n i=1 x2 i �= 0
(0,0,...,1) if r = 0 .
This function is a homeomorphism whose restriction to the boundary of S n + is the
identity. With this in mind, we may say that RPn is obtained from Sn + by identifying
the antipodal points of ∂Sn + ∼ = Sn−1 .
Let Kn−1 be the standard triangulation of Sn−1 (see p. 55). We define a triangulation
Kn + of Sn + as the join of Kn−1 and {an+1}, wherean+1 =(0,...,0,1) ∈ Rn+1 .
We are tempted to define a triangulation for RPn by identifying the antipodal vertices
of Sn−1 ; it does not work, as we could have different simplexes defined by the
same vertices in RPn . The trick is to work with barycentric subdivisions. Hence, we
define the triangulation Mn of RPn , identifying the antipodal vertices of (Kn−1 ) (1) in
(Kn + )(1) .Thesetenof n-simplexes of Mn is an n-block of Mn : in fact, the simplicial
function ((Kn + )(1) ,(K n−1 ) (1) ) → (en, • en) is injective into (Kn + )(1) �(K n−1 ) (1) and induces
therefore an isomorphism among the chain complexes C((Kn + )(1) ,(K n−1 ) (1) ),
and C(en, • en); on the other hand, the relative version of the chain complexes homomorphism
ℵ : C((K n + ),(Kn−1 );Z) → C(K n + )(1) ,(K n−1 ) (1) ;Z) ,
defined in Theorem (III.2.2), induces an isomorphism between the relative homology
of pairs ((K n + )(1) ,(K n−1 ) (1) )) and (en, • en); hence,
Hr(en, • en;Z) ∼ =
�
Z if r = n
0 ifr �= n.