Simplicial Structures in Topology

128 III Homology of Polyhedra
III.5 Homology of Real Projective Spaces
We have already computed the homology groups of some elementary polyhedra
such as the sphere S 2 and the torus T 2 (see also exercises in Sect. II.4). We have
also studied techniques that, in theory, allow us to study the homology of polyhedra
(for instance, the Exact Homology Sequence Theorem). In this section, we compute
the homology of real projective spaces; we shall see that the methods learned so far
are not enough to complete our pre-established task and for this reason we shall
provide a new method for computing homology groups.
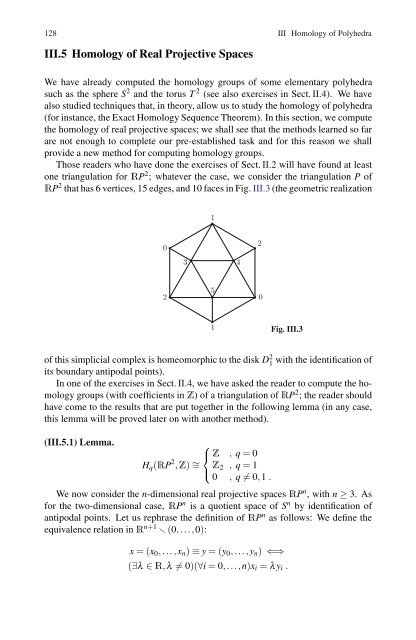
Those readers who have done the exercises of Sect. II.2 will have found at least
one triangulation for RP 2 ; whatever the case, we consider the triangulation P of
RP 2 that has 6 vertices, 15 edges, and 10 faces in Fig. III.3 (the geometric realization
0
2
1
3 4
5
1
2
0
Fig. III.3
of this simplicial complex is homeomorphic to the disk D2 1 with the identification of
its boundary antipodal points).
In one of the exercises in Sect. II.4, we have asked the reader to compute the homology
groups (with coefficients in Z) of a triangulation of RP2 ; the reader should
have come to the results that are put together in the following lemma (in any case,
this lemma will be proved later on with another method).
(III.5.1) Lemma.
Hq(RP 2 ,Z) ∼ ⎧
⎨ Z , q = 0
= Z2 , q = 1
⎩
0 , q �= 0,1 .
We now consider the n-dimensional real projective spaces RPn , with n ≥ 3. As
for the two-dimensional case, RPn is a quotient space of Sn by identification of
antipodal points. Let us rephrase the definition of RPn as follows: We define the
equivalence relation in Rn+1 � (0,...,0):
x =(x0,...,xn) ≡ y =(y0,...,yn) ⇐⇒
(∃λ ∈ R,λ �= 0)(∀i = 0,...,n)xi = λyi .