Simplicial Structures in Topology
Simplicial Structures in Topology
Simplicial Structures in Topology
Create successful ePaper yourself
Turn your PDF publications into a flip-book with our unique Google optimized e-Paper software.
III.1 The Category of Polyhedra 107<br />
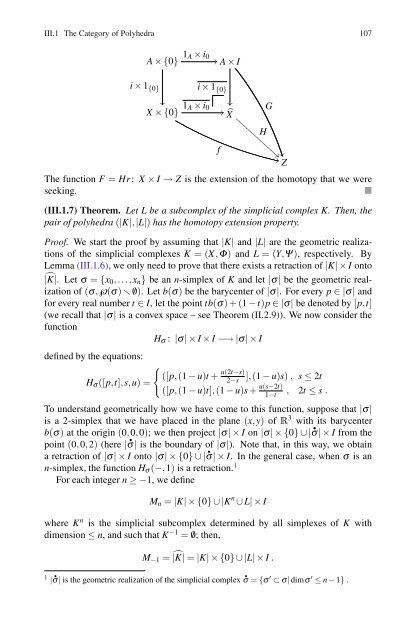
A ×{0} 1A × i0 ��<br />
A × I<br />
��<br />
��<br />
��<br />
��<br />
��<br />
��<br />
i × 1 {0} i × 1 {0}<br />
1A × i0<br />
X ×{0}<br />
G<br />
�X ���<br />
���<br />
�H<br />
���<br />
f<br />
��<br />
Z<br />
The function F = Hr: X × I → Z is the extension of the homotopy that we were<br />
seek<strong>in</strong>g. �<br />
(III.1.7) Theorem. Let L be a subcomplex of the simplicial complex K. Then, the<br />
pair of polyhedra (|K|,|L|) has the homotopy extension property.<br />
Proof. We start the proof by assum<strong>in</strong>g that |K| and |L| are the geometric realizations<br />
of the simplicial complexes K =(X,Φ) and L =(Y,Ψ), respectively. By<br />
Lemma (III.1.6), we only need to prove that there exists a retraction of |K|×I onto<br />
�|K|. Let σ = {x0,...,xn} be an n-simplex of K and let |σ| be the geometric realization<br />
of (σ,℘(σ) � /0). Letb(σ) be the barycenter of |σ|. Foreveryp ∈|σ| and<br />
for every real number t ∈ I, let the po<strong>in</strong>t tb(σ)+(1 − t)p ∈|σ| be denoted by [p,t]<br />
(we recall that |σ| is a convex space – see Theorem (II.2.9)). We now consider the<br />
function<br />
Hσ : |σ|×I × I −→ |σ|×I<br />
def<strong>in</strong>ed by the equations:<br />
Hσ([p,t],s,u)=<br />
�<br />
([p,(1 − u)t + u(2t−s)<br />
2−s ],(1 − u)s) , s ≤ 2t<br />
([p,(1 − u)t],(1 − u)s + u(s−2t)<br />
1−t , 2t ≤ s .<br />
To understand geometrically how we have come to this function, suppose that |σ|<br />
is a 2-simplex that we have placed <strong>in</strong> the plane (x,y) of R3 with its barycenter<br />
b(σ) at the orig<strong>in</strong> (0,0,0); we then project |σ|×I on |σ|×{0}∪| •<br />
σ|×I from the<br />
po<strong>in</strong>t (0,0,2) (here | •<br />
σ| is the boundary of |σ|). Note that, <strong>in</strong> this way, we obta<strong>in</strong><br />
a retraction of |σ|×I onto |σ|×{0}∪| •<br />
σ|×I. In the general case, when σ is an<br />
n-simplex, the function Hσ (−,1) is a retraction. 1<br />
For each <strong>in</strong>teger n ≥−1, we def<strong>in</strong>e<br />
Mn = |K|×{0}∪|K n ∪ L|×I<br />
where K n is the simplicial subcomplex determ<strong>in</strong>ed by all simplexes of K with<br />
dimension ≤ n, and such that K −1 = /0; then,<br />
M−1 = � |K| = |K|×{0}∪|L|×I .<br />
1 | • σ| is the geometric realization of the simplicial complex • σ = {σ ′ ⊂ σ|dimσ ′ ≤ n − 1} .