Simplicial Structures in Topology
Simplicial Structures in Topology
Simplicial Structures in Topology
Create successful ePaper yourself
Turn your PDF publications into a flip-book with our unique Google optimized e-Paper software.
84 II <strong>Simplicial</strong> Complexes<br />
(that is to say, ˜hn(c+Cn(L)) = ˜jn ˜kn(c)). The function ˜hn is well def<strong>in</strong>ed; <strong>in</strong> fact, had<br />
c ′ ∈ Cn(K) been such that c−c ′ ∈ Cn(L), we would have c−c ′ ∈ Cn(K ∩CL) and so<br />
˜jn ˜kn(c − c ′ )=˜jnĩn(c − c ′ )=0.<br />
The homomorphism sequences ˜h = {˜hn|n ≥ 0} and ˜k = {˜kn|n ≥ 0} are homomorphisms<br />
of cha<strong>in</strong> complexes giv<strong>in</strong>g rise to a commutative diagram<br />
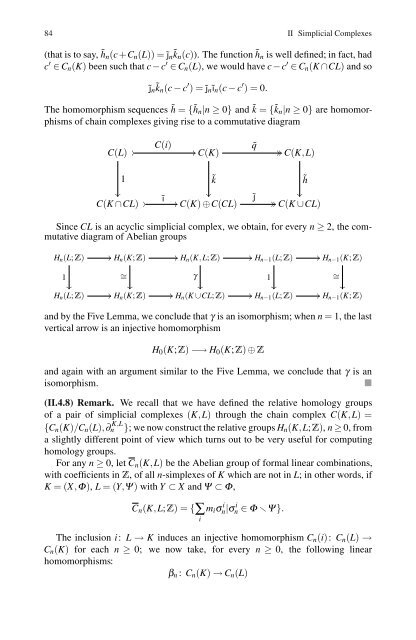
C(L) ��<br />
C(i)<br />
��<br />
��<br />
1<br />
˜k<br />
C(K ∩CL) ��<br />
��<br />
ĩ<br />
C(K) ⊕C(CL)<br />
��<br />
C(K)<br />
˜q<br />
˜j<br />
��<br />
��<br />
C(K,L)<br />
˜h<br />
��<br />
��<br />
��<br />
C(K ∪CL)<br />
S<strong>in</strong>ce CL is an acyclic simplicial complex, we obta<strong>in</strong>, for every n ≥ 2, the commutative<br />
diagram of Abelian groups<br />
Hn(L;Z)<br />
1<br />
��<br />
Hn(L;Z)<br />
��<br />
Hn(K;Z)<br />
∼=<br />
��<br />
��<br />
Hn(K;Z)<br />
��<br />
Hn(K,L;Z)<br />
γ<br />
��<br />
��<br />
Hn(K ∪CL;Z)<br />
��<br />
Hn−1(L;Z)<br />
1<br />
��<br />
��<br />
Hn−1(L;Z)<br />
��<br />
Hn−1(K;Z)<br />
∼=<br />
��<br />
��<br />
Hn−1(K;Z)<br />
and by the Five Lemma, we conclude that γ is an isomorphism; when n = 1, the last<br />
vertical arrow is an <strong>in</strong>jective homomorphism<br />
H0(K;Z) −→ H0(K;Z) ⊕ Z<br />
and aga<strong>in</strong> with an argument similar to the Five Lemma, we conclude that γ is an<br />
isomorphism. �<br />
(II.4.8) Remark. We recall that we have def<strong>in</strong>ed the relative homology groups<br />
of a pair of simplicial complexes (K,L) through the cha<strong>in</strong> complex C(K,L) =<br />
{Cn(K)/Cn(L),∂ K,L<br />
n }; we now construct the relative groups Hn(K,L;Z), n ≥ 0, from<br />
a slightly different po<strong>in</strong>t of view which turns out to be very useful for comput<strong>in</strong>g<br />
homology groups.<br />
For any n ≥ 0, let Cn(K,L) be the Abelian group of formal l<strong>in</strong>ear comb<strong>in</strong>ations,<br />
with coefficients <strong>in</strong> Z, ofalln-simplexes of K which are not <strong>in</strong> L; <strong>in</strong> other words, if<br />
K =(X,Φ), L =(Y,Ψ) with Y ⊂ X and Ψ ⊂ Φ,<br />
Cn(K,L;Z)={∑ i<br />
miσ i n |σ i n ∈ Φ �Ψ}.<br />
The <strong>in</strong>clusion i: L → K <strong>in</strong>duces an <strong>in</strong>jective homomorphism Cn(i): Cn(L) →<br />
Cn(K) for each n ≥ 0; we now take, for every n ≥ 0, the follow<strong>in</strong>g l<strong>in</strong>ear<br />
homomorphisms:<br />
βn : Cn(K) → Cn(L)