COMPUTATIONAL PROBLEMS IN ABSTRACT ALGEBRA.

368 Shen Lin
sequence of squares, the a,‘s settle down to around 5. Since we know that
all sufficiently large odd numbers can be expressed as a sum of three
or fewer primes and all sufficiently large numbers can be expressed
as a sum of five or fewer distinct squares, we are led to the following conjecture
and theorem :
CONJECTURE. Lim sup a,, exists for all complete polynomial sequences
and the sequence of primes, sequence of squares of primes, etc. In particular,
[lim sup a,] = 3 for the sequence of primes and [lim sup a,] = 5 for the
sequence generated by x2.
Note that if this conjecture is true, then the status of the Goldbach
conjecture can be settled by finite enumeration as can be seen from the
following theorem :
THEOREM: Let S E (~1, s2, . . . , Sk, . . .> be an essentially complete
sequence. Suppose there exists an N and an a such that for all n - N,
Wn,,)
a, E ___
%
-C a. Then all sufficiently large integers can be expressed
as a sum of at most [a] distinct terms from S, where [x] stands for the
largest integer G x. As a consequence thereof, S forms an integral basis
for large numbers of order at most [a]. At any rate, the conclusion is true
for all numbers y, O(S,) -C y -z q(S,,+,), for which a, -K a.
Proof. Let y =- 6(&J; then we may find an n Z= N such that 6(S,J -C y <
e(S,+,). Since y is greater than 0(&J, y is representable as a sum of distinct
terms from S,,, say y = Si,+Si, + . . . +Si, where each s4 3 s,. Hence
y * ts,. On the other hand, y 4 0(S,,+,) < asn. Hence t -K a. Since t is
an integer, t < [a].
While computer work cannot yet establish the validity of the assumption
needed in the above theorem, we believe that it can give us a fairly good
indication of what a may be, if it exists. It is hoped that experimental
work of this kind can help us formulate meaningful conjectures that some
one can prove at a later date.
APPENDIX A
Thresholds of completeness have been computed for many sequences,
and the behavior of the respective a,‘s studied. In the following tables we
give a summary of the computed results obtained for some selected sequences.
The generating function for each sequence (si = f(i)) is given on
top of each table and max (an) means the largest a,, in the range between the
two values of n heading the column in which the value of max(aJ is found.
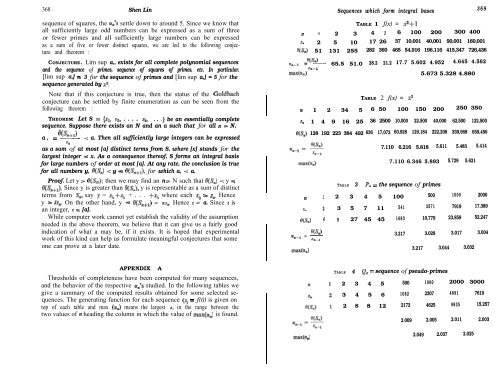
Sequences which form integral bases
TABLE 1 f(x) = x2+1
n 1 2 3 4 5 6 100 200 300 400
&a 2 5 10 17 26 37 10,001 40,001 90,001 160,001
WI) 51 131 255 282 360 465 54,916 196,116 415,347 726,436
q&l
a.-1 = - 65.5 51.0 28.2 21.2 17.7 5.602 4.952 4.645 4.562
h-1
max@,) 5.673 5.328 4.880
TABLE 2 f(x) = x2
n 1 2 34 5 6 50 100 150 200 250 350
$98 1 4 9 16 25 36 2500 10,000 22,500 40,000 62,500 122,500
x9(&) 128 192 223 384 492 636 17,072 60,928 129,184 222,208 339,968 659,456
n
&I
1
2
&S,> 6
~(S,>
asel = -I_
G-1
max@,)
max(4
369
7.110 6.216 5.818 5.611 5.483 5.414
7.110 6.346 5.893
TABLE 3 P,, FE the sequence of primes
2 3 4 5 100 500
3 5 7 11 541 3571
9 27 45 45 1683 10,779
3.217 3.028
5.729 5.621
1000 2000
7919 17,389
23,859 52,247
3.017 3.004
3.217 3.044 3.032
TABLE 4 Q,, E sequence of pseudo-primes
2 3 4 5 500 1000 2000 3000
3 4 5 6 1082 2307 4891 7619
2 8 8 12 2172 4625 9835 15,257
2.009 2.005 2.011 2.003
2.049 2.037 2.025