COMPUTATIONAL PROBLEMS IN ABSTRACT ALGEBRA.

More documents

Recommendations

Info

358 J. H. Conway 1. 2. 3. 4. 5. 6. 7. 8. 9. 10. 11. REFERENCES J. W. ALEXANDER and G. B. BRIGGS: On types of knotted curves. Ann. of Math. 28 (1926-27), 562. A. L. ANGER: Machine calculation of knot polynomials. Princeton Senior Thesis, 1959. R. H. FOX: A quick trip through knot theory. Topology of 3-manifolds and related topics (Prentice-Hall, Englewood Cliffs, N.J., 1962). C. N. LITTLE: On knots, with a census to order 10. Trans. Conn. Acad. Sci. 18 (1885), 374378. C. N. LITTLE: Alternate + knots of order 11. Trans. Roy. Sot. Edin. 36 (1890), 253-255. C. N. LITTLE: Non-alternate + knots. Trans. Roy. Sot. Edin. 39 (1900), 771-778. K. MURASUGI: On a certain numerical invariant of link types. Trans. Amer. Math. Sot. 117 (1965), 387-422. K. REIDEMEISTER: Knotentheorie (reprint) (Chelsea, New York, 1948). P. G. TAIT: On knots, I. First published separately, but available togeth- P. G. TAIT: On knots, II. er in Tait’s Scientific Papers, Vol. I, pp. 273- P. G. TAIT: On knots, III. 347 (C.U.P., London, 1898). Computations in knot theory H. F. 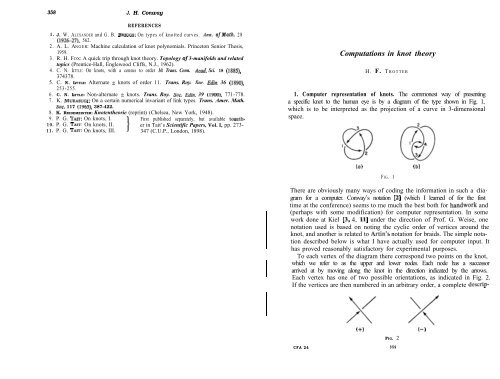T ROTTER 1. Computer representation of knots. The commonest way of presenting a specific knot to the human eye is by a diagram of the type shown in Fig. 1, which is to be interpreted as the projection of a curve in 3-dimensional space. (0) FIG. 1 There are obviously many ways of coding the information in such a dia- I gram for a computer. Conway’s notation [2] (which I learned of for the first time at the conference) seems to me much the best both for handwork and (perhaps with some modification) for computer representation. In some work done at Kiel [3, 4, 111 under the direction of Prof. G. Weise, one notation used is based on noting the cyclic order of vertices around the knot, and another is related to Artin’s notation for braids. The simple notation described below is what I have actually used for computer input. It has proved reasonably satisfactory for experimental purposes. To each vertex of the diagram there correspond two points on the knot, which we refer to as the upper and lower nodes. Each node has a successor arrived at by moving along the knot in the direction indicated by the arrows. Each vertex has one of two possible orientations, as indicated in Fig. 2. I If the vertices are then numbered in an arbitrary order, a complete descrip- I CFA 24 (b) (+I t-1 FIG. 2 359
H. F. Trotter Computations in knot theory tion of the diagram is obtained by listing, for each vertex, its orientation and the successors of its upper and lower nodes. The descriptions of the diagrams in Fig. 1 are then (a) lSL2 U2 (b) 1 -L4 L2 2+L3 U3 2-U4 U3 3+Ll Ul 3-Ul u2 4-Ll L3 where L, U stand for “lower” and “upper”. This description is highly redundant, but the redundancy is at least in part a virtue, since it increases the likelihood that a coded description is correct if it is self-consistent. In practice, the notation is fairly easy to use, although errors in recording the orientations of vertices do crop up. The coding just described is intended to be convenient to write down, and does not correspond directly to a useful internal representation for a knot. Some sort of list organization appears to be most appropriate, and the list-processing language L6 [7] was chosen because it was available and t seemed to be well-suited to the problem. In addition to efficient mechanisms for storage allocation and subroutine organization, L6 has several distinctive features. It deals with blocks of computer words as single objects, and provides for declaration of fields (denoted by single letters) within blocks. A field may contain either a pointer to another block, or numerical or coded data. Indirect referencing (up to five levels deep) is denoted simply by concatenation. For example, XAT refers to field T of the block pointed to by field A of the block pointed to by pseudo-register X. A program has been written which reads a knot description, making some elementary checks for consistency, and produces a linked list of blocks in which there is one block for each node. Each block contains fields which point to the preceding and following nodes and to the other node at the same vertex. Other fields contain the vertex number and indicators for the orientation of the vertex and the type of the node (upper or lower). The program will also handle links (i.e. knots with more than one component) and another field contains the number of the component to which the node belongs. In addition, each block contains two fields used to link it temporarily into various lists for housekeeping purposes during computation. In this representation it turned out to be quite easy to write a program to perform trivial simplifications of diagrams by application of the Reidemeister moves 9.1,0.2 ([12], p. 7) (see Fig. 3). 2. Computation of algebraic invariants of knots. The most straightforward application of computers to knot theory is in the computation of known algebraic invariants. The first work of this kind that I know of was done in 1959 at Princeton [l] on an IBM 650 computer, and consisted of the calculation of Alexander polynomials for the alternating lo-crossing knots in Tait’s tables [15]. Similar calculations were later made for the non- i I alternating lo-crossing knots and for the alternating 1 l-crossing knots in the tables of Tait and Little [8,9,10,15]. (Conway independently did these calculations by hand for his own knot tables [2].) Programs for computing the Alexander polynomial and several other invariants are described in [3]. The most generally useful algebraic invariants of knots are connected with the homology of cyclic coverings of the complement of the knot. Seifert [14] showed that these invariants can be computed from an integer matrix constructed as follows. The first step is to find a non-self-intersecting orientable surface with the knot as boundary. (It is not altogether obvious that such a surface always exists, but Seifert actually indicates a method of constructing one for any knot diagram.) Unless the knot is trivial, the surface has a genus h greater than 0, and its first homology group is free abelian on 2h generators. A system of 2h closed curves which represent an integral basis for this homology group can be found on the surface. A Seifert matrix for the knot is then obtained by taking as ijth entry the linking number (in 3-space) of the ith basis curve with a curve obtained by lifting the jth basis curve slightly above the surface. (The side of the surface which is “above” is of course determined by the orientation of the surface.) Any knot has infinitely many different Seifert matrices belonging to it (and any Seifert matrix belongs to infinitely many distinct knots). Programming an algorithm to find a Seifert matrix from a knot description is an interesting problem. An especially noteworthy program in [3] actually finds a Seifert surface and transforms it into the canonical form of a disk with attached bands, from which a Seifert matrix is then easily obtained. I have written an L6 program which finds a set of basis curves and computes the matrix without first altering the surface. Let us say that two Seifert matrices are s-equivalent if they are the first and last members of some finite sequence of matrices such that for each consecutive pair in the sequence there is some knot to which both matrices of the pair belong. No purely algebraic characterization of s-equivalence is known, but it is closely related to the property of congruence of matrices. We say that matrices A and B are congruent over a ring R if A = PBP’ where P’ is the transpose of P, and both P and its inverse have elements in R. It is quite obvious that two Seifert matrices which are congruent over the integers are s-equivalent, since any such change in the matrix can be obtained simply by choosing a different basis for the first homology group of the Seifert surface. The converse is known to be false, but a modified converse is true [16]. Before stating this converse we must remark that any Seifert matrix is s-equivalent to a non-singular one (unless it is s-equivalent to one belonging to the trivial knot), and that if V and W are s-equivalent non-singular matricesthendet(V)=det(W).(Infactdet(V- tV’)=det(W- tW’)=A(t), the Alexander polynomial of the knot.) The statement then is that two 24.
Page 1 and 2:
COMPUTATIONAL PROBLEMS IN ABSTRACT
Page 3 and 4:
vi Contents E. KRAUSE and K. WESTON
Page 5 and 6:
X Preface I am indebted to the auth
Page 7 and 8:
4 J. Neubiiser 2.3.5. Added in proo
Page 9 and 10:
8 J. Neubiiser Investigations of gr
Page 11 and 12:
12 J. Neubiiser For 1 es 4 r, ws is
Page 13 and 14:
16 J. Neubiiser Investigations of g
Page 23 and 24:
Some examples using coset enumerati
Page 25 and 26:
40 C. M. Campbell Some examples usi
Page 27 and 28:
44 N. S. Mendelsohn for example, in
Page 29 and 30:
48 H. Jiirgensen Calculation with e
Page 31 and 32:
Page 33 and 34:
Page 35 and 36:
60 V. Fe&h and J. Neubiiser 2. The
Page 37 and 38:
64 L. Gerhards and E. Altmann Autom
Page 39 and 40:
68 L. Gerhards and E. Altmann Autom
Page 41 and 42:
72 L. Gerhards and E. Altmann ni E
Page 43 and 44:
76 W. Lindenberg and L. Gerhards Se
Page 45 and 46:
80 W. Lindenberg and L. Gerhards Se
Page 47 and 48:
84 K. Ferber and H. Jiirgensen The
Page 49 and 50:
7 The construction of the character
Page 51 and 52:
92 John McKay defining multiplicati
Page 53 and 54:
96 John McKay Construction of chara
Page 55 and 56:
100 John McKay In the table, c indi
Page 57 and 58:
104 C. Brott and J. Neubiiser irred
Page 59 and 60:
108 C. Brott and J. Neubiiser eleme
Page 61 and 62:
112 J. S. Frame The characters of t
Page 63 and 64:
116 J. S. Frame 3. The decompositio
Page 65 and 66:
Page 67 and 68:
Page 69 and 70:
TABLE 5. The Z, character block for
Page 71 and 72:
132 R. Biilow and J. Neubiiser Deri
Page 73 and 74:
A search for simple groups of order
Page 75 and 76:
140 Marshall Hall Jr. Simple groups
Page 77 and 78:
Page 79 and 80:
148 Marshall Hall Jr. otherwise no
Page 81 and 82:
152 Marshall Hall Jr. easily found
Page 83 and 84:
Page 85 and 86:
160 Marshall Hail Jr. This leads to
Page 87 and 88:
164 Marshall Hall Jr. b= (OO)(Ol, 0
Page 89 and 90:
168 Marshall Hall Jr. 11. R. BRAUER
Page 91 and 92:
172 Charles C. Sims THEOREM 2.3. Th
Page 93 and 94:
176 Charles C. Sims has a set of im
Page 95 and 96:
180 Degrc - No. Order t N (-63 Gene
Page 97 and 98:
An algorithm related to the restric
Page 99 and 100:
A module-theoretic computation rela
Page 101 and 102:
192 A. L. Tritter expressed in the
Page 103 and 104:
196 A. L. Tritter a question is mos
Page 105 and 106:
200 John J. Cannon stack. The Cayle
Page 107 and 108:
, I The computation of irreducible
Page 109 and 110:
208 P. G. Ruud and R. Keown product
Page 111 and 112:
212 P. G. Ruud and R. Keown TX wher
Page 113 and 114:
216 P. G. Ruud and R. Keown 4. L. G
Page 115 and 116:
220 N. S. Mendelsohn where it is kn
Page 117 and 118:
224 Robert J. Plemmons such class [
Page 119 and 120:
228 Robert J. Plemmons ! TOTALS Sem
Page 121 and 122:
232 Takayuki Tamura 8” = 0B if an
Page 123 and 124:
236 Takayuki Tamura Case ,Q = {e).
Page 125 and 126:
240 Takayuki Tamura The calculation
Page 127 and 128:
244 Takayuki Tamura We calculate46
Page 129 and 130:
248 Takayuki Tamura Immediately we
Page 131 and 132:
252 Takayuki Tamura Let U be a subs
Page 133 and 134: 256 Takayuki Tamura TABLE 8. AI1 Se
Page 135 and 136: I The author and R. Dickinson have
Page 137 and 138: 264 Donald E. Knuth and Peter B. Be
Page 155 and 156: 300 Lowell J. Paige Non-associative
Page 157 and 158: 304 Lowell J. Paige We may lineariz
Page 159 and 160: 308 T(n) U(n) 0) [VI R-4 C. M. Glen
Page 161 and 162: 312 C. M. Glennie where in each cas
Page 163 and 164: 316 A. D. Keedwell of the elements
Page 165 and 166: A projective coqfiguration J. W. P.
Page 167 and 168: The uses of computers in Galois the
Page 169 and 170: 328 W. D. Maurer mod p; for a facto
Page 171 and 172: 332 J. H. Conway Enumeration of kno
Page 173 and 174: J. H. Conway Enumeration of knots a
Page 175 and 176: 340 J. H. Conway -thus our symmetry
Page 177 and 178: 344 J. H. Conway 2 string links to
Page 179 and 180: 348 J. H. Conway 3 and 4 string lin
Page 181 and 182: 352 Non-alternating J. H. Conway L
Page 183: 356 J. H. Conway Alternating 11 cro
Page 187 and 188: 364 H. F. Trotter to a. Schubert [1
Page 189 and 190: 368 Shen Lin sequence of squares, t
Page 191 and 192: 372 Harvey Cohn We also consider GZ
Page 193 and 194: 376 Harvey Cohn In the case of Fig.
Page 195 and 196: 380 Harvey Cohn always dictated by
Page 197 and 198: 384 Hans Zassenhaus A real root cal
Page 199 and 200: 388 Hans Zassenhaus the elements A,
Page 201 and 202: 392 Hans Zassenhaus It is clear fro
Page 203 and 204: 396 R. E. Kalman Invariant factors
Page 205 and 206: 400 List of participants DR. J. J.
show all

COMPUTATIONAL PROBLEMS IN ABSTRACT ALGEBRA.

Create successful ePaper yourself

Delete template?

Save as template?