COMPUTATIONAL PROBLEMS IN ABSTRACT ALGEBRA.

342 J. H. Conway
each knot has been subjected to a reduction procedure which in every
known case has been shown to yield all forms with minimal crossing
number.) By the same token, all knots listed are believed to be prime.
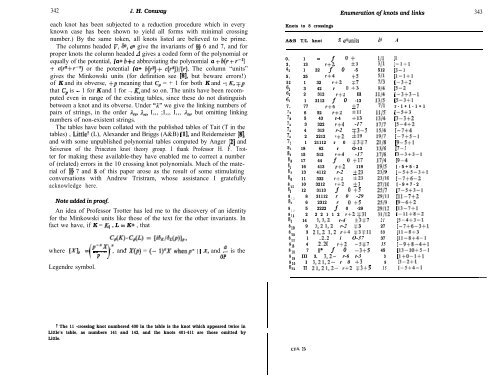
The columns headed V, 60, a0 give the invariants of @ 6 and 7, and for
proper knots the column headed d gives a coded form of the polynomial or
equally of the potential, [a+ b+c abbreviating the polynomial a + b(r + r-3
+ c(r2+rm2) or the potential (a+ b{r2}+ c{r”})/{r}. The column “units”
gives the Minkowski units (for definition see [S], but beware errors!)
of K and its obverse, fp meaning that C, = + 1 for both K and 1 K, +p
that C, is - 1 for K and 1 for 7 K, and so on. The units have been recomputed
even in range of the existing tables, since these do not distinguish
between a knot and its obverse. Under “1” we give the linking numbers of
pairs of strings, in the order il,,, &,, I,,, ;I,,, I,,, &,,, but omitting linking
numbers of non-existent strings.
The tables have been collated with the published tables of Tait (T in the
tables) , Little? (L), Alexander and Briggs (A&B) [l], and Reidemeister [8],
and with some unpublished polynomial tables computed by Anger [2] and
Seiverson of the Princeton knot theory group. I thank Professor H. F. Trotter
for making these available-they have enabled me to correct a number
of (related) errors in the 10 crossing knot polynomials. Much of the material
of $0 7 and 8 of this paper arose as the result of some stimulating
conversations with Andrew Tristram, whose assistance I gratefully
acknowledge here.
Note added in proof.
An idea of Professor Trotter has led me to the discovery of an identity
for the Minkowski units like those of the text for the other invariants. In
fact we have, if K = KO , L = K+ , that
where [Xl, = - ,
( P )
Legendre symbol.
and X(p) = (- l>xX whenpX 11 X, and 5 is the
0P
t The 11 -crossing knot numbered 400 in the table is the knot which appeared twice in
Little’s table, as numbers 141 and 142, and the knots 401-411 are those omitted by
Little.
Knots to 8 crossings
Enumeration of knots and links
A&B T/L knot S a%nits 60 A
0, 1 - f Of l/l D
3, 13 r+2 +3 3/l [-lfl
41 1 22 f 0 -5 512 [3- 1
5, 25 r+4 +5 5/l [1-lfl
52 1 32 rf2 T7 713 [-3+2
6, 3 42 r 0+3 914 [5-2
62 2 312 rf2 ill 11/4 [-3+3-l
63 1 2112 f 0 -13 13/5 [5-3+1
7, 77 rf6 f7 7/l r-1+1-1+1
72 6 52 r+2 *11 11/5 r--5+3
7a 5 43 r-4 +13 1314 [3-3+2
7, 3 322 rf4 -17 1717 [5-4+2
7, 4 313 r-2 73-5 1514 i-7+4
7, 2 2212 r+2 +19 1917 r-7+5-1
7, 1 21112 r 0 f3f7 21/a [9-5;1
8, 18 62 r O-13 13/6 [7-3
82 15 512 r-k4 -17 17/G [3-3+3-l
88 17 44 f 0+17 1714 [9-4
84 16 413 r+2 119 19/5 [-5+5-2
8, 13 4112 r-2 t23 2319 r-5+5-3-!-1
8, 11 332 r+2 f23 23/10 r-7+6-2
8 11 10 3212 rf2 f3 27/10 [-9+7-2
f-6 12 3113 f 0+5 2517 [7-5+3-l
8 12 8 31112 r 0 -29 29/11 [ll-7+2
8, 6 2312 r 0+5 2519 [9-6+2
8 12 5 2222 f 0 -29 29/12 [13-7fl
8 14 2 2 2 1 1 2 r+2 +31 31/12 [-11+8-2
85 14 3,3,2 r-4 +3+7 21 [5-4+3-l
8 10 9 3,21,2 r-2 f3 27 [-7+6-3+1
8 15 3 21,2 1,2 rf4 T3fll 33 [11-8+3
8 17 1 .2.2 i O-37 37 [11-8$-4-l
8 16 4 .2.20 r+2 -5+7 35 [-9+8-4+1
8 18 7 8* f 0 -3+5 45 [13-10+5-l
8 t9 III 3, 3,2- r-6 t-3 3 [1+0-l-+1
8 20 I 3,21,2- r 0 +3 9 [3-2fl
821 II 21,21,2- r+2 $3+5 15 [-5+4-l
CPA 23
343
-