COMPUTATIONAL PROBLEMS IN ABSTRACT ALGEBRA.

304 Lowell J. Paige
We may linearize the defining identity (a2b)a = a2(ba) of a Jordan algebra
to obtain the identities:
and
(w4Dz) + (wJ>W + (4bY)
= [w(xy>lz+ [wtiz)lx+ [w(zx)ly (3.1)
= [(wx)zl.Y + [(WY)ZlX - [(WZ>XlY - KWY)XlZ. (3.2)
Again, for convenience, let us denote the generators of Jc3) by a, b and c. If
we are interested in the identities of degree 8, then we might as well restrict
our attention to identities involving the generators a, b and c. Moreover,
it is known that any identity linear in one of the generators is valid for all
special Jordan algebras [13]. Hence, we may begin by considering those of
degree 3 in a, degree 3 in b and degree 2 in c.
We can now proceed to select w, x, y, z in (3.1) and (3.2) in all possible
ways so as to yield monomials a@cY compatible with the total degree
being 8 and involving a, b and c to the total degree 3, 3 and 2 respectively.
This will give us a set of homogeneous equations in the various monomial
elements of Jc3). Many duplications will be present and obvious reductions
may be made by using the commutative law. Let us assume that we have p
equations in m elements. The m elements may be ordered and we proceed to
reduce the equations to echelon form.
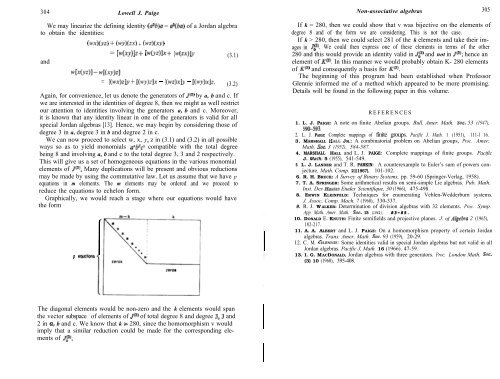
Graphically, we would reach a stage where our equations would have
the form
p equotiom
The diagonal elements would be non-zero and the k elements would span
the vector subspace of elements of Jc3) of total degree 8 and degree 3,3 and
2 in a, b and c. We know that k == 280, since the homomorphism v would
imply that a similar reduction could be made for the corresponding elements
of Jh3).
Non-associative algebras
If k = 280, then we could show that v was bijective on the elements of
degree 8 and of the form we are considering. This is not the case.
If k > 280, then we could select 281 of the k elements and take their images
in J,, t3). We could then express one of these elements in terms of the other
280 and this would provide an identity valid in Ji3) and not in Jc3); hence an
element of Kc3). In this manner we would probably obtain K- 280 elements
of Kc31 and consequently a basis for S3).
The beginning of this program had been established when Professor
Glennie informed me of a method which appeared to be more promising.
Details will be found in the following paper in this volume.
REFERENCES
1. L. J. PAIGE: A note on finite Abelian groups. Bull. Amer. Math. Sot. 53 (1947),
590493.
2. L. J. PAIGE: Complete mappings of finite groups. Pacific J. Math. 1 (1951), 111-l 16.
3. MARSHALL HAL JR.: A combinatorial problem on Abelian groups, Proc. Amer.
Math. Sot. 3 (1952), 584-587.
4. MARSHALL HALL and L. J. PAIGE: Complete mappings of finite groups. Pacific
J. Math. 5 (1955), 541-549.
5. L. J. LANDER and T. R. PARKIN: A counterexample to Euler’s sum of powers conjecture.
Math. Comp. 21(1967), 101-102.
6. R. H. BRUCK: A Survey of Binary Systems, pp. 59-60 (Springer-Verlag, 1958).
7. T. A. SPRINGER: Some arithmetical results on semi-simple Lie algebras. Pub. Math.
Inst. Des Halites Etudes Scientifique, 30 (1966), 475-498.
8. ERWIN KLEINFELD: Techniques for enumerating Veblen-Wedderburn systems.
J. Assoc. Comp. Mach. 7 (1960), 330-337.
9. R. J. W ALKER: Determination of division algebras with 32 elements. Proc. Symp.
App. Math. Amer. Math. Sot. 15 (1962), 83-85.
10. DONALD E. KNUTH: Finite semifields and projective planes. J. of AZgebra 2 (1965),
182-217.
11. A. A. ALBERT and L. J. PAIGE: On a homomorphism property of certain Jordan
algebras. Trans. Amer. Math. Sot. 93 (1959), 20-29.
12. C. M. GLENWIE: Some identities valid in special Jordan algebras but not valid in all
Jordan algebras. Pacific J. Math. 16 (1966). 47-59.
13. I. G. MACDONALD, Jordan algebras with three generators. Proc. London Math. Sot.
(3) 10 (1960), 395-408.
305