5 Hirsch-Fye quantum Monte Carlo method for ... - komet 337

5.14 Nils Blümer
Σ0
Fourier →
Σ
G
�
ρ0(ε)
G(iωn) = dε
iωn−ε−Σ(iωn)
G −1 (iωn)=G −1 (iωn)+Σ(iωn)
G(τ) = −〈ΨΨ ∗ 〉 G[τ]
G
G
← Fourier
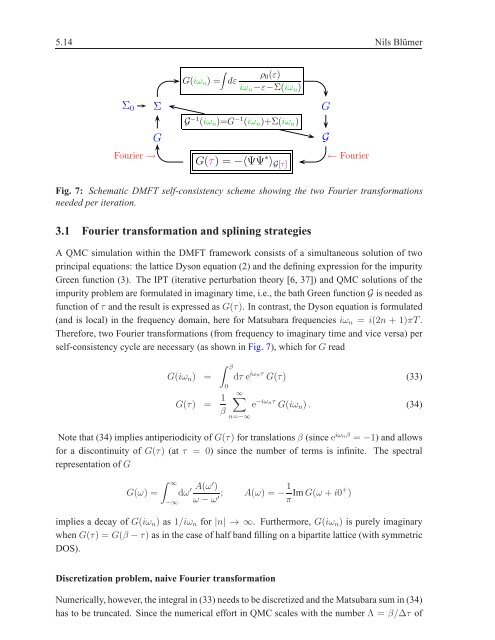
Fig. 7: Schematic DMFT self-consistency scheme showing the two Fourier transformations
needed per iteration.
3.1 Fourier transformation and splining strategies
A QMC simulation within the DMFT framework consists of a simultaneous solution of two
principal equations: the lattice Dyson equation (2) and the defining expression for the impurity
Green function (3). The IPT (iterative perturbation theory [6, 37]) and QMC solutions of the
impurity problem are formulated in imaginary time, i.e., the bath Green functionG is needed as
function ofτ and the result is expressed as G(τ). In contrast, the Dyson equation is formulated
(and is local) in the frequency domain, here for Matsubara frequencies iωn = i(2n + 1)πT .
Therefore, two Fourier transformations (from frequency to imaginary time and vice versa) per
self-consistency cycle are necessary (as shown in Fig. 7), which forGread
G(iωn) =
G(τ) = 1
β
� β
dτ e iωnτ G(τ) (33)
0
∞�
n=−∞
e −iωnτ G(iωn). (34)
Note that (34) implies antiperiodicity ofG(τ) for translationsβ (sincee iωnβ = −1) and allows
for a discontinuity of G(τ) (at τ = 0) since the number of terms is infinite. The spectral
representation ofG
� ∞
G(ω) = dω ′ A(ω′ )
ω −ω ′;
−∞
A(ω) = −1
π ImG(ω +i0+ )
implies a decay of G(iωn) as 1/iωn for |n| → ∞. Furthermore, G(iωn) is purely imaginary
when G(τ) = G(β −τ) as in the case of half band filling on a bipartite lattice (with symmetric
DOS).
Discretization problem, naive Fourier transformation
Numerically, however, the integral in (33) needs to be discretized and the Matsubara sum in (34)
has to be truncated. Since the numerical effort in QMC scales with the number Λ = β/∆τ of