The Nucleon-Nucleon Interaction in a Chiral Effective Field Theory

84
.... .
- - .... \ .
•
A
.... .
. - - - - .... \
•
A
.. _ - - - - - ,
•
a)
3. The derivation of nuclear forces from chiral Lagrangians
- - - _. - .... .
.... .
.... .
\
. .... \ \
• • •
b)
\
- -- - -, ...
'"'
.... .
- - - - \
•
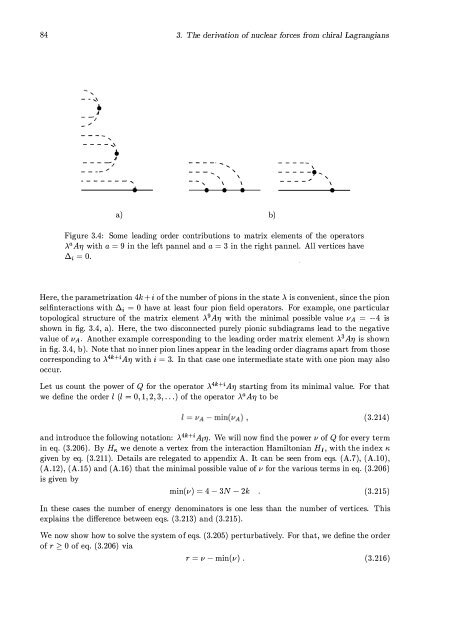
Figure 3.4: Some leading order contributions to matrix elements of the operators
.x a A'T} with a = 9 in the left pannel and a = 3 in the right pannel. All vertices have
ßi = o.
Here, the parametrization 4k + i of the number of pions in the state .x is convenient, since the pion
selfinteractions with ßi = 0 have at least four pion field operators. For example, one particular
topological structure of the matrix element .x 9 A'T} with the minimal possible value VA = -4 is
shown in fig. 3.4, a). Here, the two disconnected purely pionic subdiagrams lead to the negative
value of VA. Another example corresponding to the leading order matrix element .x3 A'T} is shown
in fig. 3.4, b). Note that no inner pion lines appear in the leading order diagrams apart from those
corresponding to .x 4k +i A'T} with i = 3. In that case one intermediate state with one pion may aiso
occur.
Let us count the power of Q for the operator .x4k +iA'T} starting from its minimal value. For that
we define the order l (l = 0, 1,2,3, ... ) of the operator .xa A'T} to be
(3.214)
and introduce the following notation: .x4k + i A/'T}. We will now find the power v of Q for every term
in eq. (3.206). By HK, we denote a vertex from the interaction Hamiltonian HJ, with the index K,
given by eq. (3.211). Details are relegated to appendix A. It can be seen from eqs. (A.7), (A.10),
(A.12), (A.15) and (A.16) that the minimal possible value of v for the various terms in eq. (3.206)
is given by
min(v) = 4 - 3N - 2k (3.215)
In these cases the number of energy denominators is one less than the number of vertices. This
explains the difference between eqs. (3.213) and (3.215).
We now show how to solve the system of eqs. (3.205) perturbatively. For that, we define the order
of r :::: : 0 of eq. (3.206) via
r = v - min(v) . (3.216)