The Nucleon-Nucleon Interaction in a Chiral Effective Field Theory

70 3. The derivation of nuclear forces from chiral Lagrangians
derivatives of
the pion field or pion mass insertions. We count the inner lines twice because the pion propagator
scales as 1/ q2 . The total number of independent integrations (loops) equals the number of inner
lines reduced by the number of delta functions corresponding to each vertex:
where L is the number of loops and Vi the number of vertices of the type i with di
L = 1 - (2: Vi - 1) , (3.145)
where we took into account the overall delta function in eq. (3.142). Therefore we obtain the
following result for the total scaling dimension LI of the transition amplitude M:
(3.146)
Weinberg made in 1979 a crucial observation [60] that this number LI is bounded from below.
Indeed, due to the spontaneously broken chiral symmetry and the Lorentz invariance of the Lagrangian,
no interactions with di � 2 are allowed.28 Furthermore, including loops leads to a
suppression by at least two powers of external momenta against the tree graph with the same
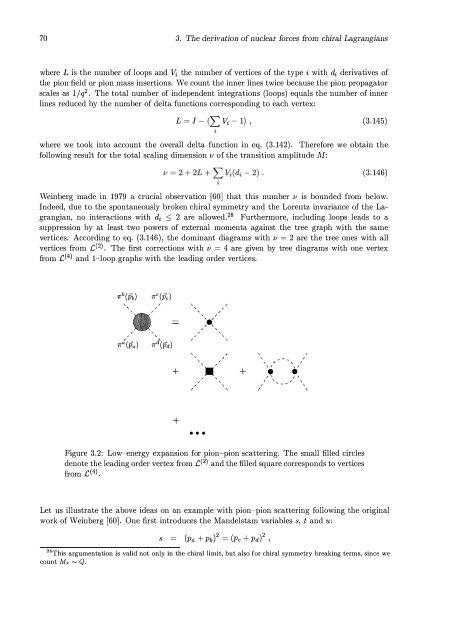
vertices. According to eq. (3.146), the dominant diagrams with LI = 2 are the tree ones with all
vertices from [J2). The first corrections with LI = 4 are given by tree diagrams with one vertex
from .c(4) and l-loop graphs with the leading order vertices.
1['b (Pb) 1['c (Pc)
1['a (Pa)
/
,
1[' d' (Pd)
+
+
,
,
• • •
/
'e / ,
,
•
/
/
/
\ /
\ I
I
, I
\ I
I \
I \
I \
/ \
+ • •
Figure 3.2: Low-energy expansion for pion-pion scattering. The small filled circles
denote the leading order vertex from [P) and the filled square corresponds to vertices
from .c(4).
Let us illustrate the above ideas on an example with pion-pion scattering following the original
work of Weinberg [60]. One first intro duces the Mandelstarn variables s, t and u:
S = (Pa + Pb) 2 = (Pe + Pd) 2 ,
28This argumentation is valid not only in the chiral limit, but also for chiral symmetry breaking terms, since we
count Mrr � Q.
, - -