fluid_mechanics

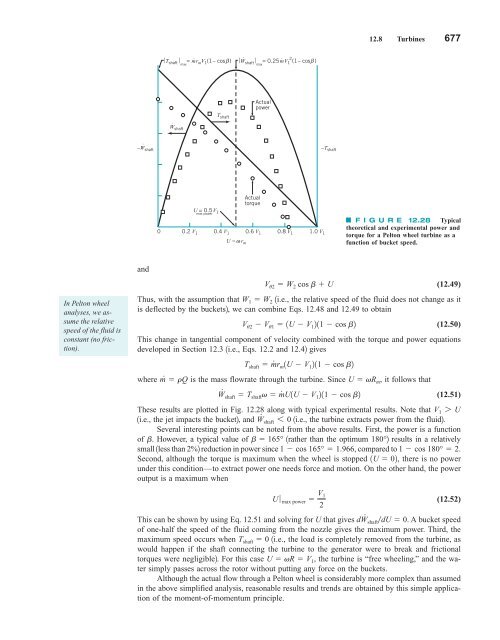
12.8 Turbines 677
⎥T shaft ⎥ = mr
⋅
β
max m V 1 (1– cos )
⋅
⎥ W ⎥ = 0.25mV
⋅ 2
shaft max 1 (1– cos β )
Actual
power
⋅
Wshaft
T shaft
⋅
–W shaft
–T shaft
U = 0.5V 1
max power
Actual
torque
0 0.2 V 1 0.4 V 1 0.6 V 1 0.8 V 1 1.0 V 1
U = ω r m
F I G U R E 12.28 Typical
theoretical and experimental power and
torque for a Pelton wheel turbine as a
function of bucket speed.
and
In Pelton wheel
analyses, we assume
the relative
speed of the fluid is
constant (no friction).
V u2 W 2 cos b U
(12.49)
Thus, with the assumption that W 1 W 2 1i.e., the relative speed of the fluid does not change as it
is deflected by the buckets2, we can combine Eqs. 12.48 and 12.49 to obtain
V u2 V u1 1U V 1 211 cos b2
(12.50)
This change in tangential component of velocity combined with the torque and power equations
developed in Section 12.3 1i.e., Eqs. 12.2 and 12.42 gives
T shaft m # r m 1U V 1 211 cos b2
where m # rQ is the mass flowrate through the turbine. Since U vR m , it follows that
W # shaft T shaft v m # U1U V 1 211 cos b2
(12.51)
These results are plotted in Fig. 12.28 along with typical experimental results. Note that
1i.e., the jet impacts the bucket2, and W # V 1 7 U
shaft 6 0 1i.e., the turbine extracts power from the fluid2.
Several interesting points can be noted from the above results. First, the power is a function
of b. However, a typical value of b 165° 1rather than the optimum 180° 2 results in a relatively
small 1less than 2%2 reduction in power since 1 cos 165° 1.966, compared to 1 cos 180° 2.
Second, although the torque is maximum when the wheel is stopped 1U 02, there is no power
under this condition—to extract power one needs force and motion. On the other hand, the power
output is a maximum when
U ƒ max power V 1
2
(12.52)
This can be shown by using Eq. 12.51 and solving for U that gives dW # shaftdU 0. A bucket speed
of one-half the speed of the fluid coming from the nozzle gives the maximum power. Third, the
maximum speed occurs when T shaft 0 1i.e., the load is completely removed from the turbine, as
would happen if the shaft connecting the turbine to the generator were to break and frictional
torques were negligible2. For this case U vR V 1 , the turbine is “free wheeling,” and the water
simply passes across the rotor without putting any force on the buckets.
Although the actual flow through a Pelton wheel is considerably more complex than assumed
in the above simplified analysis, reasonable results and trends are obtained by this simple application
of the moment-of-momentum principle.