fluid_mechanics
Share Embed Flag
Download ePaper

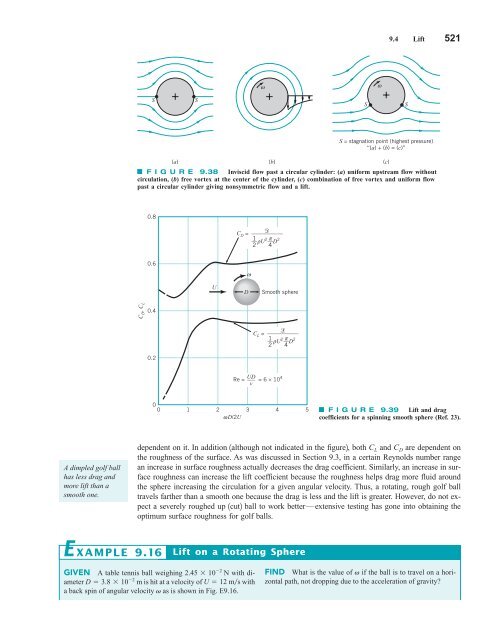
fluid_mechanics

claudia.marcela.becerra.rativa
from claudia.marcela.becerra.rativa More from this publisher
19.09.2019 Views

520 Chapter 9 ■ Flow over Immersed Bodies U B Bound vortex A Trailing vortex (a) A Low pressure High pressure Bound vortex Trailing vortex B F I G U R E 9.37 Flow past a finite length wing: (a) the horseshoe vortex system produced by the bound vortex and the trailing vortices; (b) the leakage of air around the wing tips produces the trailing vortices. (b) F l u i d s i n t h e N e w s Why winglets? Winglets, those upward turning ends of airplane wings, boost the performance by reducing drag. This is accomplished by reducing the strength of the wingtip vortices formed by the difference between the high pressure on the lower surface of the wing and the low pressure on the upper surface of the wing. These vortices represent an energy loss and an increase in drag. In essence, the winglet provides an effective increase in the aspect ratio of the wing without extending the wingspan. Winglets come in a variety of styles—the Airbus A320 has a very small upper and lower winglet; the Boeing 747-400 has a conventional, vertical upper winglet; and the Boeing Business Jet (a derivative of the Boeing 737) has an eight-foot winglet with a curving transition from wing to winglet. Since the airflow around the winglet is quite complicated, the winglets must be carefully designed and tested for each aircraft. In the past, winglets were more likely to be retrofitted to existing wings, but new airplanes are being designed with winglets from the start. Unlike tailfins on cars, winglets really do work. (See Problem 9.111.) A spinning sphere or cylinder can generate lift. As is indicated above, the generation of lift is directly related to the production of a swirl or vortex flow around the object. A nonsymmetric airfoil, by design, generates its own prescribed amount of swirl and lift. A symmetric object like a circular cylinder or sphere, which normally provides no lift, can generate swirl and lift if it rotates. As is discussed in Section 6.6.3, the inviscid flow past a circular cylinder has the symmetrical flow pattern indicated in Fig. 9.38a. By symmetry the lift and drag are zero. However, if the cylinder is rotated about its axis in a stationary real 1m 02 fluid, the rotation will drag some of the fluid around, producing circulation about the cylinder as in Fig. 9.38b. When this circulation is combined with an ideal, uniform upstream flow, the flow pattern indicated in Fig. 9.38c is obtained. The flow is no longer symmetrical about the horizontal plane through the center of the cylinder; the average pressure is greater on the lower half of the cylinder than on the upper half, and a lift is generated. This effect is called the Magnus effect, after Heinrich Magnus 11802–18702, a German chemist and physicist who first investigated this phenomenon. A similar lift is generated on a rotating sphere. It accounts for the various types of pitches in baseball 1i.e., curve ball, floater, sinker, etc.2, the ability of a soccer player to hook the ball, and the hook or slice of a golf ball. Typical lift and drag coefficients for a smooth, spinning sphere are shown in Fig. 9.39. Although the drag coefficient is fairly independent of the rate of rotation, the lift coefficient is strongly

A dimpled golf ball has less drag and more lift than a smooth one. dependent on it. In addition 1although not indicated in the figure2, both C and C D are dependent on 9.4 Lift 521 ω ω S S S S (a) (b) S = stagnation point (highest pressure) “(a) + (b) = (c)” (c) F I G U R E 9.38 Inviscid flow past a circular cylinder: (a) uniform upstream flow without circulation, (b) free vortex at the center of the cylinder, (c) combination of free vortex and uniform flow past a circular cylinder giving nonsymmetric flow and a lift. 0.8 C D = ____________ __ 1 U 2 __ π ρ D 2 2 4 0.6 ω U D Smooth sphere 0.4 0.2 C L = ____________ __ 1 U 2 __ π ρ D 2 2 4 Re = ___ = 6 × 10 4 v 0 0 1 2 3 4 5 F I G U R E 9.39 Lift and drag ωD/2U coefficients for a spinning smooth sphere (Ref. 23). L the roughness of the surface. As was discussed in Section 9.3, in a certain Reynolds number range an increase in surface roughness actually decreases the drag coefficient. Similarly, an increase in surface roughness can increase the lift coefficient because the roughness helps drag more fluid around the sphere increasing the circulation for a given angular velocity. Thus, a rotating, rough golf ball travels farther than a smooth one because the drag is less and the lift is greater. However, do not expect a severely roughed up 1cut2 ball to work better—extensive testing has gone into obtaining the optimum surface roughness for golf balls. C D , C L E XAMPLE 9.16 Lift on a Rotating Sphere GIVEN A table tennis ball weighing 2.45 10 2 N with diameter D 3.8 10 2 m is hit at a velocity of U 12 ms with zontal path, not dropping due to the acceleration of gravity? FIND What is the value of v if the ball is to travel on a hori- a back spin of angular velocity v as is shown in Fig. E9.16.

A dimpled golf ball<br />

has less drag and<br />

more lift than a<br />

smooth one.<br />

dependent on it. In addition 1although not indicated in the figure2, both C and C D are dependent on<br />

9.4 Lift 521<br />

ω<br />

ω<br />

S<br />

S<br />

S<br />

S<br />

(a)<br />

(b)<br />

S = stagnation point (highest pressure)<br />

“(a) + (b) = (c)”<br />

(c)<br />

F I G U R E 9.38 Inviscid flow past a circular cylinder: (a) uniform upstream flow without<br />

circulation, (b) free vortex at the center of the cylinder, (c) combination of free vortex and uniform flow<br />

past a circular cylinder giving nonsymmetric flow and a lift.<br />

0.8<br />

C D = ____________ <br />

__ 1 U 2 __ π<br />

ρ D 2<br />

2 4<br />

0.6<br />

ω<br />

U<br />

D Smooth sphere<br />

0.4<br />

0.2<br />

C L = ____________ <br />

__ 1 U 2 __ π<br />

ρ D 2<br />

2 4<br />

Re = ___ = 6 × 10 4<br />

v<br />

0<br />

0 1 2 3 4 5 F I G U R E 9.39 Lift and drag<br />

ωD/2U<br />

coefficients for a spinning smooth sphere (Ref. 23).<br />

L<br />

the roughness of the surface. As was discussed in Section 9.3, in a certain Reynolds number range<br />

an increase in surface roughness actually decreases the drag coefficient. Similarly, an increase in surface<br />

roughness can increase the lift coefficient because the roughness helps drag more <strong>fluid</strong> around<br />

the sphere increasing the circulation for a given angular velocity. Thus, a rotating, rough golf ball<br />

travels farther than a smooth one because the drag is less and the lift is greater. However, do not expect<br />

a severely roughed up 1cut2 ball to work better—extensive testing has gone into obtaining the<br />

optimum surface roughness for golf balls.<br />

C D , C L<br />

E XAMPLE 9.16<br />

Lift on a Rotating Sphere<br />

GIVEN A table tennis ball weighing 2.45 10 2 N with diameter<br />

D 3.8 10 2 m is hit at a velocity of U 12 ms with zontal path, not dropping due to the acceleration of gravity?<br />

FIND What is the value of v if the ball is to travel on a hori-<br />

a back spin of angular velocity v as is shown in Fig. E9.16.

logo

products

Resources

Company

Terms of service
Privacy policy
Cookie policy
Cookie settings
Imprint
Change language
Made with love in Switzerland
© 2024 Yumpu.com all rights reserved

Hooray! Your file is uploaded and ready to be published.

Saved successfully!

Ooh no, something went wrong!