fluid_mechanics

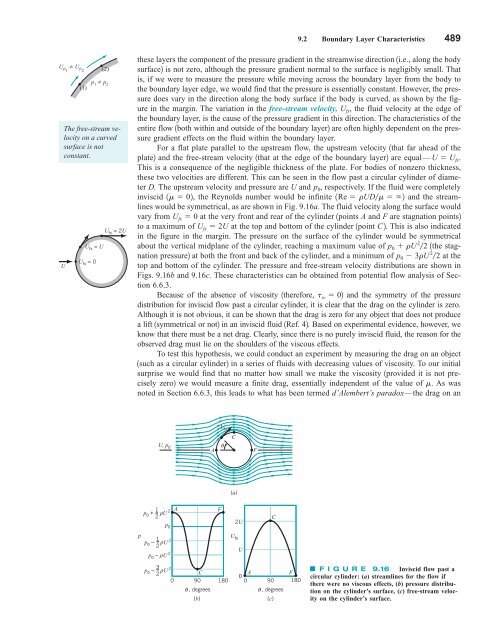
9.2 Boundary Layer Characteristics 489
U fs1 U fs2
p 1 p 2
The free-stream velocity
on a curved
surface is not
constant.
U
(1)
U fs = U
U fs = 0
(2)
U fs = 2U
these layers the component of the pressure gradient in the streamwise direction 1i.e., along the body
surface2 is not zero, although the pressure gradient normal to the surface is negligibly small. That
is, if we were to measure the pressure while moving across the boundary layer from the body to
the boundary layer edge, we would find that the pressure is essentially constant. However, the pressure
does vary in the direction along the body surface if the body is curved, as shown by the figure
in the margin. The variation in the free-stream velocity, U fs , the fluid velocity at the edge of
the boundary layer, is the cause of the pressure gradient in this direction. The characteristics of the
entire flow 1both within and outside of the boundary layer2 are often highly dependent on the pressure
gradient effects on the fluid within the boundary layer.
For a flat plate parallel to the upstream flow, the upstream velocity 1that far ahead of the
plate2 and the free-stream velocity 1that at the edge of the boundary layer2 are equal— U U fs .
This is a consequence of the negligible thickness of the plate. For bodies of nonzero thickness,
these two velocities are different. This can be seen in the flow past a circular cylinder of diameter
D. The upstream velocity and pressure are U and p 0 , respectively. If the fluid were completely
inviscid 1m 02, the Reynolds number would be infinite 1Re rUDm 2 and the streamlines
would be symmetrical, as are shown in Fig. 9.16a. The fluid velocity along the surface would
vary from U fs 0 at the very front and rear of the cylinder 1points A and F are stagnation points2
to a maximum of U fs 2U at the top and bottom of the cylinder 1point C2. This is also indicated
in the figure in the margin. The pressure on the surface of the cylinder would be symmetrical
about the vertical midplane of the cylinder, reaching a maximum value of p 0 rU 2 2 1the stagnation
pressure2 at both the front and back of the cylinder, and a minimum of p 0 3rU 2 2 at the
top and bottom of the cylinder. The pressure and free-stream velocity distributions are shown in
Figs. 9.16b and 9.16c. These characteristics can be obtained from potential flow analysis of Section
6.6.3.
Because of the absence of viscosity 1therefore, t w 02 and the symmetry of the pressure
distribution for inviscid flow past a circular cylinder, it is clear that the drag on the cylinder is zero.
Although it is not obvious, it can be shown that the drag is zero for any object that does not produce
a lift 1symmetrical or not2 in an inviscid fluid 1Ref. 42. Based on experimental evidence, however, we
know that there must be a net drag. Clearly, since there is no purely inviscid fluid, the reason for the
observed drag must lie on the shoulders of the viscous effects.
To test this hypothesis, we could conduct an experiment by measuring the drag on an object
1such as a circular cylinder2 in a series of fluids with decreasing values of viscosity. To our initial
surprise we would find that no matter how small we make the viscosity 1provided it is not precisely
zero2 we would measure a finite drag, essentially independent of the value of m. As was
noted in Section 6.6.3, this leads to what has been termed d’Alembert’s paradox—the drag on an
U fs
C
A
θ
F
(a)
U, p 0
A
1
p 0 + ρU 2
2
p 0
F
2U
C
p
1
p 0 – ρU 2
2
p 0 – ρU 2
U fs
U
3
p 0 – ρU 2
2
C
0 90 180
θ , degrees
(b)
A
F
0
0 90 180
θ , degrees
(c)
F I G U R E 9.16 Inviscid flow past a
circular cylinder: (a) streamlines for the flow if
there were no viscous effects, (b) pressure distribution
on the cylinder’s surface, (c) free-stream velocity
on the cylinder’s surface.