fluid_mechanics

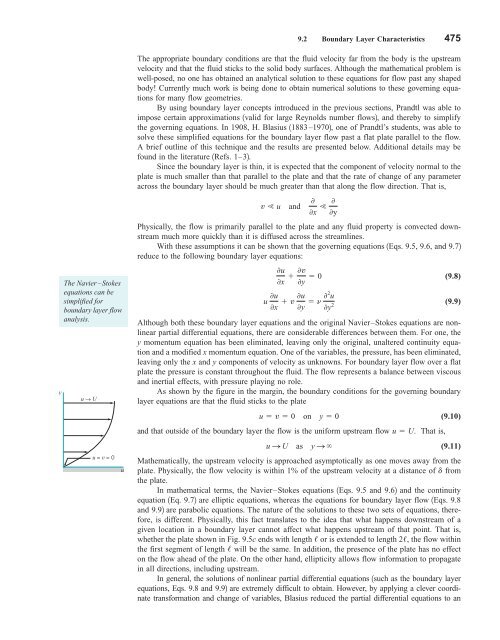
9.2 Boundary Layer Characteristics 475
y
The Navier –Stokes
equations can be
simplified for
boundary layer flow
analysis.
u → U
u = v = 0
u
The appropriate boundary conditions are that the fluid velocity far from the body is the upstream
velocity and that the fluid sticks to the solid body surfaces. Although the mathematical problem is
well-posed, no one has obtained an analytical solution to these equations for flow past any shaped
body! Currently much work is being done to obtain numerical solutions to these governing equations
for many flow geometries.
By using boundary layer concepts introduced in the previous sections, Prandtl was able to
impose certain approximations 1valid for large Reynolds number flows2, and thereby to simplify
the governing equations. In 1908, H. Blasius 11883 –19702, one of Prandtl’s students, was able to
solve these simplified equations for the boundary layer flow past a flat plate parallel to the flow.
A brief outline of this technique and the results are presented below. Additional details may be
found in the literature 1Refs. 1–32.
Since the boundary layer is thin, it is expected that the component of velocity normal to the
plate is much smaller than that parallel to the plate and that the rate of change of any parameter
across the boundary layer should be much greater than that along the flow direction. That is,
v u and 0
0x 0
0y
Physically, the flow is primarily parallel to the plate and any fluid property is convected downstream
much more quickly than it is diffused across the streamlines.
With these assumptions it can be shown that the governing equations 1Eqs. 9.5, 9.6, and 9.72
reduce to the following boundary layer equations:
0u
0x 0v
0y 0
u 0u
0x v 0u
0y n 02 u
0y 2
Although both these boundary layer equations and the original Navier–Stokes equations are nonlinear
partial differential equations, there are considerable differences between them. For one, the
y momentum equation has been eliminated, leaving only the original, unaltered continuity equation
and a modified x momentum equation. One of the variables, the pressure, has been eliminated,
leaving only the x and y components of velocity as unknowns. For boundary layer flow over a flat
plate the pressure is constant throughout the fluid. The flow represents a balance between viscous
and inertial effects, with pressure playing no role.
As shown by the figure in the margin, the boundary conditions for the governing boundary
layer equations are that the fluid sticks to the plate
u v 0 on y 0
and that outside of the boundary layer the flow is the uniform upstream flow u U. That is,
u S U as y S
(9.8)
(9.9)
(9.10)
(9.11)
Mathematically, the upstream velocity is approached asymptotically as one moves away from the
plate. Physically, the flow velocity is within 1% of the upstream velocity at a distance of d from
the plate.
In mathematical terms, the Navier–Stokes equations 1Eqs. 9.5 and 9.62 and the continuity
equation 1Eq. 9.72 are elliptic equations, whereas the equations for boundary layer flow 1Eqs. 9.8
and 9.92 are parabolic equations. The nature of the solutions to these two sets of equations, therefore,
is different. Physically, this fact translates to the idea that what happens downstream of a
given location in a boundary layer cannot affect what happens upstream of that point. That is,
whether the plate shown in Fig. 9.5c ends with length / or is extended to length 2/, the flow within
the first segment of length / will be the same. In addition, the presence of the plate has no effect
on the flow ahead of the plate. On the other hand, ellipticity allows flow information to propagate
in all directions, including upstream.
In general, the solutions of nonlinear partial differential equations 1such as the boundary layer
equations, Eqs. 9.8 and 9.92 are extremely difficult to obtain. However, by applying a clever coordinate
transformation and change of variables, Blasius reduced the partial differential equations to an