fluid_mechanics

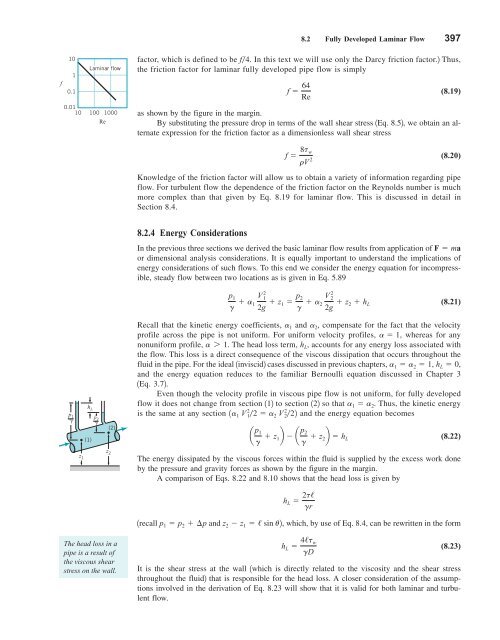
8.2 Fully Developed Laminar Flow 397
f
10
1
0.1
0.01
10 100
Laminar flow
Re
1000
factor, which is defined to be f4. In this text we will use only the Darcy friction factor.2 Thus,
the friction factor for laminar fully developed pipe flow is simply
f 64
Re
(8.19)
as shown by the figure in the margin.
By substituting the pressure drop in terms of the wall shear stress 1Eq. 8.52, we obtain an alternate
expression for the friction factor as a dimensionless wall shear stress
f 8t w
rV 2
(8.20)
Knowledge of the friction factor will allow us to obtain a variety of information regarding pipe
flow. For turbulent flow the dependence of the friction factor on the Reynolds number is much
more complex than that given by Eq. 8.19 for laminar flow. This is discussed in detail in
Section 8.4.
8.2.4 Energy Considerations
In the previous three sections we derived the basic laminar flow results from application of F ma
or dimensional analysis considerations. It is equally important to understand the implications of
energy considerations of such flows. To this end we consider the energy equation for incompressible,
steady flow between two locations as is given in Eq. 5.89
p 1
g a 1 V 1 2
2g z 1 p 2
g a 2 V 2 2
2g z 2 h L
(8.21)
p 1
h L
z 1
(1)
p 2
(2)
z 2
Recall that the kinetic energy coefficients, a 1 and a 2 , compensate for the fact that the velocity
profile across the pipe is not uniform. For uniform velocity profiles, a 1, whereas for any
nonuniform profile, a 7 1. The head loss term, h L , accounts for any energy loss associated with
the flow. This loss is a direct consequence of the viscous dissipation that occurs throughout the
fluid in the pipe. For the ideal 1inviscid2 cases discussed in previous chapters, a 1 a 2 1, h L 0,
and the energy equation reduces to the familiar Bernoulli equation discussed in Chapter 3
1Eq. 3.72.
Even though the velocity profile in viscous pipe flow is not uniform, for fully developed
flow it does not change from section 112 to section 122 so that a 1 a 2 . Thus, the kinetic energy
is the same at any section 1a 1 V 12 2 a 2 V 22 22 and the energy equation becomes
a p 1
g z 1b a p 2
g z 2b h L
(8.22)
The energy dissipated by the viscous forces within the fluid is supplied by the excess work done
by the pressure and gravity forces as shown by the figure in the margin.
A comparison of Eqs. 8.22 and 8.10 shows that the head loss is given by
h L 2t/
gr
1recall p 1 p 2 ¢p and z 2 z 1 / sin u2, which, by use of Eq. 8.4, can be rewritten in the form
The head loss in a
pipe is a result of
the viscous shear
stress on the wall.
h L 4/t w
gD
(8.23)
It is the shear stress at the wall 1which is directly related to the viscosity and the shear stress
throughout the fluid2 that is responsible for the head loss. A closer consideration of the assumptions
involved in the derivation of Eq. 8.23 will show that it is valid for both laminar and turbulent
flow.