fluid_mechanics

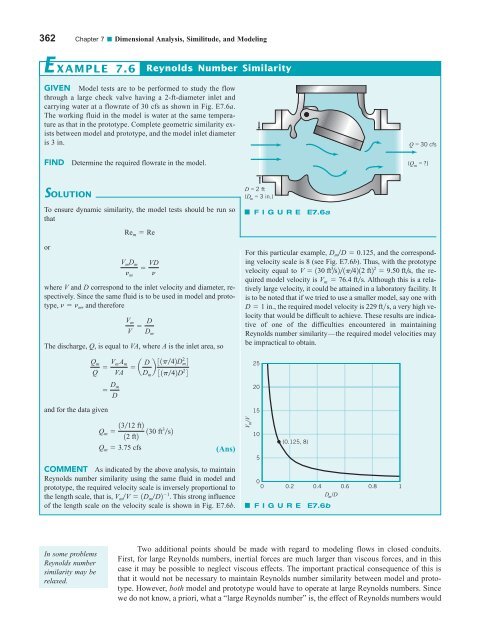
362 Chapter 7 ■ Dimensional Analysis, Similitude, and Modeling
E XAMPLE 7.6
Reynolds Number Similarity
GIVEN Model tests are to be performed to study the flow
through a large check valve having a 2-ft-diameter inlet and
carrying water at a flowrate of 30 cfs as shown in Fig. E7.6a.
The working fluid in the model is water at the same temperature
as that in the prototype. Complete geometric similarity exists
between model and prototype, and the model inlet diameter
is 3 in.
Q = 30 cfs
FIND
Determine the required flowrate in the model.
(Q m = ?)
SOLUTION
To ensure dynamic similarity, the model tests should be run so
that
or
where V and D correspond to the inlet velocity and diameter, respectively.
Since the same fluid is to be used in model and prototype,
n n m , and therefore
The discharge, Q, is equal to VA, where A is the inlet area, so
and for the data given
Q m
Q V mA m
VA
a D 31p42D 2 m 4
b
D m 31p42D 2 4
D m
D
Re m Re
V m D m
n m
V m
VD n
V D D m
D = 2 ft
(D m = 3 in.)
F I G U R E E7.6a
For this particular example, D mD 0.125, and the corresponding
velocity scale is 8 (see Fig. E7.6b). Thus, with the prototype
velocity equal to V 130 ft 3 s21p4212 ft2 2 9.50 fts, the required
model velocity is V m 76.4 fts. Although this is a relatively
large velocity, it could be attained in a laboratory facility. It
is to be noted that if we tried to use a smaller model, say one with
D 1 in., the required model velocity is 229 fts, a very high velocity
that would be difficult to achieve. These results are indicative
of one of the difficulties encountered in maintaining
Reynolds number similarity—the required model velocities may
be impractical to obtain.
25
20
15
Q m 13 12 ft2
12 ft2
Q m 3.75 cfs
130 ft 3 s2
(Ans)
COMMENT As indicated by the above analysis, to maintain
Reynolds number similarity using the same fluid in model and
prototype, the required velocity scale is inversely proportional to
the length scale, that is, V mV 1D mD2 1 . This strong influence
of the length scale on the velocity scale is shown in Fig. E7.6b.
V m /V
10
(0.125, 8)
5
0
0 0.2 0.4 0.6
D m /D
0.8 1
F I G U R E E7.6b
In some problems
Reynolds number
similarity may be
relaxed.
Two additional points should be made with regard to modeling flows in closed conduits.
First, for large Reynolds numbers, inertial forces are much larger than viscous forces, and in this
case it may be possible to neglect viscous effects. The important practical consequence of this is
that it would not be necessary to maintain Reynolds number similarity between model and prototype.
However, both model and prototype would have to operate at large Reynolds numbers. Since
we do not know, a priori, what a “large Reynolds number” is, the effect of Reynolds numbers would