fluid_mechanics

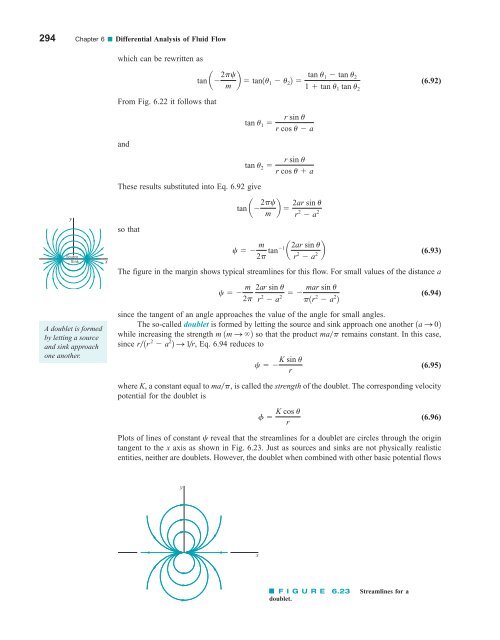
294 Chapter 6 ■ Differential Analysis of Fluid Flow
which can be rewritten as
tan a 2pc
m b tan1u 1 u 2 2 tan u 1 tan u 2
1 tan u 1 tan u 2
(6.92)
From Fig. 6.22 it follows that
y
Source
Sink
A doublet is formed
by letting a source
and sink approach
one another.
x
and
These results substituted into Eq. 6.92 give
so that
tan u 1
tan u 2
tan a 2pc
m
r sin u
r cos u a
r sin u
r cos u a
b 2ar sin u
r
2 a 2
c m 2ar sin u
2p tan1 a
r 2 a b 2
(6.93)
The figure in the margin shows typical streamlines for this flow. For small values of the distance a
c m 2ar sin u sin u
mar
2p r
2 2
a p1r 2 a 2 2
(6.94)
since the tangent of an angle approaches the value of the angle for small angles.
The so-called doublet is formed by letting the source and sink approach one another 1a S 02
while increasing the strength m 1m S 2 so that the product map remains constant. In this case,
since r1r 2 a 2 2 S 1r, Eq. 6.94 reduces to
c K sin u
r
(6.95)
where K, a constant equal to map, is called the strength of the doublet. The corresponding velocity
potential for the doublet is
f K cos u
r
(6.96)
Plots of lines of constant c reveal that the streamlines for a doublet are circles through the origin
tangent to the x axis as shown in Fig. 6.23. Just as sources and sinks are not physically realistic
entities, neither are doublets. However, the doublet when combined with other basic potential flows
y
x
F I G U R E 6.23
doublet.
Streamlines for a