Create successful ePaper yourself
Turn your PDF publications into a flip-book with our unique Google optimized e-Paper software.
Section 7.3 Volumes 399<br />
7.3<br />
Volumes<br />
What you’ll learn about<br />
• Volume As an Integral<br />
• Square Cross Sections<br />
• Circular Cross Sections<br />
• Cylindrical Shells<br />
• Other Cross Sections<br />
. . . and why<br />
The techniques of this section<br />
allow us to compute volumes of<br />
certain solids in three dimensions.<br />
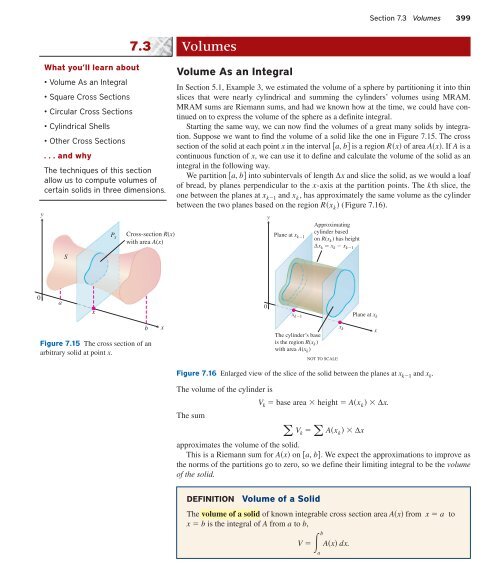
y<br />
S<br />
P x<br />
Cross-section R(x)<br />
with area A(x)<br />
Volume As an Integral<br />
In Section 5.1, Example 3, we estimated the volume of a sphere by partitioning it into thin<br />
slices that were nearly cylindrical and summing the cylinders’ volumes using MRAM.<br />
MRAM sums are Riemann sums, and had we known how at the time, we could have continued<br />
on to express the volume of the sphere as a definite integral.<br />
Starting the same way, we can now find the volumes of a great many solids by integration.<br />
Suppose we want to find the volume of a solid like the one in Figure 7.15. The cross<br />
section of the solid at each point x in the interval a, b is a region Rx of area Ax. If A is a<br />
continuous function of x, we can use it to define and calculate the volume of the solid as an<br />
integral in the following way.<br />
We partition a, b into subintervals of length Δx and slice the solid, as we would a loaf<br />
of bread, by planes perpendicular to the x-axis at the partition points. The kth slice, the<br />
one between the planes at x k1 and x k , has approximately the same volume as the cylinder<br />
between the two planes based on the region Rx k (Figure 7.16).<br />
y<br />
Plane at x k1<br />
Approximating<br />
cylinder based<br />
on R(x k ) has height<br />
Δx k x k x k1<br />
0<br />
a<br />
x<br />
0<br />
x k1<br />
Plane at x k<br />
Figure 7.15 The cross section of an<br />
arbitrary solid at point x.<br />
b<br />
x<br />
The cylinder’s base<br />
is the region R(x k )<br />
with area A(x k )<br />
NOT TO SCALE<br />
x k<br />
x<br />
Figure 7.16 Enlarged view of the slice of the solid between the planes at x k1 and x k .<br />
The volume of the cylinder is<br />
V k base area height Ax k Δx.<br />
The sum<br />
V k Ax k Δx<br />
approximates the volume of the solid.<br />
This is a Riemann sum for Ax on a, b. We expect the approximations to improve as<br />
the norms of the partitions go to zero, so we define their limiting integral to be the volume<br />
of the solid.<br />
DEFINITION<br />
Volume of a Solid<br />
The volume of a solid of known integrable cross section area Ax from x a to<br />
x b is the integral of A from a to b,<br />
V b<br />
a<br />
Ax dx.