5128_Ch07_pp378-433

Section 7.1 Integral as Net Change 389
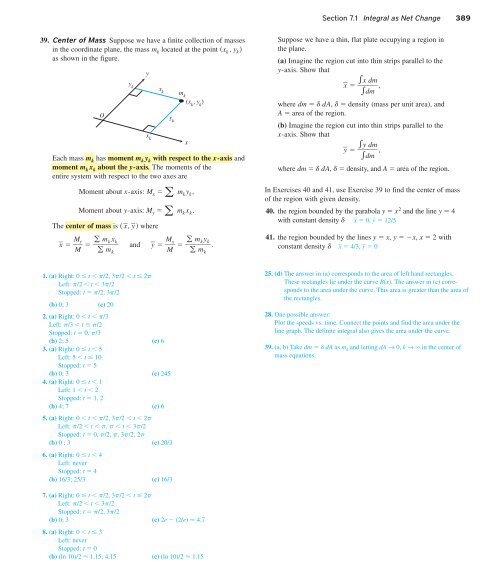
39. Center of Mass Suppose we have a finite collection of masses
in the coordinate plane, the mass m k located at the point x k , y k
as shown in the figure.
O
Each mass m k has moment m k y k with respect to the x-axis and
moment m k x k about the y-axis. The moments of the
entire system with respect to the two axes are
Moment about x-axis: M x
m k y k ,
Moment about y-axis: M y
m k x k .
The center of mass is xJ, yJ where
xJ M y m
and
M
yJ M k x x m k y
k
k
.
m k
M m k
y
y k x k m k(xk
, y k )
y k
x k
x
Suppose we have a thin, flat plate occupying a region in
the plane.
(a) Imagine the region cut into thin strips parallel to the
y-axis. Show that
xJ x dm
,
dm
where dm d dA, d density (mass per unit area), and
A area of the region.
(b) Imagine the region cut into thin strips parallel to the
x-axis. Show that
yJ y dm
,
dm
where dm d dA, d density, and A area of the region.
In Exercises 40 and 41, use Exercise 39 to find the center of mass
of the region with given density.
40. the region bounded by the parabola y x 2 and the line y 4
with constant density d x 0, y 12/5
41. the region bounded by the lines y x, y x, x 2 with
constant density d x 4/3, y 0
1. (a) Right: 0 t p/2, 3p/2 t 2p
Left: p/2 t 3p/2
Stopped: t p/2, 3p/2
(b) 0; 3 (c) 20
2. (a) Right: 0 t p/3
Left: p/3 t p/2
Stopped: t 0, p/3
(b) 2; 5 (c) 6
3. (a) Right: 0 t 5
Left: 5 t 10
Stopped: t 5
(b) 0; 3 (c) 245
4. (a) Right: 0 t 1
Left: 1 t 2
Stopped: t 1, 2
(b) 4; 7 (c) 6
5. (a) Right: 0 t p/2, 3p/2 t 2p
Left: p/2 t p, p t 3p/2
Stopped: t 0, p/2, p, 3p/2, 2p
(b) 0 ; 3 (c) 20/3
6. (a) Right: 0 t 4
Left: never
Stopped: t 4
(b) 16/3; 25/3 (c) 16/3
25. (d) The answer in (a) corresponds to the area of left hand rectangles.
These rectangles lie under the curve B(x). The answer in (c) corresponds
to the area under the curve. This area is greater than the area of
the rectangles.
28. One possible answer:
Plot the speeds vs. time. Connect the points and find the area under the
line graph. The definite integral also gives the area under the curve.
39. (a, b) Take dm d dA as m k and letting dA → 0, k →∞in the center of
mass equations.
7. (a) Right: 0 t p/2, 3p/2 t 2p
Left: p/2 t 3p/2
Stopped: t p/2, 3p/2
(b) 0; 3 (c) 2e (2/e) 4.7
8. (a) Right: 0 t 3
Left: never
Stopped: t 0
(b) (ln 10)/2 1.15; 4.15 (c) (ln 10)/2 1.15