5128_Ch03_pp098-184

180 Chapter 3 Derivatives
Exploration
17. 1 x , x 0 18. 1 x , x 0 21. x l
2
n4
63. Let y 1 a x , y 2 NDER y 1 , y 3 y 2y 1 , and y 4 e y 3.
(a) Describe the graph of y 4 for a 2, 3, 4, 5. Generalize
your description to an arbitrary a 1.
(b) Describe the graph of y 3 for a 2, 3, 4, 5. Compare a
table of values for y 3 for a 2, 3, 4, 5 with ln a.
Generalize your description to an arbitrary a 1.
(c) Explain how parts (a) and (b) support the statement
1
22.
1
, x 0
x l 2x
n2 ln 5
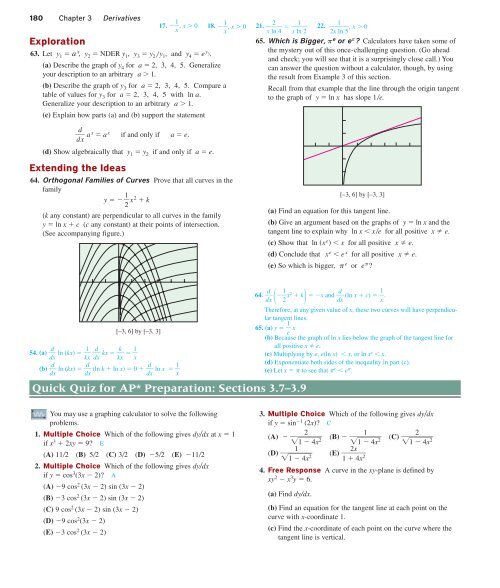
65. Which is Bigger, p e or e p ? Calculators have taken some of
the mystery out of this once-challenging question. (Go ahead
and check; you will see that it is a surprisingly close call.) You
can answer the question without a calculator, though, by using
the result from Example 3 of this section.
Recall from that example that the line through the origin tangent
to the graph of y ln x has slope 1e.
d
a d x
x a x if and only if a e.
(d) Show algebraically that y 1 y 2 if and only if a e.
Extending the Ideas
64. Orthogonal Families of Curves Prove that all curves in the
family
y 1 2 x2 k
(k any constant) are perpendicular to all curves in the family
y ln x c (c any constant) at their points of intersection.
(See accompanying figure.)
[–3, 6] by [–3, 3]
(a) Find an equation for this tangent line.
(b) Give an argument based on the graphs of y ln x and the
tangent line to explain why ln x xe for all positive x e.
(c) Show that ln x e x for all positive x e.
(d) Conclude that x e e x for all positive x e.
(e) So which is bigger, p e or e p ?
[–3, 6] by [–3, 3]
d 1
54. (a) ln (kx) d x k x d k
kx
dx
k x 1 x
d d
d
(b) ln (kx) (ln k ln x) 0 ln x 1 d x d x
d x x
Quick Quiz for AP* Preparation: Sections 3.7–3.9
d
64. d x 1 2 x2 k x and d
(ln x c) 1 dx
x .
Therefore, at any given value of x, these two curves will have perpendicular
tangent lines.
65. (a) y 1 e x
(b) Because the graph of ln x lies below the graph of the tangent line for
all positive x e.
(c) Multiplying by e, e(ln x) x, or ln x e x.
(d) Exponentiate both sides of the inequality in part (c).
(e) Let x to see that e e .
You may use a graphing calculator to solve the following
problems.
1. Multiple Choice Which of the following gives dydx at x 1
if x 3 2xy 9? E
(A) 112 (B) 52 (C) 32 (D) 52 (E) 112
2. Multiple Choice Which of the following gives dydx
if y cos 3 (3x 2)? A
(A) 9 cos 2 (3x 2) sin (3x 2)
(B) 3 cos 2 (3x 2) sin (3x 2)
(C) 9 cos 2 (3x 2) sin (3x 2)
(D) 9 cos 2 (3x 2)
(E) 3 cos 2 (3x 2)
3. Multiple Choice Which of the following gives dydx
if y sin 1 (2x)? C
2
(A) 1 4x
2 (B) 1
1 4x
2 (C) 2
1 4x
2
1
(D) 1 4x
2 (E) 2x4x 1 2
4. Free Response A curve in the xy-plane is defined by
xy 2 x 3 y 6.
(a) Find dy/dx.
(b) Find an equation for the tangent line at each point on the
curve with x-coordinate 1.
(c) Find the x-coordinate of each point on the curve where the
tangent line is vertical.