5128_Ch03_pp098-184
You also want an ePaper? Increase the reach of your titles
YUMPU automatically turns print PDFs into web optimized ePapers that Google loves.
172 Chapter 3 Derivatives<br />
3.9<br />
What you’ll learn about<br />
• Derivative of e x<br />
• Derivative of a x<br />
• Derivative of lnx<br />
• Derivative of log a x<br />
• Power Rule for Arbitrary Real<br />
Powers<br />
. . . and why<br />
The relationship between<br />
exponential and logarithmic<br />
functions provides a powerful<br />
differentiation tool called<br />
logarithmic differentiation.<br />
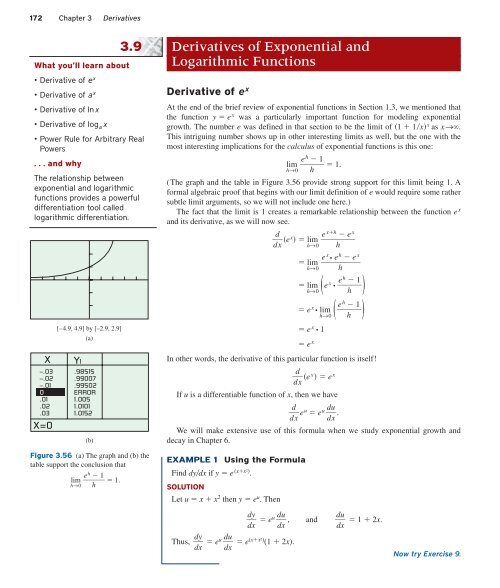
X<br />
–.03<br />
–.02<br />
–.01<br />
0<br />
.01<br />
.02<br />
.03<br />
X=0<br />
[–4.9, 4.9] by [–2.9, 2.9]<br />
(a)<br />
Y1<br />
.98515<br />
.99007<br />
.99502<br />
ERROR<br />
1.005<br />
1.0101<br />
1.0152<br />
(b)<br />
Figure 3.56 (a) The graph and (b) the<br />
table support the conclusion that<br />
1<br />
lim<br />
h→0 eh 1.<br />
h<br />
Derivatives of Exponential and<br />
Logarithmic Functions<br />
Derivative of e x<br />
At the end of the brief review of exponential functions in Section 1.3, we mentioned that<br />
the function y e x was a particularly important function for modeling exponential<br />
growth. The number e was defined in that section to be the limit of 1 1x x as x→.<br />
This intriguing number shows up in other interesting limits as well, but the one with the<br />
most interesting implications for the calculus of exponential functions is this one:<br />
1<br />
lim<br />
h→0 eh 1.<br />
h<br />
(The graph and the table in Figure 3.56 provide strong support for this limit being 1. A<br />
formal algebraic proof that begins with our limit definition of e would require some rather<br />
subtle limit arguments, so we will not include one here.)<br />
The fact that the limit is 1 creates a remarkable relationship between the function e x<br />
and its derivative, as we will now see.<br />
d<br />
e d x<br />
x lim e xh e<br />
<br />
x<br />
h→0 h<br />
h e x<br />
lim e x •<br />
<br />
h→0<br />
eh<br />
lim<br />
h→0 ( e x • eh 1<br />
h<br />
e x • lim<br />
h→0 (<br />
e x • 1<br />
e x<br />
)<br />
)<br />
1<br />
eh h<br />
In other words, the derivative of this particular function is itself!<br />
d<br />
e d x<br />
x e x<br />
If u is a differentiable function of x, then we have<br />
d<br />
e d x<br />
u e u d u<br />
.<br />
dx<br />
We will make extensive use of this formula when we study exponential growth and<br />
decay in Chapter 6.<br />
EXAMPLE 1 Using the Formula<br />
Find dydx if y e (xx2) .<br />
SOLUTION<br />
Let u x x 2 then y e u . Then<br />
dy<br />
e u du<br />
du<br />
, and 1 2x.<br />
dx dx<br />
dx<br />
dy<br />
Thus, e u du<br />
e (xx2) (1 2x).<br />
dx dx<br />
Now try Exercise 9.