Class Notes on Rotational Motion - Galileo and Einstein

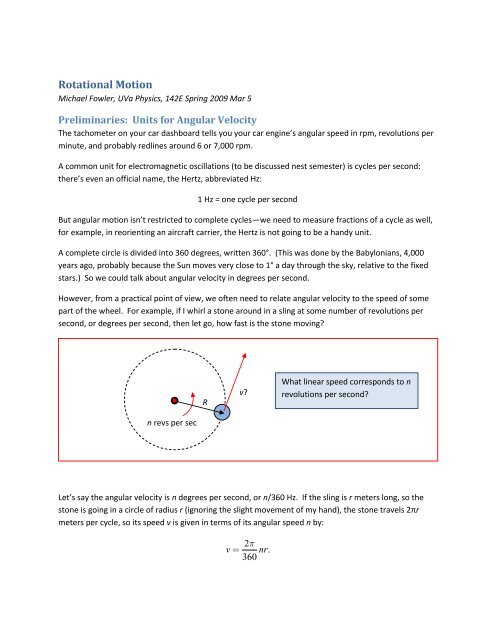
Rotational MotionMichael Fowler, UVa Physics, 142E Spring 2009 Mar 5Preliminaries: Units for Angular VelocityThe tachometer on your car dashboard tells you your car engine’s angular speed in rpm, revolutions perminute, and probably redlines around 6 or 7,000 rpm.A common unit for electromagnetic oscillations (to be discussed nest semester) is cycles per second:there’s even an official name, the Hertz, abbreviated Hz:1 Hz = one cycle per secondBut angular motion isn’t restricted to complete cycles—we need to measure fractions of a cycle as well,for example, in reorienting an aircraft carrier, the Hertz is not going to be a handy unit.A complete circle is divided into 360 degrees, written 360°. (This was done by the Babylonians, 4,000years ago, probably because the Sun moves very close to 1° a day through the sky, relative to the fixedstars.) So we could talk about angular velocity in degrees per second.However, from a practical point of view, we often need to relate angular velocity to the speed of somepart of the wheel. For example, if I whirl a stone around in a sling at some number of revolutions persecond, or degrees per second, then let go, how fast is the stone moving?Rv?What linear speed corresponds to nrevolutions per second?n revs per secLet’s say the angular velocity is n degrees per second, or n/360 Hz. If the sling is r meters long, so thestone is going in a circle of radius r (ignoring the slight movement of my hand), the stone travels 2πrmeters per cycle, so its speed v is given in terms of its angular speed n by:2v nr.360

2This rather clumsy formula is a consequence of following the Babylonians in defining the unit of angle as1/360 of a full circle. We’d have a much nicer formula if instead we defined our unit of angle as 1/2π ofa full circle!So we define: the radian = 1/2π of a full circle. This works out to be about 57.3°, and since thecomplete circumference of a circle is 2πr, the distance around the circle corresponding to an angle ofone radian is one radius. Duh.Angle of one radianthe red solid arc has lengthequal to the radius of the circle.sinθ ≈ θ for θ smallfor r = 1, the arc length is θ,the short black line = sinθ.It’s easy to see that radians are the natural angle unit for trig: look at the sine of a small angle.Our default notation for an angle is θ, measured from the x-axis, with counterclockwise as positive.Angular velocity in radians per second will usually be denoted by ω: d/ dt.So if a wheel is rotating at positive angular velocity ω radians per second about a fixed axle, a point onthe wheel at distance r from the axle is moving at speeda formula we’ll be using a lot.vr2 2The standard notation for angular acceleration is d / dt d / dt .Notice that if we have constant angular acceleration, formulas for angular velocity and angulardisplacement (meaning angle turned through) are just like those for linear motion under constant force:

3 t0 tt10 0 2 2 ( ).2 20 0Just be sure your system has constant angular acceleration before using these formulas!2Torque: Seesaw PhysicsTorque is a measure of the effectiveness of a force in getting something rotating: actually we’ve comeacross this already in the discussion of the seesaw. If a child sits at the end of a level empty seesaw, itbegins to rotate. O.K., it doesn’t get far, but there is angular acceleration. And we know the if the childsits close to the pivot point, the seesaw turns more slowly.We’ve also seen that two children, one twice as heavy sitting at half the distance, balance—we said theyhad the same leverage. This “leverage” is actually what’s called torque. It’s also called the “moment” ofa force about an axis of rotation. It’s the product of the weight of the child and the distance from thepivot or axle.So, for a 20 kg child at a distance of 1.5m, the torque about the pivot point is 300 N.m, setting g = 10.d2d2mgmgBalancing a seesawWe can also have two forces on the same side of the pivot balancing—if one of them is upwards. If a20kg child is sitting half way to the pivot, with no-one on the other side, you could lift the seesaw to ahorizontal position by supplying an upward force equivalent to 10 kg weight at the end of the seesaw,the same side as the child.

4d2dT = mg2mgBalancing a seesaw the hard wayOne further point: what if you supplied the upward force by tying a (light) rope to the end of theseesaw, and tugging at some angle? In that case, the tension in the rope, where it pulls on the seesaw,is equivalent to a force perpendicular to the seesaw plus a force parallel. The latter component doesnothing (unless the seesaw can move sideways), the upward component is all that counts.2ddT = 2mgangle 30°2mgOnly the upward rope force component countsmg√3mg2mgThere’s another way to look at this which is sometimes useful: instead of resolving the force intocomponents at its point of application, as in the diagram above, we can take the whole force, actingalong the line in space through its point of application, but calculate its leverage—the torque—by takingthe lever arm to be the distance from the pivot point to the line of the force:

5Torque = force × distance of line of force from pivot = 4mgdsin30 = 2mgd2dangle 30°T = 2mg2dsin30Either way, the formula for torque strength is:Fr sin where F is the magnitude of the force, r the distance from the pivot of the point of application, and θthe angle between the vector r from the pivot point to where the force acts and the force F .Rotational Equivalent of Newton’s First LawBy the time Galileo noticed that a smooth ball on a flat surface with little friction would keep the samevelocity a long time, it was already known that a rotating grindstone, with a well-oiled axle, would keepspinning a long time too—and this, taken to the ideal limit, is Newton’s First Law for rotation: in theabsence of torque, a body will spin at constant angular velocity indefinitely.It’s worth mentioning that a vertical grindstone must have the axle through the center of gravity. If thisis not the case, gravity will exert a torque, and although the wheel might keep spinning, it won’t be atconstant angular velocity.In fact, the torque on such an off-center wheel is given by MgrCMsin MgxCM, where r CM is thedistance of the center of mass from the axle, and θ the angle between r CMand the vertical.To prove this, imagine the wheel to be made up of a large number of glued-together small masses m i atpositions r i.

6Torque around axlefrom small part ofan off-center wheelτ i = m i gx i .axlex im im i gAs discussed in the preceding section, the torque about the axle of the weight of the small mass m i isequal to m i g multiplied by the perpendicular distance from the axle to the line of action of the force,that is, m i gx i , taking the axle as the origin of coordinates.Evidently the magnitude of the total gravitational torque from the imbalancefrom the definition of the center of mass. im gxiiMgxKinds of EquilibriumIf there is no friction at the axle, the wheel can only be at rest if the center of mass lies on the verticalline through the axle. If the center of mass is directly above the axle, the wheel might be at rest, but theslightest push will start it turning: this is called “unstable equilibrium”. If the center of mass is below theaxle, a slight push to one side will cause the wheel to swing back then oscillate, a topic we’ll return tolater—this is “stable equilibrium”. If the center of mass is exactly at the axle, the wheel will rotate at asteady rate—this is “neutral equilibrium”. These all have analogies in linear motion: assuming nofriction, unstable equilibrium is a ball perched on a mountaintop, stable is a ball sitting at the bottom ofa valley, and neutral is a ball on a flat plane. In two dimensions, there are more complicatedpossibilities: a ball in the middle of a saddle might be stable for displacements parallel to the horse, butunstable for displacement the other way. Situations analogous to this horsy example do in fact occur inphysical systems.Rotational Equivalent of Newton’s Second LawA Simple Example: Introducing the Moment of Inertia Newton’s Second Law, in the form F ma , states that an object undergoes linear acceleration inresponse to an net external force, and the mass determines the magnitude of this response. It’s prettyCM

7clear by now that the angular version must be that an object undergoes angular acceleration in responseto an net external torque—but it’s less clear what plays the role of mass this time.To find out, we’ll look at an example of angular acceleration which is almost the same as linearacceleration.Mω = dθ/dtRaxleFThe mass M has speed v = Rωand acceleration a = Rdω/dt = RαSuppose a mass M is firmly attached to a horizontal disk of negligible mass on a frictionless vertical axle,so that the mass M must go around in a horizontal circle. Assume the mass is initially at rest, then theforce F is applied. What happens in the first few moments?Well, of course, the mass accelerates with acceleration a = F/M. As soon as it reaches a significantspeed, the disk will begin to exert a force towards the center, to keep it on its circular path, but let’s justconcentrate on those first few moments before that effect becomes important.Let’s translate that initial acceleration into angular language. Since the angular velocity is related tothe actual velocity by v = R , the angular acceleration must be related to the ordinary acceleration aalong a tangential direction by a = R . So we can see how to connect the two accelerations.Now we must connect the two forces, or, rather, the linear force F with the torque acting on thesystem mass + wheel.The torque is just equal to the force F multiplied by its leverage arm R, that is, = FR. Putting thistogether with a = R , we find that F = Ma translates to: = FR = MaR = MR 2 Comparing this equation, = MR 2 , with F = Ma, we notice that the quantity MR 2 plays the role ofmass, or inertia, in rotational dynamics for this simple case. It is called the moment of inertia, andlabeled I. We show in the next section that for more complicated rigid bodies, = I is still correct, andI is a sum over the body of terms like MR 2 .

8Moments of Inertia for Extended BodiesWe’ve only found the “moment of inertia” for a single mass attached to a light disk. What aboutsomething more complicated, for example a heavy disk?The key to finding the moment of inertia for a solid body is to visualize it as a lot of small masses allglued together. Think specifically of a heavy wheel of radius R, rotating about a fixed axle with negligiblefriction. Imagine the wheel to be initially at rest, then a force F is applied to the rim tangentially, so thatthe torque is FR. Of course, the whole wheel begins to rotate, so think what happens to one of the smallmasses m i we are imagining the wheel to be made up of. If it is at a distance r i from the axle, and theinitial angular acceleration of the wheel is , this small mass, m i say, must experience an initialacceleration r i , so there must be a total force on it of f i = m i r i , and therefore a torque acting on it off i r i = m i r i 2 .Rigid wheel made up ofmany little masses m i :when external torqueFR is applied, m i hasinitial linearacceleration r i α, soexperiences torque:axler im iFτ i = f i r i = m i r i 2 But where, exactly does this torque come from? Ultimately, it must come from the force F beingapplied to a different part of the wheel, but the immediate cause for the small mass m i ’s acceleration isjust the pulls and pushes of its neighbors.In other words, if you push on one part of the wheel, since it’s a solid body its rigidity transmits theeffect of the force to all the other parts, so the wheel rotates as a whole—provided of course the forceis not strong enough to tear the wheel apart.Since the torque felt by one small part of the wheel τ i = m i r i 2 , the total torque felt by all the parts ofthe wheel is:τ = Στ i = m i r i 2 = dmr 2 with the integral being over all the tiny masses dm making up the wheel.

9Now comes a crucial point. How do we relate this torque to the external force torque FR? What aboutall the internal forces—the fact that each part of the wheel feels forces from neighboring parts? How dowe take account of this very complicated situation?The answer is: we don’t have to! By Newton’s Third Law, all those internal forces are in equal oppositepairs, actions and reactions between neighboring masses. This means that when we sum over all partsof the wheel, we count all these forces, and they all cancel each other in pairs.Therefore the total torque is just that from the external forces.It follows that the analog of Newton’s Second Law for rotation about an axle isTorque = Iwhere the moment of inertia I is given by m i r i 2 = dmr 2 . (And remember that as always theacceleration must be measures in radians/sec 2 .)Moments of Inertia: Hoops, Disks, Cylinders, Rods.Finding the moment of inertia l is now just a matter of doing elementary integrals. For the case of ahoop of mass M, where all the mass can be taken at the same distance R from the axis of rotation, nointegral at all is necessary:For a hoop, I = MR 2 .A disk can be thought of as constructed of successive hoops, like a two-dimensional onion. As apractical matter, the best approach is to use the two-dimensional density, call it , (units: kg/m 2 ) sothat the total mass of the disk is density x total area, M = R 2 . Then the “hoop” which is that part ofthe disk between r and r + Δr from the center has mass m = 2rΔr , and that hoop has moment ofinertia mr 2 = (2rΔrr 2 . A disk can be seen asmade of concentrichoops glued together.Raxlerr + Δr

10Then just do an integral to add up the moments of inertia of all the nested hoops from 0 to R to find themoment of inertia of the disk:R3 1 4 1 2disk 22I 2 r dr R MR .0The same expression works for a cylinder, which for these purposes is just a stack of disks.What about a rod about one end? Assume its (constant) linear density is λ kg/m., so a length dx hasmass λdx. Then the moment of inertia isL2 3 2I x dx L / 3 ML / 3.0Suppose such a rod is hinged at one end, horizontal, and falls. What is the initial acceleration of its end?2 MgL / 2 I ( ML / 3) 3 gL / 2.So the end accelerates at Lα = 3g/2.If it is at first sight surprising that the end of the rod accelerates downwards faster then g, consider thefollowing system: a very light rod, hinged at one end, with a large weight attached to its middle.A very light rod hinged to a wall at one end has a mass atits center: how fast will the rod’s free end acceleratedownwards on release from a horizontal position?

11If let go from a horizontal position, the weight will completely dominate the situation, and accelerateddownwards very close to g. This means the far end of the light rod is forced downwards at close to 2g.Our example above is not that extreme, but the same idea.The Parallel Axis TheoremIf we know the moment of inertia of an object about a line through the center of mass, the moment ofinertia about any parallel line is easily found.We’ll prove this for a two-dimensional object (really a thin three-dimensional object):new axisrih m ir icenter of massThe moment of inertia of the two-dimensional object about any axisperpendicular to the object is simply related to that about theparallel axis through the center of mass.With the notation in the diagram,ICM2 miri, and recall mr i i0.iiNow I m r m ( r h)newaxisiCMCM2 2i i i ii I 2h m r m hIMh2.ii iii2This is the Parallel Axis Theorem:I I Mh2newaxisCM .where h is the perpendicular distance between the new axis and the parallel axis through the center ofgravity. It’s easy to extend this proof to a three-dimensional object: taking the parallel axes to be in thez-direction, replace r iwith (x i , y i ).

12Varying Moment of InertiaNewton did not actually write his Second Law as F = ma = mdv/dt, but as F = dp/dt, where p = mv iscalled the momentum, and in fact this is the correct description of nature in cases where the mass of anobject varies, such as a rocket expelling fuel, or a falling raindrop growing from condensation.Similarly, the rotational Second Law = Icould be written more generally asddtto allow for the possibility of a varying moment of inertia, and this is much more common than a varyingmass. Think of a spinning dancer or skater throwing her arms out and bringing them in, or of a divercurling up and uncurling his rotating body. The external torques are pretty small under these conditions,the variation in spin speed is largely due to the changing moment of inertia and the constancy of thetotal angular momentum IThe observed variations in angular velocity confirm the validity of the moregeneral law.Rotational Kinetic EnergyA rotating object has kinetic energy even if its center of mass is at rest. Think of it as composed of manysmall masses m i at distances r i from the center of mass.Then if the angular velocity is m i has speed v i = r i , and kinetic energy ½ m i r i 2 2 , and summing these,If a net torque is acting, this energy will change:ddtI KE of rotation = ½ I 2 .d dt1 22I I Iso the rate of working, the power, of a torque equals the magnitude of the torque multiplied by theangular velocity, just as a force F moving something at speed v is working at a rate Fv.This equation can also be integrated:dd I dt dt ddt1 22 The total change in kinetic energy equals the integral of torque times angular distance, valid even if thetorque is varying.Rolling Down a RampFor example, we’ll take the case of a hoop of radius R rolling down a ramp without slipping. Suppose atsome instant the center of the hoop is moving with speed v. Of course, other points on the hoop are

13moving at different speeds. The point at the bottom in contact with the ramp isn’t moving at all at thatinstant. But in the frame of reference in which the center of mass is momentarily stationary, that pointis moving backwards at R. This means that the angular speed and the linear speed of the center ofmass are related by v = R.2vv = Rωvω0Velocities relative to the ramp for noslip:zero at point of contact, v atcenter of hoop, 2v at farthest point.Velocities of parts of hoop relative toits center: v = Rω perpendicular tothe radius.What is the total energy of the rolling hoop? Imagine as usual that it is made up of a large number ofsmall masses m i . If the small mass m i has velocity v i , the total energy is ½ mv i 2 . Since its both movingalong and rolling, let’s write the velocity of the small mass m i as v v uwhere u iis the velocity of the small mass m i relative to the center of mass.Then the total kinetic energy of all the small massesi 2CM CM1 2 1 1 2 1 22mivi 2mi v ui 2MvCM vCM mui i 2mui i.i i i iThe first term is just the ordinary kinetic energy of linear motion, the last term is the same as the kineticenergy of rotation for the center of mass at rest. The middle term is zero, because the sum is just the d linear momentum in the center of mass frame, which is zero. ( mui i miri0in the framedtin which the center of mass is at rest.)iii

14Therefore, the total energy is just the sum of the translational kinetic energy and the rotational kineticenergy:E Mv I1 2 1 22 CM 2where I is the moment of inertia about the center of mass.So what does this mean for the hoop? If it’s rolling at speed v, it’s rotating at angular speed v/R ,and since its moment of inertia is MR 2 , its total energy is1 2 1 2 1 2 1 2 2 2E 2Mv 2I2Mv 2MR ( v / R) Mv .So it has twice the kinetic energy of a block of the same mass sliding down at the same speed. Putanother way, a block sliding down through a height h will have speed given by v 2 = 2gh, the hoop willonly reach speed v 2 = gh, because it will have lost the same amount of potential energy, so must havethe same total kinetic energy—including the rotational kinetic energy, which the block didn’t have.The equation F = ma still describes its linear motion down the ramp, and after descending a distance xits velocity is given by v 2 = 2ax. Evidently, the rolling hoop has only half the acceleration of the slidingblock, so must only be subject to half the force pulling it downhill. The difference is the retarding staticfrictional force at the point of contact: that must be just half of the gravitational force componentpointing down the hill. If there isn’t enough friction, the hoop will slide without increasing its angularvelocity. (Note: the hoop has only half the acceleration of the block, but reaches a final velocity greaterthan half that of the block, because it accelerates for a longer time.)