Chapter 6: Product operators - The James Keeler Group

More documents

Recommendations

Info

6.5 Multiple quantum terms6.5.1 Coherence orderIn NMR the directly observable quantity is the transverse magnetization, whichin product operators is represented by terms such as I 1xand 2I 1zI 2 y. Such termsare examples of single quantum coherences, or more generally coherences withorder, p = ±1. Other product operators can also be classified according tocoherence order e.g. 2I 1zI 2 zhas p = 0 and 2I 1xI 2yhas both p = 0 (zero-quantumcoherence) and ±2 (double quantum coherence). Only single quantumcoherences are observable.In heteronuclear systems it is sometimes useful to classify operatorsaccording to their coherence orders with respect to each spin. So, for example,2I 1zI 2 yhas p = 0 for spin 1 and p = ±1 for spin 2.6.5.2 Raising and lowering operatorsThe classification of operators according to coherence order is best carried outbe re-expressing the Cartesian operators I xand I yin terms of the raising andlowering operators, I +and I –, respectively. These are defined as followsI = I + iI I = I − iI[6.6]+ x y − x ywhere i is the square root of –1. I +has coherence order +1 and I –has coherenceorder –1; coherence order is a signed quantity.Using the definitions of Eqn. [6.6] I xand I ycan be expressed in terms of theraising and lowering operators( ) = ( )11Ix = 2 I+ + I− Iy 2 i I+ – I−[6.7]from which it is seen that I xand I yare both mixtures of coherences with p = +1and –1.The operator product 2I 1xI 2xcan be expressed in terms of the raising andlowering operators in the following way (note that separate operators are usedfor each spin: I 1±and I 2±)112I1 I = 22 × ( 2 I + 1I )× ( 1 2 I + 2I )x x + − + 2−[6.8]11= 2 ( I1 +I2+ + I1 −I2− )+ 2 ( I1 +I2− + I1 −I2+)The first term on the right of Eqn. [6.8] has p = (+1+1) = 2 and the second termhas p = (–1–1) = –2; both are double quantum coherences. The third and fourthterms both have p = (+1–1) = 0 and are zero quantum coherences. The value ofp can be found simply by noting the number of raising and lowering operatorsin the product.The pure double quantum part of 2I 1 xI 2xis, from Eqn. [6.8],1double quantum part[ 2I 1 xI 2 x]= 2 ( I 1 +I 2 ++ I 1 −I2 − ) [6.9]The raising and lowering operators on the right of Eqn. [6.9] can be reexpressedin terms of the Cartesian operators:6–12
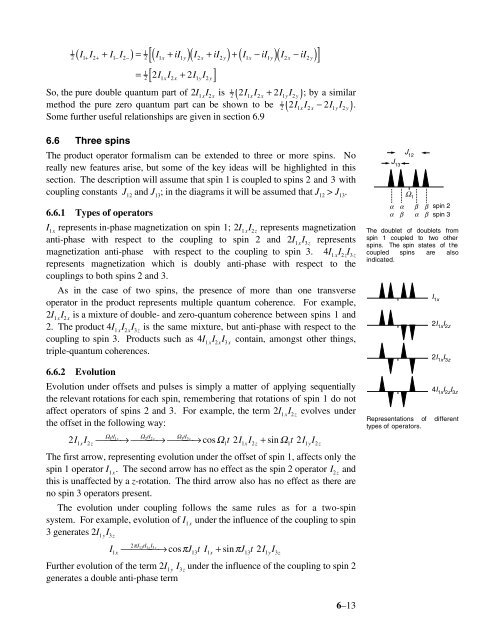
( I I + I I )= ( I + iI )( I + iI )+( I −iI ) I − iI12 1+ 2+ 1− 2−[ ( )][ ]12 1x 1y 2x 2y 1x 1y 2x 2y1= 2 2I1xI2x + 2I1yI2ySo, the pure double quantum part of 2I 1xI 2xis 1 2 ( 2I1xI2x + 2I1yI2y); by a similarmethod the pure zero quantum part can be shown to be 1 2 ( 2I1xI2x − 2I1yI2y).Some further useful relationships are given in section 6.96.6 Three spinsThe product operator formalism can be extended to three or more spins. Noreally new features arise, but some of the key ideas will be highlighted in thissection. The description will assume that spin 1 is coupled to spins 2 and 3 withcoupling constants J 12and J 13; in the diagrams it will be assumed that J 12> J 13.6.6.1 Types of operatorsI 1xrepresents in-phase magnetization on spin 1; 2I 1xI 2zrepresents magnetizationanti-phase with respect to the coupling to spin 2 and 2I 1xI 3zrepresentsmagnetization anti-phase with respect to the coupling to spin 3. 4I 1xI 2zI 3 zrepresents magnetization which is doubly anti-phase with respect to thecouplings to both spins 2 and 3.As in the case of two spins, the presence of more than one transverseoperator in the product represents multiple quantum coherence. For example,2I 1xI 2xis a mixture of double- and zero-quantum coherence between spins 1 and2. The product 4I 1 xI 2xI 3zis the same mixture, but anti-phase with respect to thecoupling to spin 3. Products such as 4I 1xI 2xI 3xcontain, amongst other things,triple-quantum coherences.6.6.2 EvolutionEvolution under offsets and pulses is simply a matter of applying sequentiallythe relevant rotations for each spin, remembering that rotations of spin 1 do notaffect operators of spins 2 and 3. For example, the term 2I 1xI 2zevolves underthe offset in the following way:Ω1tI1z Ω2tI2z Ω3tI3z2I I ⎯⎯⎯→⎯⎯⎯→⎯⎯⎯ →cosΩt 2I I + sin Ω t 2I I1x2z1 1x 2z 1 1y 2zThe first arrow, representing evolution under the offset of spin 1, affects only thespin 1 operator I 1 x. The second arrow has no effect as the spin 2 operator I 2zandthis is unaffected by a z-rotation. The third arrow also has no effect as there areno spin 3 operators present.The evolution under coupling follows the same rules as for a two-spinsystem. For example, evolution of I 1 xunder the influence of the coupling to spin3 generates 2I 1 yI 3z2πJ tI zIzI ⎯⎯⎯⎯ 13 1 3 →cosπJ t I + sinπJ t 2 I I1x13 1x 13 1y 3zFurther evolution of the term 2I 1 yI 3zunder the influence of the coupling to spin 2generates a double anti-phase termJ 13J 12Ω 1α α β β spin 2α β α β spin 3The doublet of doublets fromspin 1 coupled to two otherspins. The spin states of thecoupled spins are alsoindicated.I 1x2I 1xI 2z2I 1xI 3z4I 1xI 2zI 3zRepresentations of differenttypes of operators.6–13
Page 1 and 2: 6 Product Operators †The vector m
Page 3 and 4: and z-components of the magnetizati
Page 5 and 6: ( ) −{ } ( )≡ { − }+ { }exp
Page 7 and 8: means that the two lines of the spi
Page 9 and 10: IVxVy-yzzzyzxzzz-xz-xangle = πJtFo
Page 11: 6.1.3 Spin echoes in heteronuclear
Page 15 and 16: 6.9 Multiple -quantum coherence6.9.

Chapter 6: Product operators - The James Keeler Group

You also want an ePaper? Increase the reach of your titles

Delete template?

Save as template?