Dynamical Systems in Neuroscience:

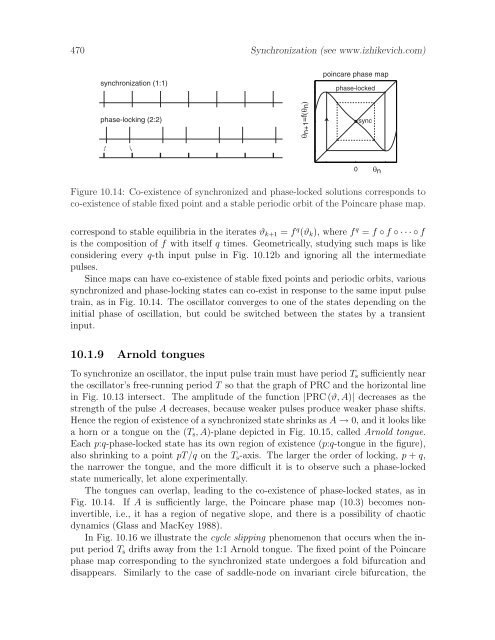
470 Synchronization (see www.izhikevich.com)synchronization (1:1)poincare phase mapphase-lockedphase-locking (2:2)n+1=f nsync0nFigure 10.14: Co-existence of synchronized and phase-locked solutions corresponds toco-existence of stable fixed point and a stable periodic orbit of the Poincare phase map.correspond to stable equilibria in the iterates ϑ k+1 = f q (ϑ k ), where f q = f ◦ f ◦ · · · ◦ fis the composition of f with itself q times. Geometrically, studying such maps is likeconsidering every q-th input pulse in Fig. 10.12b and ignoring all the intermediatepulses.Since maps can have co-existence of stable fixed points and periodic orbits, varioussynchronized and phase-locking states can co-exist in response to the same input pulsetrain, as in Fig. 10.14. The oscillator converges to one of the states depending on theinitial phase of oscillation, but could be switched between the states by a transientinput.10.1.9 Arnold tonguesTo synchronize an oscillator, the input pulse train must have period T s sufficiently nearthe oscillator’s free-running period T so that the graph of PRC and the horizontal linein Fig. 10.13 intersect. The amplitude of the function |PRC (ϑ, A)| decreases as thestrength of the pulse A decreases, because weaker pulses produce weaker phase shifts.Hence the region of existence of a synchronized state shrinks as A → 0, and it looks likea horn or a tongue on the (T s , A)-plane depicted in Fig. 10.15, called Arnold tongue.Each p:q-phase-locked state has its own region of existence (p:q-tongue in the figure),also shrinking to a point pT/q on the T s -axis. The larger the order of locking, p + q,the narrower the tongue, and the more difficult it is to observe such a phase-lockedstate numerically, let alone experimentally.The tongues can overlap, leading to the co-existence of phase-locked states, as inFig. 10.14. If A is sufficiently large, the Poincare phase map (10.3) becomes noninvertible,i.e., it has a region of negative slope, and there is a possibility of chaoticdynamics (Glass and MacKey 1988).In Fig. 10.16 we illustrate the cycle slipping phenomenon that occurs when the inputperiod T s drifts away from the 1:1 Arnold tongue. The fixed point of the Poincarephase map corresponding to the synchronized state undergoes a fold bifurcation anddisappears. Similarly to the case of saddle-node on invariant circle bifurcation, the